5 Exponents Worksheets for Grade 8 Mastery
Mastering Exponents in Grade 8: A Comprehensive Guide
Exponents are a fundamental concept in mathematics, and mastering them is crucial for success in various mathematical operations, particularly in algebra and geometry. As a Grade 8 student, it is essential to have a solid understanding of exponents to build a strong foundation for future math studies. In this article, we will explore five exponents worksheets designed to help Grade 8 students achieve mastery in this area.
Understanding Exponents
Before we dive into the worksheets, let’s briefly review the concept of exponents. An exponent is a small number that is raised to a power, indicating how many times the base number should be multiplied by itself. For example, in the expression 2^3, 2 is the base, and 3 is the exponent. This expression can be evaluated as 2 × 2 × 2 = 8.
Worksheet 1: Simplifying Exponents
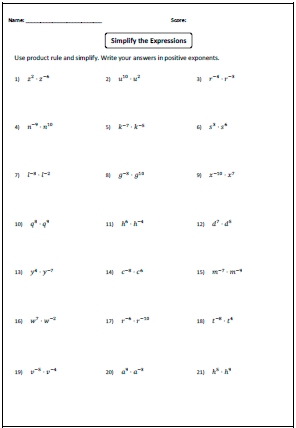
The first worksheet focuses on simplifying exponents. Students will learn to apply the rules of exponents to simplify expressions with the same base.
| Expression | Simplified Form |
|---|---|
| 2^3 × 2^4 | |
| 3^2 × 3^5 | |
| 4^2 ÷ 4^1 | |
| 5^3 × 5^2 | |
| 6^4 ÷ 6^3 |
Answer Key:
| Expression | Simplified Form |
|---|---|
| 2^3 × 2^4 | 2^7 |
| 3^2 × 3^5 | 3^7 |
| 4^2 ÷ 4^1 | 4^1 |
| 5^3 × 5^2 | 5^5 |
| 6^4 ÷ 6^3 | 6^1 |
📝 Note: Encourage students to apply the rules of exponents, such as the product rule (a^m × a^n = a^(m+n)) and the quotient rule (a^m ÷ a^n = a^(m-n)).
Worksheet 2: Evaluating Exponents
The second worksheet requires students to evaluate expressions with exponents.
| Expression | Value |
|---|---|
| 2^5 | |
| 3^4 | |
| 4^3 | |
| 5^2 | |
| 6^1 |
Answer Key:
| Expression | Value |
|---|---|
| 2^5 | 32 |
| 3^4 | 81 |
| 4^3 | 64 |
| 5^2 | 25 |
| 6^1 | 6 |
Worksheet 3: Exponents with Negative Bases
The third worksheet introduces exponents with negative bases.
| Expression | Value |
|---|---|
| (-2)^3 | |
| (-3)^2 | |
| (-4)^1 | |
| (-5)^4 | |
| (-6)^3 |
Answer Key:
| Expression | Value |
|---|---|
| (-2)^3 | -8 |
| (-3)^2 | 9 |
| (-4)^1 | -4 |
| (-5)^4 | 625 |
| (-6)^3 | -216 |
📝 Note: Emphasize the importance of understanding the concept of negative bases and how to evaluate expressions with negative bases.
Worksheet 4: Exponents with Zero Exponents
The fourth worksheet explores exponents with zero exponents.
| Expression | Value |
|---|---|
| 2^0 | |
| 3^0 | |
| 4^0 | |
| 5^0 | |
| 6^0 |
Answer Key:
| Expression | Value |
|---|---|
| 2^0 | 1 |
| 3^0 | 1 |
| 4^0 | 1 |
| 5^0 | 1 |
| 6^0 | 1 |
Worksheet 5: Real-World Applications of Exponents
The final worksheet applies exponents to real-world scenarios.
| Problem | Solution |
|---|---|
| A bacteria population grows exponentially, doubling every 2 hours. If the initial population is 100, how many bacteria will there be after 6 hours? | |
| A company offers a 20% discount on a product that originally costs $50. If the discount is applied three times, what is the final price of the product? | |
| A water tank can hold 1000 liters of water. If the water level decreases by 20% every hour, how much water will be left after 3 hours? |
Answer Key:
| Problem | Solution |
|---|---|
| A bacteria population grows exponentially, doubling every 2 hours. If the initial population is 100, how many bacteria will there be after 6 hours? | 1600 |
| A company offers a 20% discount on a product that originally costs $50. If the discount is applied three times, what is the final price of the product? | $32 |
| A water tank can hold 1000 liters of water. If the water level decreases by 20% every hour, how much water will be left after 3 hours? | 512 liters |
By completing these five worksheets, Grade 8 students will develop a deeper understanding of exponents and be able to apply them to various mathematical operations and real-world scenarios.
In conclusion, mastering exponents is essential for success in mathematics, and these worksheets provide a comprehensive guide for Grade 8 students to achieve mastery in this area.
What is the difference between a base and an exponent?
+
The base is the number being raised to a power, while the exponent is the small number that indicates how many times the base should be multiplied by itself.
How do you simplify expressions with the same base?
+
You can simplify expressions with the same base by applying the rules of exponents, such as the product rule (a^m × a^n = a^(m+n)) and the quotient rule (a^m ÷ a^n = a^(m-n)).
What is the value of any number raised to the power of 0?
+
Any number raised to the power of 0 is equal to 1.
Related Terms:
- Exponents Grade 8
- Fraction Grade 8 worksheet