Exponent Practice Worksheet for Algebra Success
Exponent Practice Worksheet for Algebra Success
Mastering exponents is a crucial skill for algebra success. Exponents are used to represent repeated multiplication of a number by itself. For example, 2³ means 2 multiplied by itself three times (2 × 2 × 2). In this worksheet, we’ll provide you with practice exercises to help you become proficient in working with exponents.
Understanding Exponent Rules
Before we dive into the practice exercises, let’s review some essential exponent rules:
- Product of Powers: When multiplying two powers with the same base, add the exponents. For example, 2³ × 2⁴ = 2³⁻⁴ = 2⁷.
- Power of a Power: When raising a power to another power, multiply the exponents. For example, (2³)⁴ = 2³⁻⁴ = 2¹².
- Zero Exponent: Any number raised to the zero power is equal to 1. For example, 2⁰ = 1.
- Negative Exponent: A negative exponent represents the reciprocal of the base raised to the positive exponent. For example, 2⁻³ = 1/2³.
Practice Exercises
Now that we’ve reviewed the exponent rules, it’s time to put them into practice. Here are some exercises to help you master exponents:
Simplifying Exponents
Simplify each expression by applying the exponent rules:
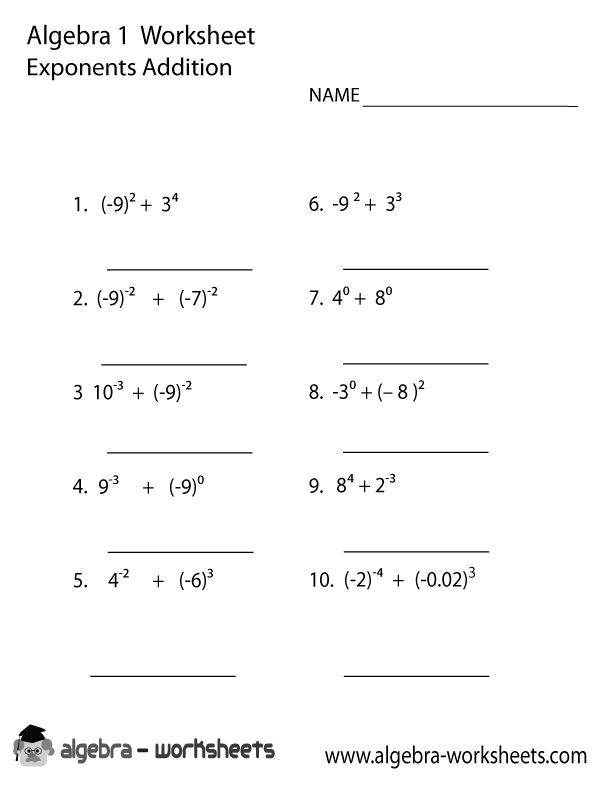
- 3⁵ × 3² =?
- (2⁴)³ =?
- 5⁰ =?
- 2⁻⁵ =?

| Expression | Simplified Answer |
|---|---|
| 3⁵ × 3² | 3⁷ |
| (2⁴)³ | 2¹² |
| 5⁰ | 1 |
| 2⁻⁵ | 1/2⁵ |
Evaluating Exponents
Evaluate each expression by substituting the given values:
- 2 × 2³, where x = 2
- x⁴, where x = 3
- (5 × 2)², where x = 4
- x⁻³, where x = 2
| Expression | Evaluate Answer |
|---|---|
| 2 × 2³ | 2 × 8 = 16 |
| x⁴ | 3⁴ = 81 |
| (5 × 2)² | (10)² = 100 |
| x⁻³ | 2⁻³ = 1/8 |
Important Notes:
📝 Note: When simplifying expressions, always follow the order of operations (PEMDAS/BODMAS).
📝 Note: When evaluating expressions, substitute the given values carefully to avoid errors.
Exponent Word Problems
Exponents are used in real-world applications, such as finance, physics, and computer science. Here are some word problems that involve exponents:
- Compound Interest: If $1000 is invested at a 5% annual interest rate, compounded annually, how much will it be worth after 5 years?
- Population Growth: A city’s population grows at a rate of 2% per year. If the current population is 500,000, how many people will live in the city after 10 years?
- Radioactive Decay: A radioactive substance decays at a rate of 10% per hour. If 100 grams of the substance are present initially, how much will remain after 5 hours?
| Word Problem | Solution |
|---|---|
| Compound Interest | $1000 × (1 + 0.05)⁵ = $1276.78 |
| Population Growth | 500,000 × (1 + 0.02)¹⁰ = 662,100 |
| Radioactive Decay | 100 × (1 - 0.10)⁵ = 59.04 grams |
In conclusion, mastering exponents is essential for algebra success. By practicing simplifying and evaluating exponents, you’ll become proficient in using exponent rules to solve problems. Remember to apply these skills to real-world applications, such as finance, physics, and computer science.
What is the difference between a power and an exponent?
+A power and an exponent are often used interchangeably, but technically, a power is the result of raising a number to an exponent. For example, 2³ is a power, and 3 is the exponent.
How do I simplify expressions with negative exponents?
+To simplify expressions with negative exponents, use the rule: a⁻n = 1/aⁿ. For example, 2⁻³ = 1/2³.
Can I use exponents to solve real-world problems?
+Yes, exponents are used in many real-world applications, such as finance, physics, and computer science. They help model population growth, compound interest, and radioactive decay, among other phenomena.