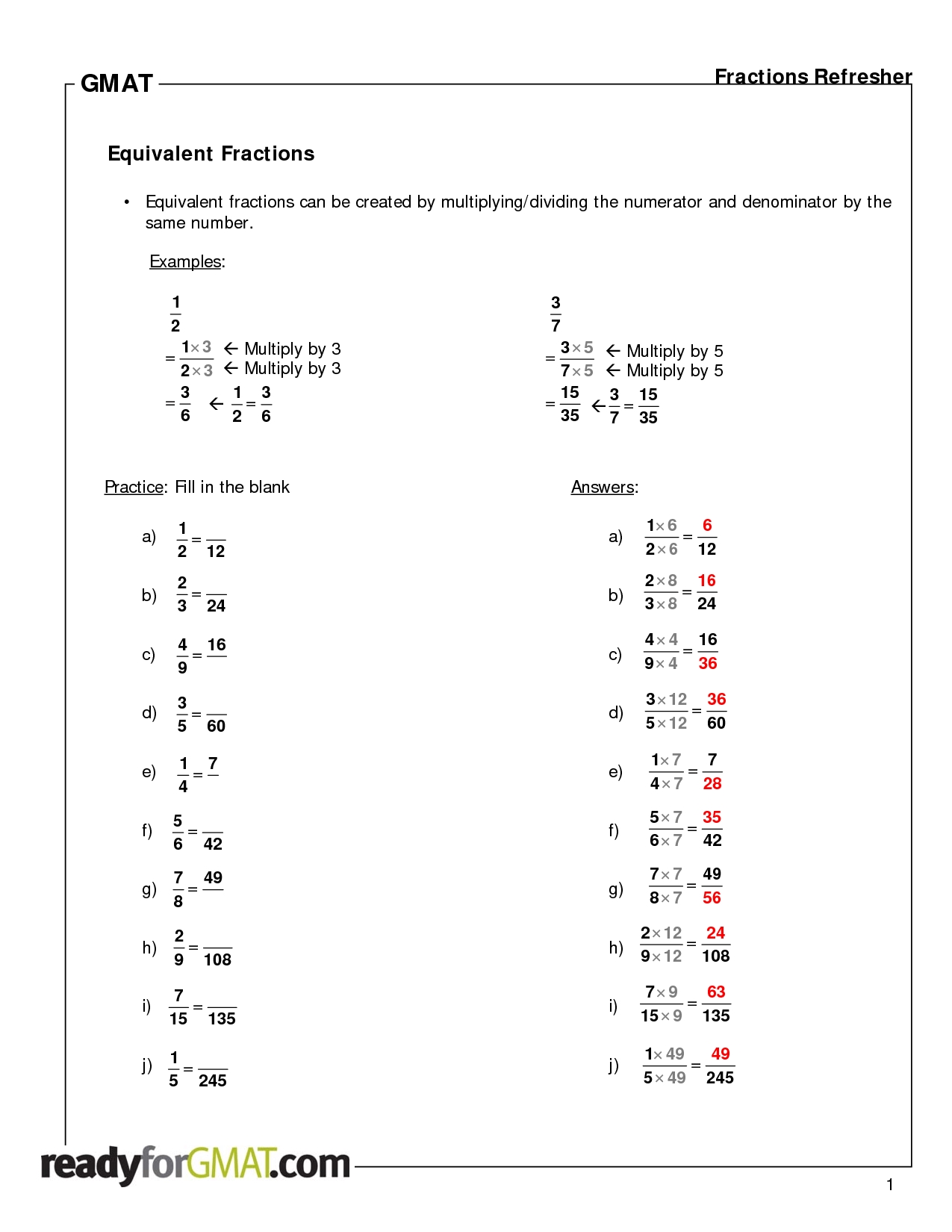
5 Ways to Simplify Equivalent Fractions

Understanding Equivalent Fractions
Equivalent fractions are fractions that have the same value, but with different numerators and denominators. For example, 1⁄2, 2⁄4, and 3⁄6 are all equivalent fractions. Simplifying equivalent fractions is an essential math skill that can help you solve problems more efficiently and accurately. In this post, we will explore 5 ways to simplify equivalent fractions.
Method 1: Divide Both Numerator and Denominator by the Greatest Common Divisor (GCD)
One of the simplest ways to simplify equivalent fractions is to divide both the numerator and denominator by the greatest common divisor (GCD). The GCD is the largest number that divides both numbers without leaving a remainder.
For example, let’s simplify the fraction 12⁄18. The GCD of 12 and 18 is 6. So, we divide both numbers by 6:
12 ÷ 6 = 2 18 ÷ 6 = 3
The simplified fraction is 2⁄3.
🤔 Note: To find the GCD, you can use a calculator or list the factors of each number and find the largest common factor.
Method 2: Cancel Out Common Factors
Another way to simplify equivalent fractions is to cancel out common factors between the numerator and denominator.
For example, let’s simplify the fraction 4⁄8. We can see that both numbers have a common factor of 4. So, we can cancel out the 4:
4⁄8 = 1⁄2
The simplified fraction is 1⁄2.
📝 Note: When canceling out common factors, make sure to divide both numbers by the same factor.
Method 3: Use the "Fast" Method
The “fast” method is a quick way to simplify equivalent fractions. To use this method, simply divide the numerator by the denominator and find the quotient.
For example, let’s simplify the fraction 6⁄12. Divide 6 by 12:
6 ÷ 12 = 0.5
The quotient is 0.5, which is equivalent to 1⁄2.
⚠️ Note: This method only works when the numerator is smaller than the denominator.
Method 4: Use Visual Aids
Visual aids such as circles, squares, or rectangles can help you simplify equivalent fractions.
For example, let’s simplify the fraction 8⁄12. Draw a circle and divide it into 12 equal parts. Shade 8 of the parts:
Circle with 8 shaded parts out of 12
You can see that the shaded parts are equivalent to 2⁄3 of the circle.
📸 Note: Visual aids can help you visualize the fraction and make it easier to simplify.
Method 5: Use a Simplifying Fractions Table
A simplifying fractions table is a chart that shows equivalent fractions with different numerators and denominators.
For example, here is a simplifying fractions table for equivalent fractions with a denominator of 12:
| Numerator | Denominator | Simplified Fraction |
|---|---|---|
| 12 | 12 | 1 |
| 8 | 12 | 2/3 |
| 6 | 12 | 1/2 |
| 4 | 12 | 1/3 |
Using this table, you can find the simplified fraction for 8⁄12, which is 2⁄3.
📊 Note: You can create your own simplifying fractions table for different denominators.
In conclusion, simplifying equivalent fractions is an important math skill that can help you solve problems more efficiently and accurately. By using one of the 5 methods outlined in this post, you can simplify equivalent fractions with ease.
What is an equivalent fraction?
+An equivalent fraction is a fraction that has the same value as another fraction, but with different numerators and denominators.
Why is it important to simplify equivalent fractions?
+Simplifying equivalent fractions can help you solve problems more efficiently and accurately. It can also make it easier to compare fractions and find equivalent ratios.
Can I use multiple methods to simplify equivalent fractions?
+Yes, you can use multiple methods to simplify equivalent fractions. For example, you can use the GCD method and then cancel out common factors to simplify the fraction further.