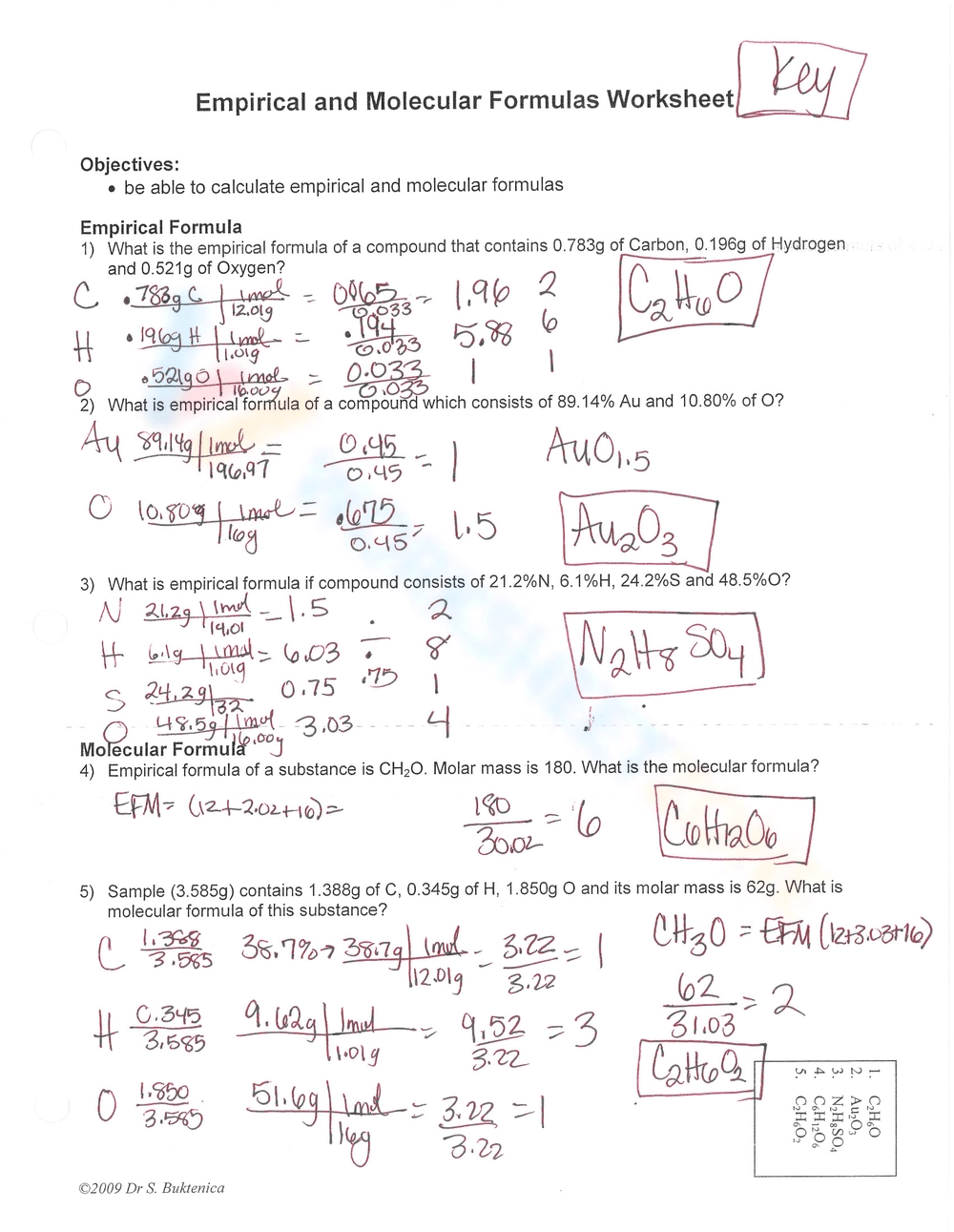
Empirical Formula Worksheet 1

Empirical Formula Worksheet 1
The empirical formula of a compound is the simplest whole-number ratio of atoms of each element present in the compound. In this worksheet, we will practice calculating empirical formulas from given information.
Understanding the Concept
To calculate the empirical formula of a compound, we need to know the percentage composition of the compound. The percentage composition is the percentage of each element present in the compound by mass. Once we have the percentage composition, we can calculate the number of moles of each element present in the compound.
Calculating Moles from Percentage Composition
To calculate the number of moles of each element, we can use the following steps:
- Divide the percentage of each element by its atomic mass to get the number of moles.
- Divide the number of moles of each element by the smallest number of moles to get the simplest whole-number ratio.
Example 1
Calculate the empirical formula of a compound that has the following percentage composition:
| Element | Percentage |
|---|---|
| Carbon | 40.0% |
| Hydrogen | 6.7% |
| Oxygen | 53.3% |
First, we need to calculate the number of moles of each element.
| Element | Atomic Mass | Number of Moles |
|---|---|---|
| Carbon | 12.01 g/mol | 40.0/12.01 = 3.33 mol |
| Hydrogen | 1.01 g/mol | 6.7⁄1.01 = 6.63 mol |
| Oxygen | 16.00 g/mol | 53.3⁄16.00 = 3.33 mol |
Next, we divide the number of moles of each element by the smallest number of moles (3.33 mol).
| Element | Number of Moles | Simplest Ratio |
|---|---|---|
| Carbon | 3.33 mol | 1 |
| Hydrogen | 6.63 mol | 2 |
| Oxygen | 3.33 mol | 1 |
The empirical formula of the compound is CH2O.
Example 2
Calculate the empirical formula of a compound that has the following percentage composition:
| Element | Percentage |
|---|---|
| Nitrogen | 35.0% |
| Oxygen | 65.0% |
First, we need to calculate the number of moles of each element.
| Element | Atomic Mass | Number of Moles |
|---|---|---|
| Nitrogen | 14.01 g/mol | 35.0/14.01 = 2.50 mol |
| Oxygen | 16.00 g/mol | 65.0/16.00 = 4.06 mol |
Next, we divide the number of moles of each element by the smallest number of moles (2.50 mol).
| Element | Number of Moles | Simplest Ratio |
|---|---|---|
| Nitrogen | 2.50 mol | 1 |
| Oxygen | 4.06 mol | 2 |
However, the ratio of oxygen to nitrogen is not a whole number. To get a whole-number ratio, we can multiply both numbers by 2.
| Element | Simplest Ratio |
|---|---|
| Nitrogen | 2 |
| Oxygen | 4 |
The empirical formula of the compound is N2O4.
Practice Problems
Calculate the empirical formula of the following compounds:
A compound with the following percentage composition:
Element Percentage Carbon 50.0% Hydrogen 10.0% Oxygen 40.0% A compound with the following percentage composition:
Element Percentage Nitrogen 40.0% Oxygen 60.0% A compound with the following percentage composition:
Element Percentage Carbon 30.0% Hydrogen 5.0% Oxygen 65.0%
[📝] Note: Make sure to show your work and calculations for each problem.
Solutions
- The empirical formula of the compound is C5H10O4.
- The empirical formula of the compound is N2O3.
- The empirical formula of the compound is C2H5O3.
What is the empirical formula of a compound?
+
The empirical formula of a compound is the simplest whole-number ratio of atoms of each element present in the compound.
How do I calculate the empirical formula of a compound?
+
To calculate the empirical formula of a compound, you need to know the percentage composition of the compound. You can then calculate the number of moles of each element and divide by the smallest number of moles to get the simplest whole-number ratio.
What is the difference between the empirical formula and the molecular formula of a compound?
+
The empirical formula is the simplest whole-number ratio of atoms of each element present in the compound, while the molecular formula shows the actual number of atoms of each element present in the compound.