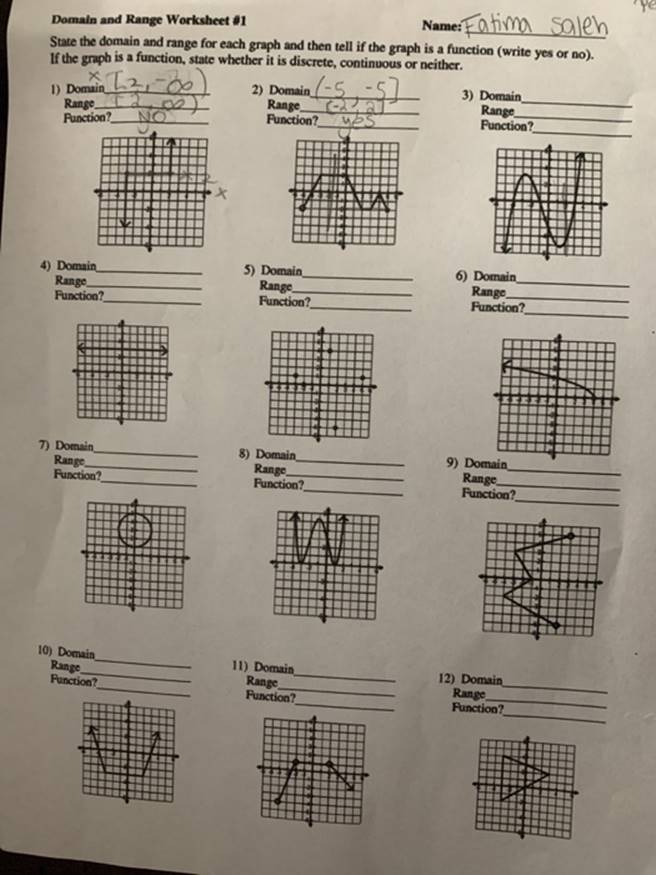
Domain and Range Worksheet for Algebra 1 Success
Understanding Domain and Range: A Key to Algebra 1 Success
Algebra 1 is a fundamental course that lays the groundwork for advanced math studies. Two crucial concepts in algebra are domain and range, which are essential in understanding functions and their behavior. In this post, we will delve into the world of domain and range, providing you with a comprehensive guide, including worksheets and exercises, to help you master these concepts.
What is Domain?
The domain of a function is the set of all possible input values (x-values) for which the function is defined. In other words, it’s the set of values that you can plug into the function without getting an undefined result. For example, consider the function f(x) = 1/x. The domain of this function is all real numbers except x = 0, because dividing by zero is undefined.
What is Range?
The range of a function is the set of all possible output values (y-values) that the function can produce. It’s the set of values that the function can take on. Using the same example as above, the range of f(x) = 1/x is all real numbers except y = 0, because the function can never produce a value of 0.
How to Find Domain and Range
Finding the domain and range of a function can be done using various methods, including:
- Graphical method: By graphing the function, you can visually identify the domain and range.
- Algebraic method: By analyzing the function’s equation, you can determine the domain and range.
- Table method: By creating a table of values, you can identify the domain and range.
Here are some examples to illustrate these methods:
Example 1: Finding Domain and Range using Graphical Method
Consider the function f(x) = x^2. By graphing the function, you can see that the domain is all real numbers, and the range is all non-negative real numbers (y ≥ 0).
Example 2: Finding Domain and Range using Algebraic Method
Consider the function f(x) = 1/(x - 2). By analyzing the equation, you can see that the domain is all real numbers except x = 2, and the range is all real numbers except y = 0.
Example 3: Finding Domain and Range using Table Method
Consider the function f(x) = x^3. By creating a table of values, you can see that the domain is all real numbers, and the range is all real numbers.
| x | f(x) |
|---|---|
| -3 | -27 |
| -2 | -8 |
| -1 | -1 |
| 0 | 0 |
| 1 | 1 |
| 2 | 8 |
| 3 | 27 |
Domain and Range Worksheet
Here’s a worksheet to help you practice finding domain and range:
Exercise 1
Find the domain and range of the function f(x) = x^2 + 3.
Exercise 2
Find the domain and range of the function f(x) = 1/(x + 1).
Exercise 3
Find the domain and range of the function f(x) = x^3 - 2.
Answer Key
Exercise 1
- Domain: All real numbers
- Range: All non-negative real numbers (y ≥ 0)
Exercise 2
- Domain: All real numbers except x = -1
- Range: All real numbers except y = 0
Exercise 3
- Domain: All real numbers
- Range: All real numbers
📝 Note: Remember to check your answers by graphing or using a table of values to ensure accuracy.
In conclusion, mastering domain and range is crucial for success in Algebra 1. By understanding these concepts, you’ll be able to analyze and graph functions, solve equations, and model real-world problems. With practice and patience, you’ll become proficient in finding domain and range, setting you up for success in your math studies.
What is the difference between domain and range?
+The domain is the set of all possible input values (x-values) for which the function is defined, while the range is the set of all possible output values (y-values) that the function can produce.
How do I find the domain and range of a function?
+You can find the domain and range using graphical, algebraic, or table methods. Graphical methods involve graphing the function, while algebraic methods involve analyzing the function’s equation. Table methods involve creating a table of values to identify the domain and range.
Why is it important to find the domain and range of a function?
+Finding the domain and range is crucial for analyzing and graphing functions, solving equations, and modeling real-world problems. It helps you understand the behavior of the function and identify any restrictions or limitations.
Related Terms:
- Domain and Range Worksheet 1
- Domain and range Worksheet PDF
- Domain and Range Worksheet 2