10 Essential Coordinate Geometry Questions and Answers
Coordinate Geometry: Understanding the Basics
Coordinate geometry is a branch of mathematics that deals with the study of geometric shapes using coordinate systems. It is a fundamental concept in mathematics and is used extensively in various fields such as physics, engineering, and computer science. In this article, we will explore the basics of coordinate geometry and provide 10 essential questions and answers to help you understand the concept better.
What is Coordinate Geometry?
Coordinate geometry is a branch of mathematics that uses a coordinate system to study geometric shapes. The coordinate system is a grid that consists of two axes, the x-axis and the y-axis, which intersect at a point called the origin. The coordinates of a point are represented as (x, y), where x is the distance from the origin to the point along the x-axis, and y is the distance from the origin to the point along the y-axis.
Key Concepts in Coordinate Geometry
There are several key concepts in coordinate geometry that you need to understand:
- Points: A point is a location on the coordinate plane, represented by its coordinates (x, y).
- Lines: A line is a set of points that extend infinitely in two directions, represented by a linear equation.
- Circles: A circle is a set of points that are equidistant from a fixed point called the center.
- Angles: An angle is formed by two lines or rays that intersect at a point.
10 Essential Coordinate Geometry Questions and Answers
Here are 10 essential questions and answers to help you understand coordinate geometry better:
Q1: What is the equation of a line that passes through the points (2, 3) and (4, 5)?
A1: The equation of a line that passes through two points (x1, y1) and (x2, y2) is given by the formula: y - y1 = (y2 - y1)/(x2 - x1) * (x - x1). Substituting the given points, we get: y - 3 = (5 - 3)/(4 - 2) * (x - 2), which simplifies to y - 3 = 1 * (x - 2).
Q2: What is the distance between the points (1, 2) and (3, 4)?
A2: The distance between two points (x1, y1) and (x2, y2) is given by the formula: √((x2 - x1)^2 + (y2 - y1)^2). Substituting the given points, we get: √((3 - 1)^2 + (4 - 2)^2) = √(4 + 4) = √8.
Q3: What is the equation of a circle with center (0, 0) and radius 3?
A3: The equation of a circle with center (a, b) and radius r is given by the formula: (x - a)^2 + (y - b)^2 = r^2. Substituting the given values, we get: (x - 0)^2 + (y - 0)^2 = 3^2, which simplifies to x^2 + y^2 = 9.
Q4: What is the slope of a line that passes through the points (2, 3) and (4, 5)?
A4: The slope of a line that passes through two points (x1, y1) and (x2, y2) is given by the formula: (y2 - y1)/(x2 - x1). Substituting the given points, we get: (5 - 3)/(4 - 2) = 2⁄2 = 1.
Q5: What is the midpoint of the line segment joining the points (1, 2) and (3, 4)?
A5: The midpoint of a line segment joining two points (x1, y1) and (x2, y2) is given by the formula: ((x1 + x2)/2, (y1 + y2)/2). Substituting the given points, we get: ((1 + 3)/2, (2 + 4)/2) = (2, 3).
Q6: What is the equation of a line that is perpendicular to the line 2x + 3y = 5?
A6: The equation of a line that is perpendicular to a given line ax + by = c is given by the formula: bx - ay = d, where d is a constant. Substituting the given line, we get: 3x - 2y = d.
Q7: What is the area of a triangle with vertices (0, 0), (3, 0), and (0, 4)?
A7: The area of a triangle with vertices (x1, y1), (x2, y2), and (x3, y3) is given by the formula: (1⁄2) * |x1(y2 - y3) + x2(y3 - y1) + x3(y1 - y2)|. Substituting the given vertices, we get: (1⁄2) * |0(0 - 4) + 3(4 - 0) + 0(0 - 0)| = (1⁄2) * |12| = 6.
Q8: What is the length of the tangent from a point (5, 6) to a circle with center (0, 0) and radius 3?
A8: The length of the tangent from a point (x1, y1) to a circle with center (a, b) and radius r is given by the formula: √((x1 - a)^2 + (y1 - b)^2 - r^2). Substituting the given values, we get: √((5 - 0)^2 + (6 - 0)^2 - 3^2) = √(25 + 36 - 9) = √52.
Q9: What is the equation of a line that passes through the point (1, 2) and is parallel to the line x + 2y = 3?
A9: The equation of a line that is parallel to a given line ax + by = c is given by the formula: ax + by = d, where d is a constant. Substituting the given line, we get: x + 2y = d. Since the line passes through the point (1, 2), we can substitute the values to get: 1 + 2(2) = d, which simplifies to d = 5. Therefore, the equation of the line is x + 2y = 5.
Q10: What is the angle between the lines 2x + 3y = 5 and x - 2y = 3?
A10: The angle between two lines a1x + b1y = c1 and a2x + b2y = c2 is given by the formula: tan(θ) = (a1b2 - a2b1)/(a1a2 + b1b2). Substituting the given lines, we get: tan(θ) = (2(-2) - 1(3))/ (2(1) + 3(-2)) = (-4 - 3)/(-4) = 7⁄4.
🔍 Note: These questions and answers cover various aspects of coordinate geometry, including equations of lines and circles, distance and midpoint formulas, and slope and angle calculations.
To summarize, coordinate geometry is a fundamental concept in mathematics that deals with the study of geometric shapes using coordinate systems. The 10 questions and answers provided above cover various aspects of coordinate geometry, including equations of lines and circles, distance and midpoint formulas, and slope and angle calculations.
What is the difference between a line and a circle in coordinate geometry?
+
A line is a set of points that extend infinitely in two directions, represented by a linear equation. A circle is a set of points that are equidistant from a fixed point called the center.
How do you find the equation of a line that passes through two points?
+
The equation of a line that passes through two points (x1, y1) and (x2, y2) is given by the formula: y - y1 = (y2 - y1)/(x2 - x1) * (x - x1).
What is the formula for the area of a triangle with vertices (x1, y1), (x2, y2), and (x3, y3)?
+
The area of a triangle with vertices (x1, y1), (x2, y2), and (x3, y3) is given by the formula: (1⁄2) * |x1(y2 - y3) + x2(y3 - y1) + x3(y1 - y2)|.
Related Terms:
- Geometri
- Trigonometri
- Geometri Euklides
- Aljabar
- Geometri analitis
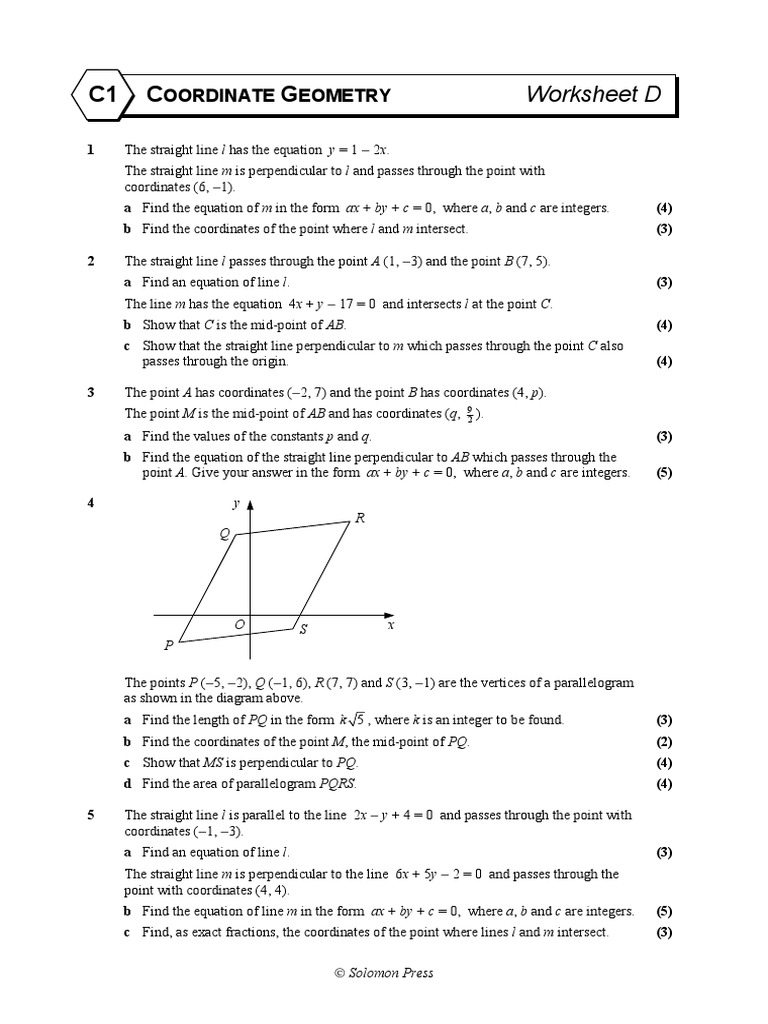
- Year 8 coordinate geometry Worksheet