Converting Mixed Numbers to Improper Fractions Made Easy

Understanding Mixed Numbers and Improper Fractions

When dealing with fractions, you may come across two types: mixed numbers and improper fractions. A mixed number is a combination of a whole number and a fraction, such as 3 ¹⁄₂. On the other hand, an improper fraction is a fraction where the numerator is greater than the denominator, like ⁵⁄₂.

Converting mixed numbers to improper fractions is a fundamental concept in mathematics, and it’s essential to understand the process. In this blog post, we will delve into the world of fractions and explore how to convert mixed numbers to improper fractions.

Why Convert Mixed Numbers to Improper Fractions?

Before we dive into the conversion process, let’s discuss why it’s essential to convert mixed numbers to improper fractions. Here are a few reasons:

• Simplification: Improper fractions can be simplified, making it easier to perform mathematical operations.
• Comparison: Converting mixed numbers to improper fractions allows for easy comparison between fractions.
• Addition and Subtraction: When adding or subtracting fractions, it’s often necessary to convert mixed numbers to improper fractions.

Step-by-Step Guide to Converting Mixed Numbers to Improper Fractions

Converting mixed numbers to improper fractions is a straightforward process. Follow these steps:

1. Multiply the Whole Number by the Denominator: Multiply the whole number part of the mixed number by the denominator.
2. Add the Numerator: Add the numerator of the mixed number to the product obtained in step 1.
3. Write the Result as an Improper Fraction: Write the result as an improper fraction, with the sum obtained in step 2 as the numerator and the original denominator as the denominator.

Let’s illustrate this process with an example:

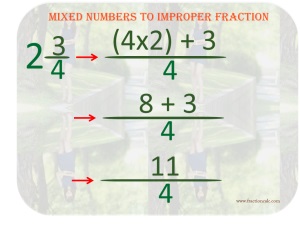
Example 1: Convert 2 ³⁄₄ to an improper fraction.

1. Multiply the whole number by the denominator: 2 × 4 = 8
2. Add the numerator: 8 + 3 = 11
3. Write the result as an improper fraction: ¹¹⁄₄

📝 Note: Make sure to multiply the whole number by the denominator, not the numerator.

More Examples and Practice

To reinforce your understanding, let’s practice with a few more examples:

Example 2: Convert 5 ¹⁄₂ to an improper fraction.

1. Multiply the whole number by the denominator: 5 × 2 = 10
2. Add the numerator: 10 + 1 = 11
3. Write the result as an improper fraction: ¹¹⁄₂

Example 3: Convert 3 ³⁄₄ to an improper fraction.

1. Multiply the whole number by the denominator: 3 × 4 = 12
2. Add the numerator: 12 + 3 = 15
3. Write the result as an improper fraction: ¹⁵⁄₄

Example 4: Convert 2 ¹⁄₃ to an improper fraction.

1. Multiply the whole number by the denominator: 2 × 3 = 6
2. Add the numerator: 6 + 1 = 7
3. Write the result as an improper fraction: ⁷⁄₃

Common Mistakes to Avoid

When converting mixed numbers to improper fractions, it’s essential to avoid common mistakes. Here are a few:

• Multiplying the Wrong Numbers: Make sure to multiply the whole number by the denominator, not the numerator.
• Forgetting to Add the Numerator: Don’t forget to add the numerator to the product obtained in step 1.
• Writing the Result Incorrectly: Ensure that you write the result as an improper fraction, with the correct numerator and denominator.

Conclusion

Converting mixed numbers to improper fractions is a straightforward process that requires attention to detail. By following the step-by-step guide and practicing with examples, you’ll become proficient in converting mixed numbers to improper fractions. Remember to avoid common mistakes and take your time to ensure accuracy.