Convert Improper Fractions to Mixed Numbers Easily

Understanding Improper Fractions and Mixed Numbers

Improper fractions and mixed numbers are two ways to represent the same value, but they are used in different contexts and have different formats. An improper fraction is a fraction where the numerator is greater than the denominator, whereas a mixed number is a combination of a whole number and a proper fraction. In this blog post, we will explore how to convert improper fractions to mixed numbers easily.

Why Convert Improper Fractions to Mixed Numbers?

There are several reasons why you might want to convert an improper fraction to a mixed number. One reason is that mixed numbers are often easier to understand and work with, especially when dealing with real-world applications. For example, if you are measuring ingredients for a recipe, it’s more intuitive to use a mixed number like 2 ¹⁄₄ cups rather than an improper fraction like ⁹⁄₄ cups.

Step-by-Step Guide to Converting Improper Fractions to Mixed Numbers

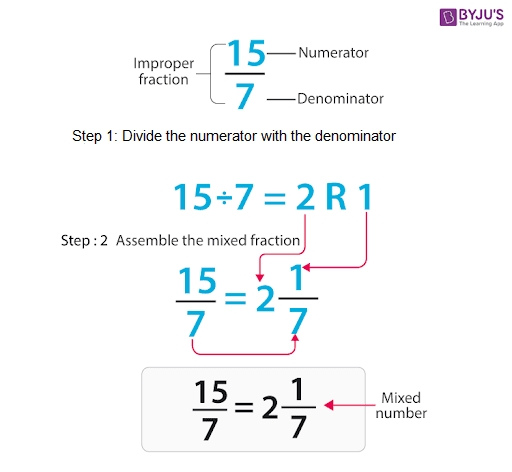
Converting an improper fraction to a mixed number is a straightforward process that involves a few simple steps. Here’s how to do it:

• Step 1: Divide the Numerator by the Denominator
  • Take the numerator (the top number) and divide it by the denominator (the bottom number).
  • This will give you the whole number part of the mixed number.
• Step 2: Find the Remainder
  • Find the remainder of the division from Step 1.
  • This will give you the numerator of the proper fraction part of the mixed number.
• Step 3: Write the Mixed Number
  • Write the whole number part from Step 1 followed by the proper fraction part from Step 2.

Let’s use an example to illustrate this process:

Suppose we want to convert the improper fraction ⁷⁄₄ to a mixed number. Here’s how we would do it:

• Step 1: Divide the Numerator by the Denominator
  • Divide 7 (the numerator) by 4 (the denominator) to get 1 with a remainder of 3.
• Step 2: Find the Remainder
  • The remainder is 3, which becomes the numerator of the proper fraction part.
• Step 3: Write the Mixed Number
  • Write the whole number part (1) followed by the proper fraction part (³⁄₄) to get the mixed number 1 ³⁄₄.

More Examples

Here are a few more examples to help you practice converting improper fractions to mixed numbers:

• Example 1: Convert the improper fraction ¹¹⁄₆ to a mixed number.
  • Divide 11 by 6 to get 1 with a remainder of 5.
  • Write the mixed number: 1 ⁵⁄₆
• Example 2: Convert the improper fraction ²⁵⁄₈ to a mixed number.
  • Divide 25 by 8 to get 3 with a remainder of 1.
  • Write the mixed number: 3 ¹⁄₈

Common Pitfalls to Avoid

When converting improper fractions to mixed numbers, there are a few common pitfalls to watch out for:

• Forgetting to Divide the Numerator by the Denominator
  • Make sure to divide the numerator by the denominator to get the whole number part.
• Getting the Remainder Wrong
  • Double-check your division to make sure you get the remainder correct.
• Writing the Mixed Number Incorrectly
  • Make sure to write the whole number part followed by the proper fraction part.

Conclusion

Converting improper fractions to mixed numbers is a simple process that requires a few basic steps. By following these steps and practicing with examples, you can become proficient in converting improper fractions to mixed numbers easily. Remember to watch out for common pitfalls and take your time to ensure accuracy.