5 Ways to Master Composite Figures Surface Area
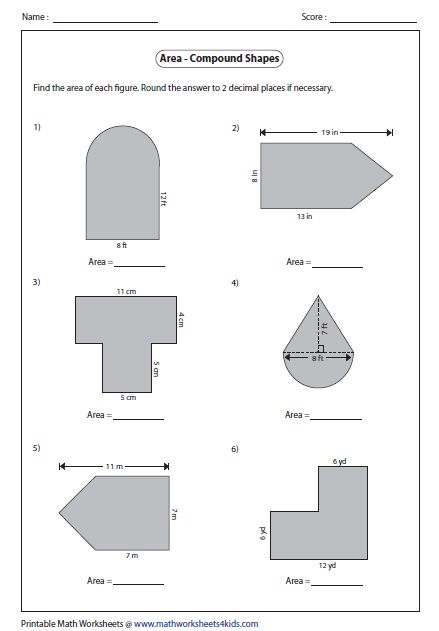
Unlocking the Secrets of Composite Figures Surface Area
Composite figures are a fascinating aspect of geometry, consisting of two or more simple shapes that are combined to form a more complex figure. One of the most essential concepts in understanding composite figures is their surface area. In this article, we will delve into the world of composite figures and explore five effective ways to master their surface area.
Understanding Composite Figures
Before we dive into the world of surface area, it’s essential to understand what composite figures are and how they are constructed. A composite figure is a shape that consists of two or more simple shapes, such as triangles, quadrilaterals, or polygons, that are combined to form a more complex figure. These figures can be two-dimensional (2D) or three-dimensional (3D), and their surface area can be calculated using various formulas and techniques.
Way #1: Break Down the Figure into Simple Shapes
One of the most effective ways to calculate the surface area of a composite figure is to break it down into its individual simple shapes. This involves identifying the different components of the figure, such as triangles, quadrilaterals, or polygons, and calculating their individual surface areas. Once you have calculated the surface area of each simple shape, you can add them up to find the total surface area of the composite figure.
For example, let’s consider a composite figure consisting of a triangle and a quadrilateral. To calculate the surface area of this figure, we can break it down into its individual components:
- Triangle: The surface area of a triangle can be calculated using the formula: Surface Area = (base × height) / 2
- Quadrilateral: The surface area of a quadrilateral can be calculated using the formula: Surface Area = side1 × side2
By calculating the surface area of each individual shape and adding them up, we can find the total surface area of the composite figure.
📝 Note: When breaking down a composite figure into simple shapes, make sure to identify any overlapping or shared sides, as these can affect the total surface area.
Way #2: Use Formulas and Equations
Another effective way to calculate the surface area of a composite figure is to use formulas and equations. These formulas can be used to calculate the surface area of individual simple shapes, as well as the total surface area of the composite figure.
For example, the surface area of a rectangular prism can be calculated using the formula: Surface Area = 2 × (length × width + length × height + width × height)
By using formulas and equations, you can quickly and accurately calculate the surface area of composite figures.
Way #3: Visualize the Figure
Visualizing the composite figure is another effective way to calculate its surface area. By drawing a diagram or sketch of the figure, you can better understand its components and how they relate to each other. This can help you identify any overlapping or shared sides, as well as calculate the surface area of individual simple shapes.
For example, let’s consider a composite figure consisting of a cube and a pyramid. By drawing a diagram of the figure, we can visualize the different components and calculate their individual surface areas.

| Shape | Surface Area Formula |
|---|---|
| Cube | Surface Area = 6 × side^2 |
| Pyramid | Surface Area = (base × height) / 2 + 4 × (side × slant height) |
By visualizing the figure and using formulas and equations, we can calculate the total surface area of the composite figure.
Way #4: Use Real-World Examples
Using real-world examples is another effective way to master the surface area of composite figures. By relating the figures to real-world objects, you can better understand their components and how they relate to each other.
For example, let’s consider a building consisting of a rectangular prism and a triangular roof. By calculating the surface area of each individual component, we can find the total surface area of the building.
- Rectangular Prism: Surface Area = 2 × (length × width + length × height + width × height)
- Triangular Roof: Surface Area = (base × height) / 2
By using real-world examples, you can develop a deeper understanding of composite figures and their surface area.
Way #5: Practice, Practice, Practice
Finally, the most effective way to master the surface area of composite figures is to practice, practice, practice! By working through numerous examples and exercises, you can develop a deeper understanding of the concepts and formulas involved.
For example, let’s consider a composite figure consisting of a sphere and a cylinder. By practicing different calculations and formulas, you can develop a better understanding of how to calculate the surface area of this figure.
| Shape | Surface Area Formula |
|---|---|
| Sphere | Surface Area = 4 × π × radius^2 |
| Cylinder | Surface Area = 2 × π × radius × height + 2 × π × radius^2 |
By practicing different calculations and formulas, you can master the surface area of composite figures and become proficient in your calculations.
In conclusion, mastering the surface area of composite figures requires a combination of understanding the concepts, using formulas and equations, visualizing the figures, using real-world examples, and practicing different calculations. By following these five effective ways, you can develop a deeper understanding of composite figures and their surface area.
What is a composite figure?
+A composite figure is a shape that consists of two or more simple shapes, such as triangles, quadrilaterals, or polygons, that are combined to form a more complex figure.
How do I calculate the surface area of a composite figure?
+The surface area of a composite figure can be calculated by breaking it down into its individual simple shapes, using formulas and equations, visualizing the figure, using real-world examples, and practicing different calculations.
What are some common formulas for calculating surface area?
+Some common formulas for calculating surface area include the formulas for triangles, quadrilaterals, polygons, and 3D shapes such as rectangular prisms and spheres.
Related Terms:
- Volume of composite figures worksheet
- Surface area composite Solids worksheet
- Surface area worksheet grade 9