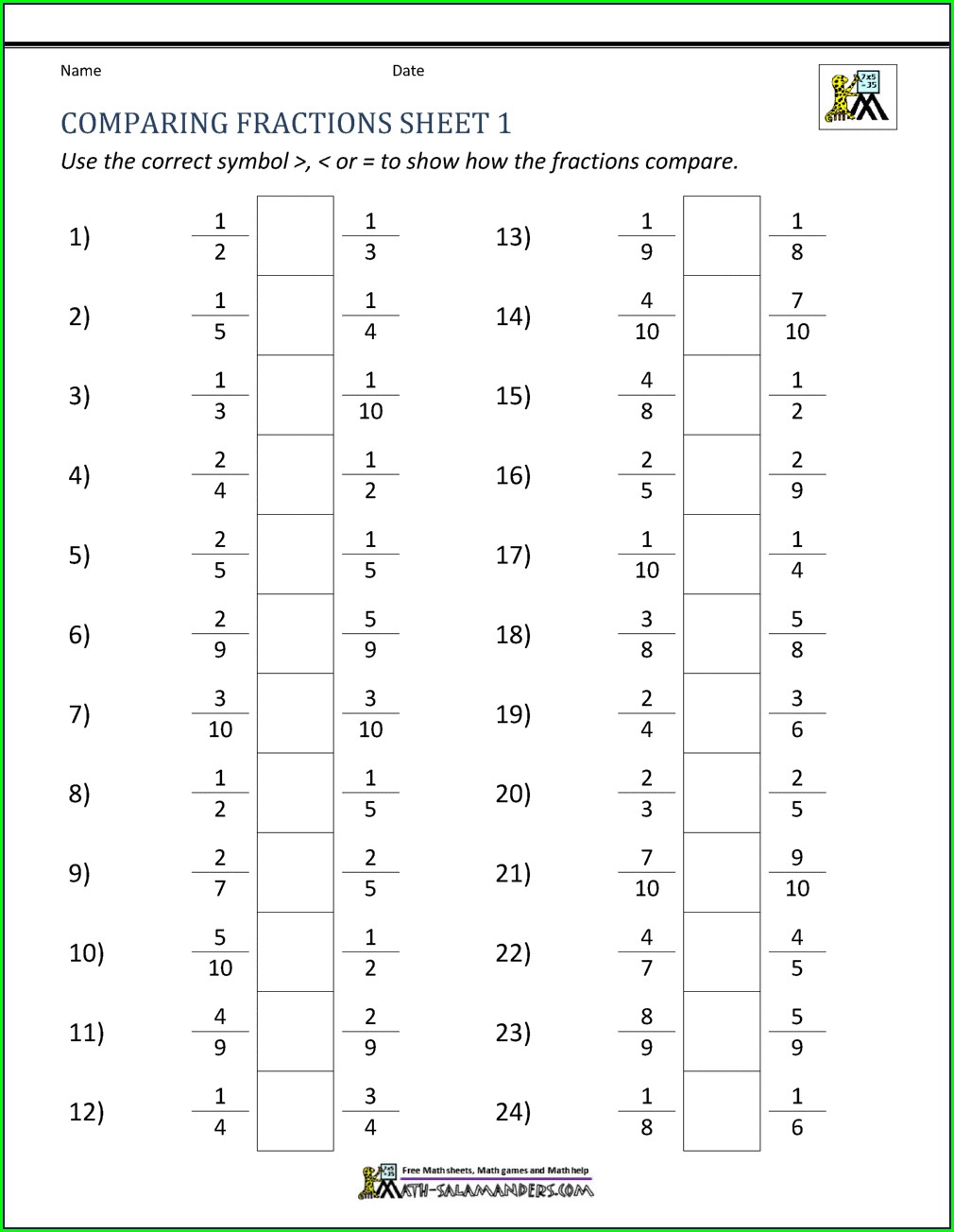
Comparing Fractions with the Same Denominator: A Simplified Guide
When it comes to comparing fractions, it can be a daunting task, especially for those who are new to the concept. However, with the right approach and techniques, it can be made easy. In this article, we will explore how to compare fractions with the same denominator, making it a simplified process for you.
Understanding Fractions
Before we dive into comparing fractions, let’s take a quick look at what fractions are. A fraction is a way to express a part of a whole. It consists of two parts: the numerator (the top number) and the denominator (the bottom number). The numerator tells us how many equal parts we have, and the denominator tells us how many parts the whole is divided into.
Same Denominator: What Does it Mean?
When we compare fractions with the same denominator, it means that the bottom numbers (the denominators) are the same. For example, 1⁄4 and 3⁄4 have the same denominator, which is 4.
How to Compare Fractions with the Same Denominator
Comparing fractions with the same denominator is relatively easy. Here’s a step-by-step guide:
- Step 1: Check the Denominators Make sure the denominators of the two fractions are the same. If they are not, you cannot compare them directly.
- Step 2: Compare the Numerators Look at the numerators (the top numbers) of the two fractions. The fraction with the larger numerator is greater.
📝 Note: When comparing fractions with the same denominator, you only need to compare the numerators.
Examples
Let’s take a look at some examples to illustrate this concept:
- Example 1: Compare 2⁄5 and 3⁄5 Since the denominators are the same (5), we only need to compare the numerators. 3 is greater than 2, so 3⁄5 is greater than 2⁄5.
- Example 2: Compare 1⁄8 and 5⁄8 Again, the denominators are the same (8), so we compare the numerators. 5 is greater than 1, so 5⁄8 is greater than 1⁄8.
Practice Time!
Now it’s your turn to practice comparing fractions with the same denominator. Here are some exercises to help you get started:
- Compare 2⁄3 and 5⁄3
- Compare 1⁄2 and 3⁄2
- Compare 4⁄9 and 7⁄9
Conclusion
Comparing fractions with the same denominator is a straightforward process. By following the simple steps outlined above, you can easily compare fractions and determine which one is greater. Remember, when the denominators are the same, you only need to compare the numerators.
What is the first step in comparing fractions with the same denominator?
+The first step is to check if the denominators of the two fractions are the same.
Why do we only need to compare the numerators when the denominators are the same?
+When the denominators are the same, the size of the fraction is determined by the numerator. Therefore, we only need to compare the numerators to determine which fraction is greater.
Can I compare fractions with different denominators using this method?
+No, this method only works when the denominators are the same. If the denominators are different, you need to use a different method, such as finding the least common multiple (LCM) or converting to equivalent fractions.
Related Terms:
- Comparing fractions Worksheet with answers
- Comparing fractions same denominator
- Comparing fractions with same numerator
