5 Ways to Compare and Order Integers

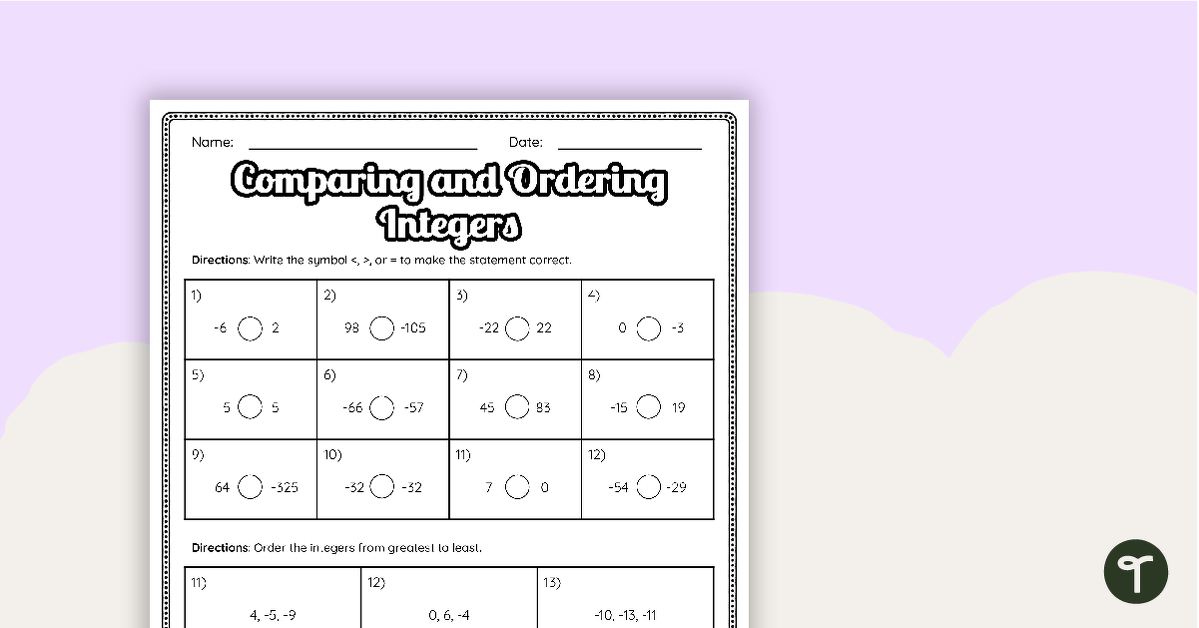
Understanding Integers and Their Comparison

Integers are whole numbers, either positive, negative, or zero, without a fractional part. Comparing and ordering integers is a fundamental concept in mathematics, essential for various mathematical operations and real-life applications. In this article, we will explore five ways to compare and order integers, including their representation on the number line, using inequality symbols, comparing absolute values, understanding the concept of opposites, and applying the rules for multiplying and dividing integers.

1. Using the Number Line

The number line is a visual representation of integers, where numbers are plotted on a line, with zero in the middle. Positive integers are plotted to the right of zero, and negative integers are plotted to the left. To compare integers using the number line, you can follow these steps:

• Plot the integers on the number line.
• The integer that is plotted to the right is greater.
• The integer that is plotted to the left is lesser.

For example, to compare -3 and 2, plot both numbers on the number line. Since 2 is plotted to the right of -3, we can conclude that 2 is greater than -3.

2. Using Inequality Symbols

Inequality symbols, such as < (less than), > (greater than), ≤ (less than or equal to), and ≥ (greater than or equal to), can be used to compare integers. For example:

• -5 < 0 (negative five is less than zero)
• 10 > -2 (ten is greater than negative two)
• 7 ≤ 7 (seven is less than or equal to seven)
• -3 ≥ -5 (negative three is greater than or equal to negative five)

3. Comparing Absolute Values

The absolute value of an integer is its distance from zero on the number line. To compare integers using their absolute values, follow these steps:

• Calculate the absolute value of each integer.
• The integer with the greater absolute value is greater.

For example, to compare -12 and 8, calculate their absolute values: |-12| = 12 and |8| = 8. Since 12 is greater than 8, we can conclude that -12 is less than 8.

4. Understanding Opposites

Opposites are integers that have the same absolute value but opposite signs. For example, 5 and -5 are opposites. To compare integers using opposites, follow these steps:

• Check if the integers are opposites.
• If they are, then one is positive and the other is negative.
• The positive integer is greater.

For example, to compare 5 and -5, we can see that they are opposites. Since 5 is positive, we can conclude that 5 is greater than -5.

5. Multiplying and Dividing Integers

When multiplying or dividing integers, the rules of signs must be followed:

• Multiplying two integers with the same sign results in a positive product.
• Multiplying two integers with different signs results in a negative product.
• Dividing two integers with the same sign results in a positive quotient.
• Dividing two integers with different signs results in a negative quotient.

For example, to compare the products of 2 × 3 and -2 × 3, we can calculate the products: 2 × 3 = 6 and -2 × 3 = -6. Since 6 is greater than -6, we can conclude that 2 × 3 is greater than -2 × 3.

📝 Note: When comparing integers, it is essential to consider the signs and the absolute values of the numbers.

By understanding and applying these five ways to compare and order integers, you can develop a solid foundation in mathematics and improve your problem-solving skills.

Related Terms:

• Comparing integers worksheet grade 7
• Comparison of integers worksheet
• Ordering Integers Worksheet grade 6