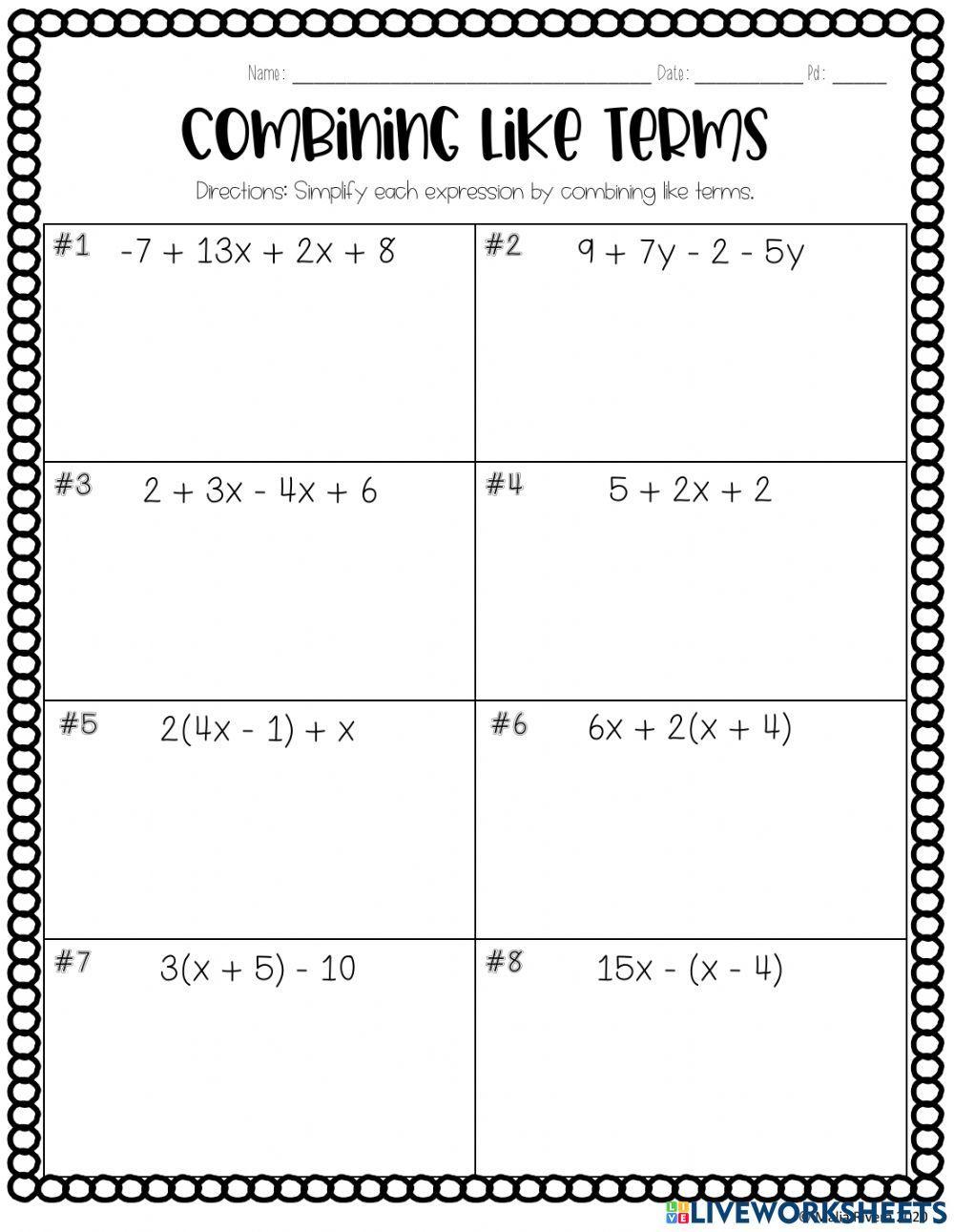
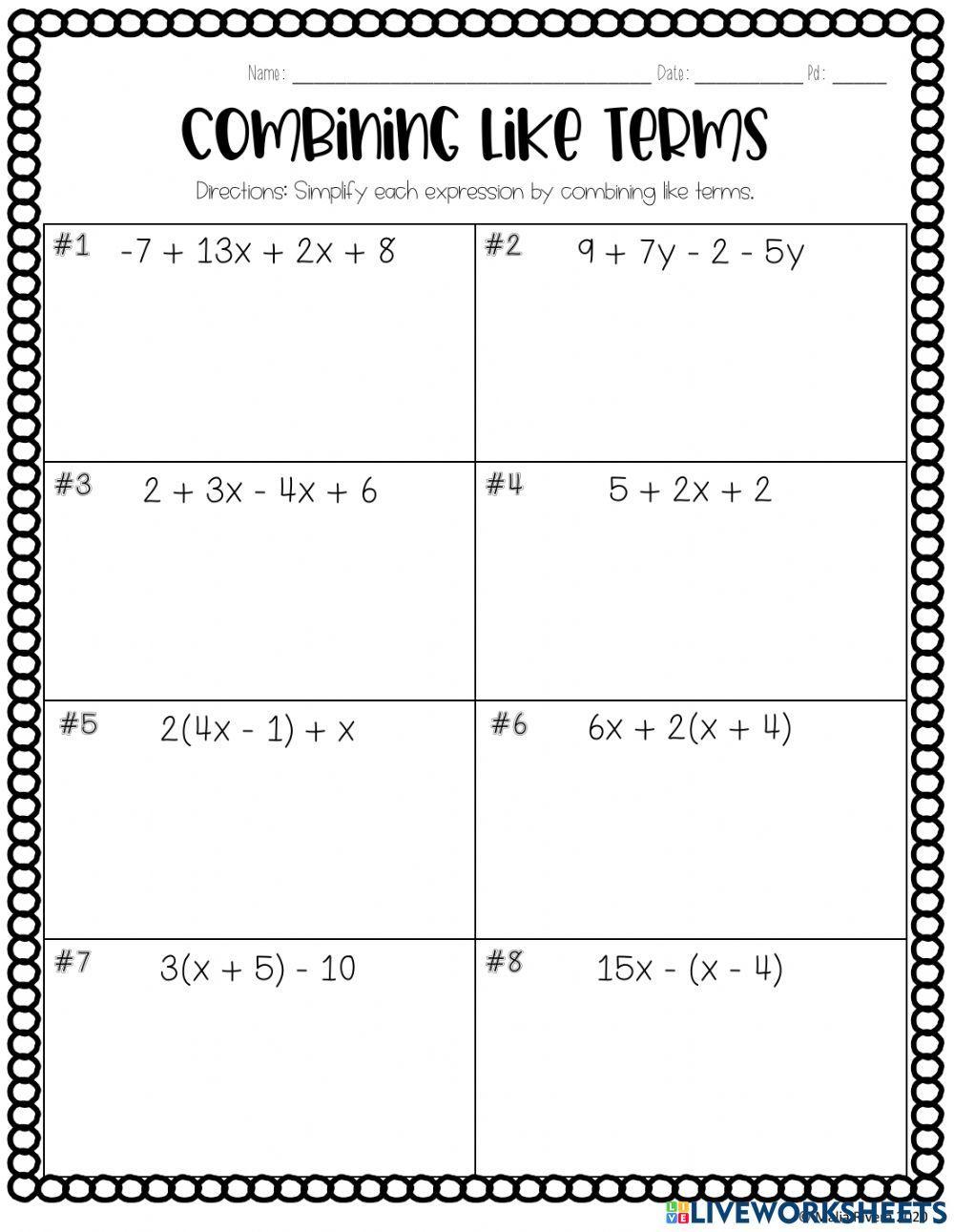
5 Ways to Master Combining Like Terms Worksheets
Mastering Combining Like Terms: Essential Strategies for Success
When it comes to algebra, one of the most crucial skills to master is combining like terms. This fundamental concept is used to simplify expressions and solve equations. However, for many students, combining like terms can be a daunting task. In this article, we will explore five effective strategies for mastering combining like terms worksheets.
Understanding Like Terms
Before we dive into the strategies, it’s essential to understand what like terms are. Like terms are terms that have the same variable(s) raised to the same power. For example, 2x and 3x are like terms because they both have the variable x raised to the power of 1. On the other hand, 2x and 3y are not like terms because they have different variables.
Strategy 1: Identifying Like Terms
The first step in combining like terms is to identify the like terms in an expression. This requires careful attention to the variables and their exponents. Here are a few examples:
- 2x + 3x + 4y (like terms: 2x and 3x)
- 5x^2 + 2x^2 + 3x (like terms: 5x^2 and 2x^2)
- 3y + 2y + 4z (like terms: 3y and 2y)
Once you have identified the like terms, you can proceed to combine them.
Strategy 2: Using the Commutative Property
The commutative property states that the order of the terms does not affect the result. This means that you can rearrange the terms in an expression to make it easier to combine like terms. For example:
- 2x + 3y + 4x = 2x + 4x + 3y (using the commutative property)
- 5x^2 + 3x + 2x^2 = 5x^2 + 2x^2 + 3x (using the commutative property)
By rearranging the terms, you can group the like terms together and make it easier to combine them.
Strategy 3: Using the Associative Property
The associative property states that you can group terms in different ways without affecting the result. This means that you can use parentheses to group like terms together. For example:
- (2x + 3x) + 4y = 5x + 4y (using the associative property)
- (5x^2 + 2x^2) + 3x = 7x^2 + 3x (using the associative property)
By using the associative property, you can group like terms together and simplify the expression.
Strategy 4: Distributing Coefficients
When combining like terms, you may need to distribute coefficients to the variables. For example:
- 2(x + 3) = 2x + 6 (distributing the coefficient 2)
- 3(2x + 4) = 6x + 12 (distributing the coefficient 3)
By distributing the coefficients, you can simplify the expression and combine like terms.
Strategy 5: Using Visual Aids
Finally, using visual aids such as charts or diagrams can help you master combining like terms. For example, you can create a chart with the variables on one axis and the coefficients on the other axis. This can help you visualize the like terms and combine them more easily.
| Variable | Coefficient |
|---|---|
| x | 2 |
| x | 3 |
| y | 4 |
By using visual aids, you can make combining like terms more engaging and fun.
📝 Note: Practice is key to mastering combining like terms. Make sure to practice regularly and use a variety of strategies to reinforce your understanding.
As you can see, mastering combining like terms requires a combination of strategies and practice. By identifying like terms, using the commutative and associative properties, distributing coefficients, and using visual aids, you can become proficient in combining like terms. Remember to practice regularly and use a variety of strategies to reinforce your understanding.
In conclusion, combining like terms is an essential skill in algebra that requires practice and patience. By using the strategies outlined in this article, you can master combining like terms and improve your overall math skills.
What are like terms in algebra?
+Like terms are terms that have the same variable(s) raised to the same power.
How do I identify like terms in an expression?
+Identify like terms by looking for terms that have the same variable(s) raised to the same power.
What is the commutative property in algebra?
+The commutative property states that the order of the terms does not affect the result.