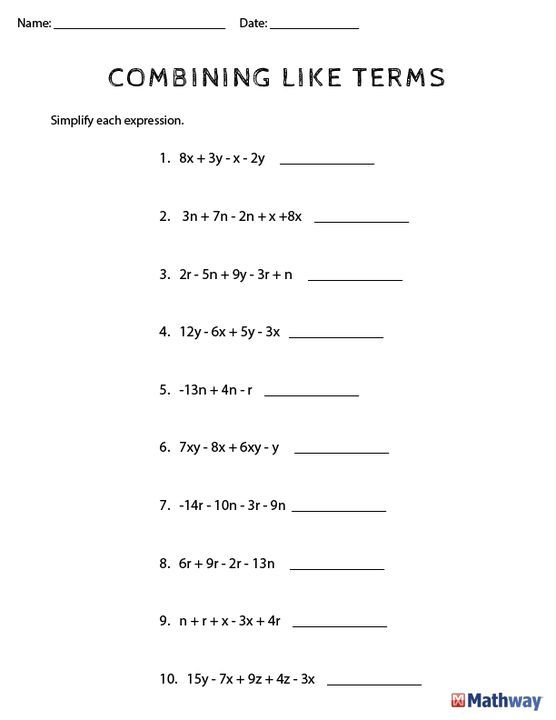
5 Ways to Simplify Combine Like Terms

Mastering Algebra: Simplifying Combine Like Terms Made Easy
Algebra can be a daunting subject for many students, but with the right strategies, it can be made more manageable. One of the key concepts in algebra is simplifying expressions by combining like terms. In this article, we will explore five ways to simplify combine like terms, making it easier for you to tackle algebra problems with confidence.
Understanding Like Terms
Before we dive into the methods, it’s essential to understand what like terms are. Like terms are terms that have the same variable(s) with the same exponent. For example, 2x and 3x are like terms because they both have the variable x with the same exponent (1). On the other hand, 2x and 3y are not like terms because they have different variables.
Method 1: Identifying and Combining Like Terms
The first method is to identify the like terms in an expression and combine them. For example, consider the expression:
2x + 3x + 4y
To simplify this expression, we can combine the like terms 2x and 3x:
2x + 3x = 5x
So, the simplified expression becomes:
5x + 4y
🔍 Note: When combining like terms, make sure to add or subtract the coefficients (numbers in front of the variables) correctly.
Method 2: Using the Distributive Property
The distributive property is a powerful tool for simplifying expressions. It states that for any numbers a, b, and c:
a(b + c) = ab + ac
We can use this property to simplify expressions by distributing the coefficients to the variables. For example, consider the expression:
2(x + 3)
Using the distributive property, we can rewrite this expression as:
2x + 6
Method 3: Combining Like Terms with Exponents
When working with exponents, we need to be careful when combining like terms. For example, consider the expression:
2x^2 + 3x^2
To simplify this expression, we can combine the like terms:
2x^2 + 3x^2 = 5x^2
However, if the exponents are different, we cannot combine the terms. For example:
2x^2 + 3x^3
These terms cannot be combined because they have different exponents.
Method 4: Simplifying Expressions with Multiple Variables
When working with expressions that have multiple variables, we need to identify the like terms carefully. For example, consider the expression:
2xy + 3xy + 4xz
To simplify this expression, we can combine the like terms:
2xy + 3xy = 5xy
So, the simplified expression becomes:
5xy + 4xz
Method 5: Using a Table to Organize Like Terms
Sometimes, it can be helpful to use a table to organize like terms. For example, consider the expression:
2x + 3x + 4y + 5y
We can create a table to organize the like terms:
| Term | Coefficient | Variable |
|---|---|---|
| 2x | 2 | x |
| 3x | 3 | x |
| 4y | 4 | y |
| 5y | 5 | y |
Using the table, we can combine the like terms:
2x + 3x = 5x 4y + 5y = 9y
So, the simplified expression becomes:
5x + 9y
By following these five methods, you can simplify expressions by combining like terms with ease. Remember to always identify the like terms carefully and combine them correctly.
To wrap up, simplifying combine like terms is a crucial skill in algebra that can make solving equations and manipulating expressions much easier. By mastering these five methods, you’ll be well on your way to becoming an algebra pro!
What are like terms in algebra?
+Like terms are terms that have the same variable(s) with the same exponent.
How do I combine like terms in an expression?
+To combine like terms, identify the like terms and add or subtract their coefficients (numbers in front of the variables) correctly.
What is the distributive property in algebra?
+The distributive property states that for any numbers a, b, and c: a(b + c) = ab + ac.
Related Terms:
- Combining like terms Equations Worksheet