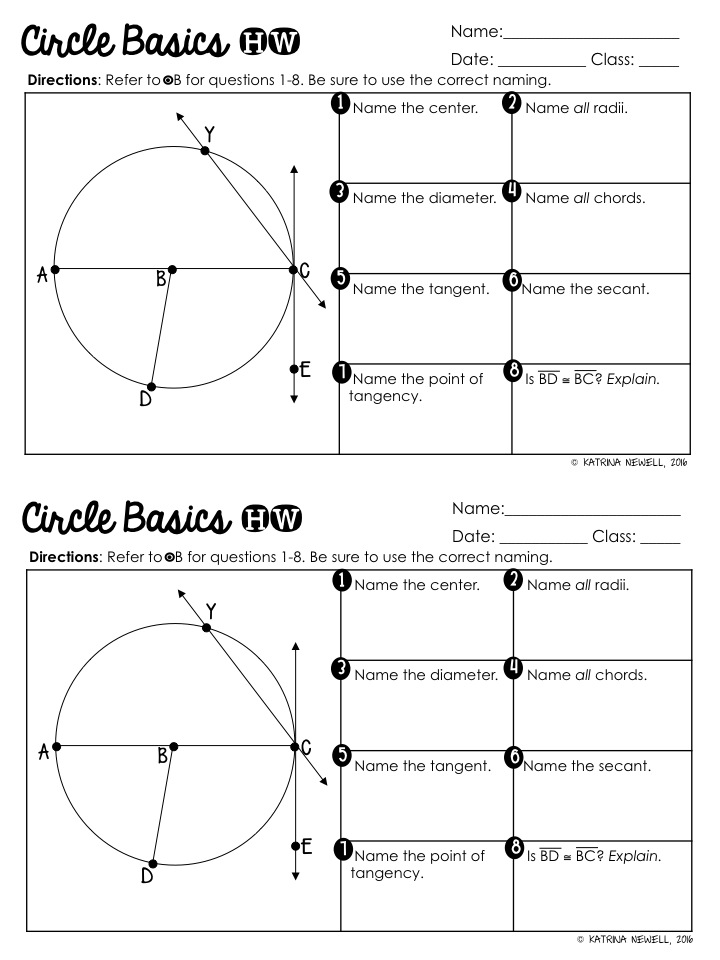
Circle Basics Answer Key Day 1 Worksheet

Circle Basics Answer Key Day 1 Worksheet
The concept of circles is a fundamental aspect of geometry, and understanding its basics is crucial for problem-solving in mathematics. Here’s a comprehensive guide to help you grasp the essential elements of circles, along with a detailed answer key for a day 1 worksheet.
Key Components of a Circle
Before diving into the worksheet, let’s review the key components of a circle:
- Center: The central point of the circle, often represented by the letter “O”.
- Radius: The distance from the center to any point on the circle’s circumference.
- Diameter: The longest distance across the circle, passing through its center.
- Circumference: The distance around the circle.
- Chord: A straight line connecting two points on the circle’s circumference.
- Arc: A portion of the circle’s circumference.
Worksheet Answer Key
Here’s the answer key for the day 1 worksheet on circle basics:
| Question # | Question | Answer |
|---|---|---|
| 1 | What is the center of a circle? | The central point of the circle |
| 2 | What is the radius of a circle? | The distance from the center to any point on the circumference |
| 3 | What is the diameter of a circle? | The longest distance across the circle, passing through its center |
| 4 | What is the circumference of a circle? | The distance around the circle |
| 5 | What is a chord in a circle? | A straight line connecting two points on the circumference |
| 6 | What is an arc in a circle? | A portion of the circle’s circumference |
| 7 | If the radius of a circle is 4 cm, what is its diameter? | 8 cm |
| 8 | If the diameter of a circle is 10 cm, what is its radius? | 5 cm |
| 9 | What is the formula for the circumference of a circle? | C = 2πr or C = πd |
| 10 | What is the formula for the area of a circle? | A = πr^2 |
📝 Note: π (pi) is approximately equal to 3.14
Important Formulas and Relationships
Here are some essential formulas and relationships to remember:
- Circumference © = 2πr or C = πd
- Area (A) = πr^2
- Diameter (d) = 2r
- Radius ® = d/2
Understanding these formulas and relationships will help you solve problems involving circles.
Real-World Applications of Circles
Circles are present in various aspects of our daily lives, from the shape of a coin to the curvature of a satellite dish. Understanding the basics of circles can help you solve problems in:
- Architecture
- Engineering
- Physics
- Computer Science
- Art and Design
In conclusion, circles are a fundamental aspect of geometry, and understanding their basics is crucial for problem-solving in mathematics. By mastering the key components, formulas, and relationships, you’ll be well-equipped to tackle more complex problems and appreciate the real-world applications of circles.
What is the center of a circle?
+
The center of a circle is the central point, often represented by the letter “O”.
What is the formula for the circumference of a circle?
+
The formula for the circumference of a circle is C = 2πr or C = πd.
What is the relationship between the radius and diameter of a circle?
+
The diameter of a circle is twice the radius (d = 2r), and the radius is half the diameter (r = d/2).