5 Ways to Master the Chain Rule

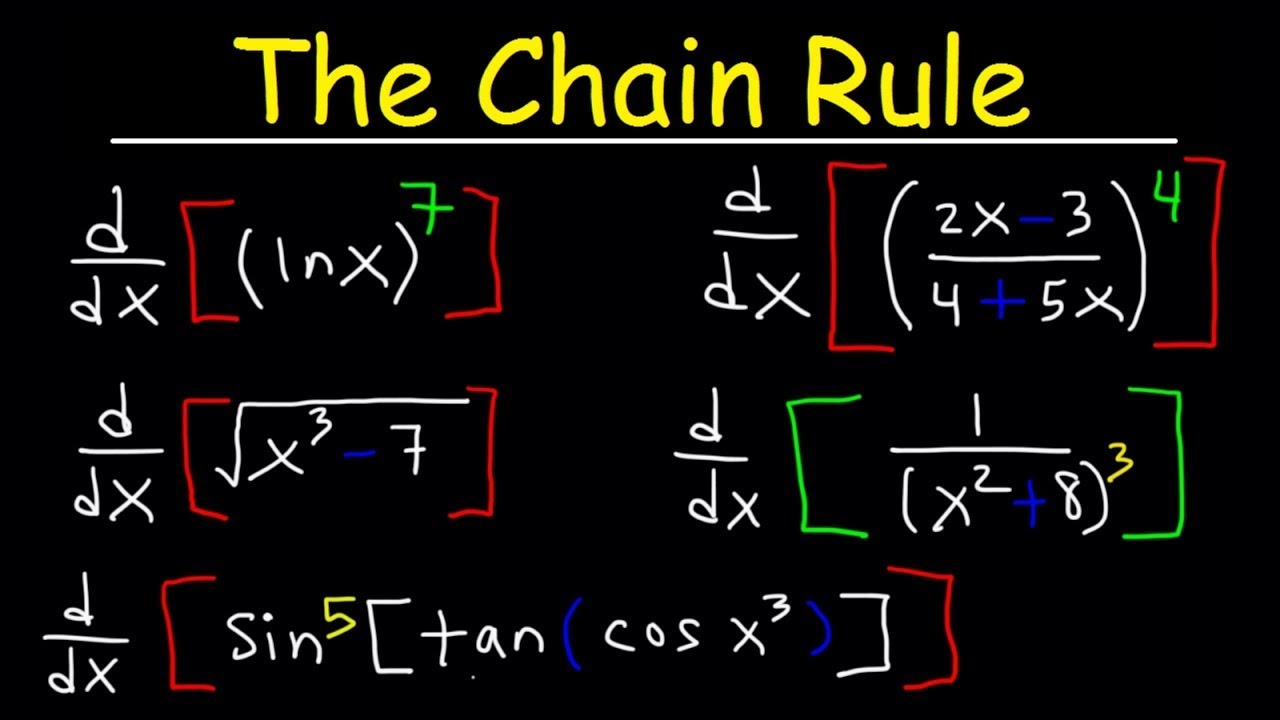
Unlocking the Power of the Chain Rule

The chain rule is a fundamental concept in calculus that is used to differentiate composite functions. It is a powerful tool that can help you solve complex problems in a wide range of fields, from physics and engineering to economics and computer science. However, mastering the chain rule can be challenging, especially for those who are new to calculus. In this article, we will explore five ways to master the chain rule and become proficient in using it to solve problems.

1. Understand the Concept of Composite Functions

Before we dive into the chain rule, it’s essential to understand the concept of composite functions. A composite function is a function that is composed of two or more functions. For example, if we have two functions, f(x) and g(x), we can create a composite function, h(x), by combining them:

h(x) = f(g(x))

The chain rule is used to differentiate composite functions like h(x). It states that the derivative of h(x) is equal to the derivative of f(x) multiplied by the derivative of g(x):

h’(x) = f’(g(x)) * g’(x)

2. Learn the Formula and Practice, Practice, Practice

The chain rule formula is:

h’(x) = f’(g(x)) * g’(x)

To master the chain rule, you need to practice using this formula to solve problems. Start with simple problems and gradually move on to more complex ones. You can find many practice problems online or in calculus textbooks.

Here’s an example problem:

Find the derivative of h(x) = (2x + 1)^3

Using the chain rule, we get:

h’(x) = d(2x + 1)^3/dx = 3(2x + 1)^2 * d(2x + 1)/dx = 3(2x + 1)^2 * 2 = 6(2x + 1)^2

📝 Note: When using the chain rule, make sure to identify the outer and inner functions correctly. The outer function is the function that is being composed, while the inner function is the function that is being composed with.

3. Use the Chain Rule to Differentiate Trigonometric Functions

The chain rule is particularly useful when differentiating trigonometric functions. For example, if we want to find the derivative of sin(2x), we can use the chain rule:

sin(2x)’ = cos(2x) * d(2x)/dx = cos(2x) * 2 = 2cos(2x)

Similarly, if we want to find the derivative of cos(3x), we can use the chain rule:

cos(3x)’ = -sin(3x) * d(3x)/dx = -sin(3x) * 3 = -3sin(3x)

4. Master the Chain Rule for Exponential and Logarithmic Functions

The chain rule is also essential when differentiating exponential and logarithmic functions. For example, if we want to find the derivative of e^(2x), we can use the chain rule:

e^(2x)’ = e^(2x) * d(2x)/dx = e^(2x) * 2 = 2e^(2x)

Similarly, if we want to find the derivative of ln(3x), we can use the chain rule:

ln(3x)’ = 1/3x * d(3x)/dx = 1/3x * 3 = 1/x

5. Use the Chain Rule to Solve Real-World Problems

The chain rule is not just a theoretical concept; it has many practical applications in real-world problems. For example, in physics, the chain rule is used to describe the motion of objects. In economics, it is used to model the behavior of markets.

Here’s an example problem:

A company’s profit is given by the function:

P(x) = 2x^2 + 10x + 5

where x is the number of units sold. The company’s revenue is given by the function:

R(x) = 3x^2 + 20x + 10

Find the rate at which the profit is changing when the revenue is changing at a rate of $100 per unit.

Using the chain rule, we get:

dP/dR = dP/dx * dx/dR = (4x + 10) * (1/(6x + 20))

Substituting x = 10, we get:

dP/dR = (40 + 10) * (1/(60 + 20)) = ⁵⁰⁄₈₀ = ⁵⁄₈

This means that the profit is changing at a rate of 5/8 per unit when the revenue is changing at a rate of 100 per unit.

When it comes to mastering the chain rule, practice is key. With consistent practice and a deep understanding of the concept, you can become proficient in using the chain rule to solve a wide range of problems. Whether you’re a student or a professional, the chain rule is an essential tool that can help you unlock the power of calculus.