5 Essential Inequality Problems to Solve
Understanding Inequality: A Foundational Concept in Mathematics
Inequality is a fundamental concept in mathematics that deals with the relationship between two quantities. It is used to compare the magnitude of two values and determine which one is greater or lesser. Inequality problems are encountered in various mathematical disciplines, including algebra, geometry, and calculus. In this article, we will explore five essential inequality problems to solve, which will help you develop a deeper understanding of this concept.
Problem 1: Linear Inequality
Solve the inequality 2x + 5 > 11.
This is a linear inequality, which means it can be solved by isolating the variable x. To solve this inequality, we need to isolate x by subtracting 5 from both sides of the inequality.
2x + 5 > 11
Subtracting 5 from both sides gives us:
2x > 6
Dividing both sides by 2 gives us:
x > 3
Therefore, the solution to the inequality is x > 3.
📝 Note: When solving linear inequalities, it is essential to remember that the direction of the inequality sign remains the same when adding or subtracting the same value from both sides. However, the direction of the inequality sign changes when multiplying or dividing both sides by a negative number.
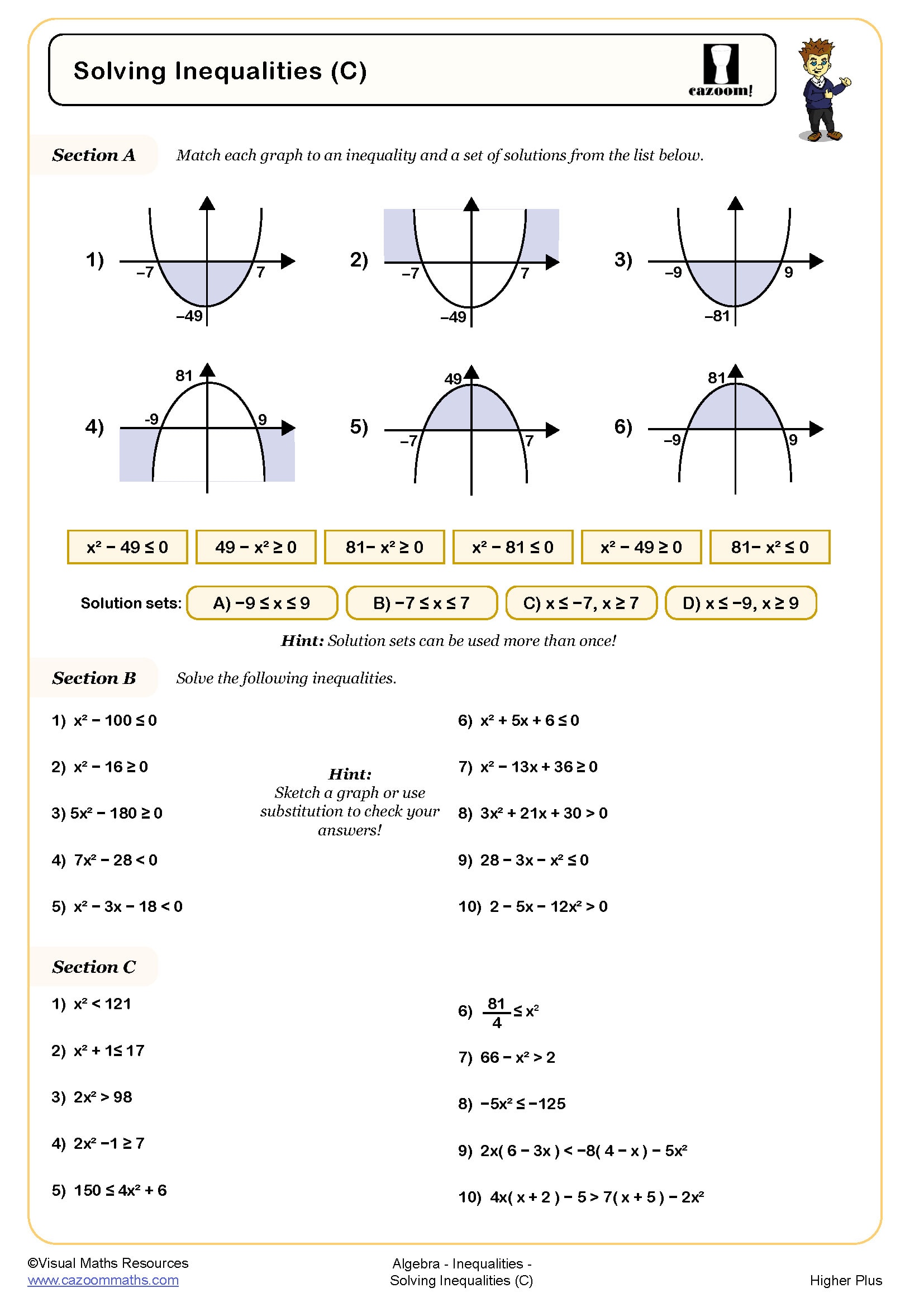
Problem 2: Quadratic Inequality
Solve the inequality x^2 + 4x + 4 ≤ 0.
This is a quadratic inequality, which means it can be solved by factoring or using the quadratic formula. To solve this inequality, we can factor the left-hand side of the inequality.
x^2 + 4x + 4 ≤ 0
Factoring the left-hand side gives us:
(x + 2)(x + 2) ≤ 0
This inequality can be rewritten as:
(x + 2)^2 ≤ 0
The square of any real number is always non-negative, so the only way for this inequality to be true is if x + 2 = 0.
x + 2 = 0
Subtracting 2 from both sides gives us:
x = -2
Therefore, the solution to the inequality is x = -2.
Problem 3: Rational Inequality
Solve the inequality (x + 1)/(x - 1) > 0.
This is a rational inequality, which means it involves a fraction with variables in the numerator and denominator. To solve this inequality, we need to find the critical points where the numerator and denominator are equal to zero.
The critical points are x = -1 and x = 1.
We can use a number line to test the intervals between the critical points.

| Interval | (x + 1) | (x - 1) | (x + 1)/(x - 1) |
|---|---|---|---|
| (-∞, -1) | - | - | + |
| (-1, 1) | + | - | - |
| (1, ∞) | + | + | + |
The intervals where the inequality is true are (-∞, -1) and (1, ∞).
Therefore, the solution to the inequality is x ∈ (-∞, -1) ∪ (1, ∞).
Problem 4: Absolute Value Inequality
Solve the inequality |x - 2| > 3.
This is an absolute value inequality, which means it involves the absolute value of an expression. To solve this inequality, we need to find the values of x that satisfy the inequality.
|x - 2| > 3
This inequality can be rewritten as:
x - 2 > 3 or x - 2 < -3
Solving the first inequality gives us:
x > 5
Solving the second inequality gives us:
x < -1
Therefore, the solution to the inequality is x > 5 or x < -1.
Problem 5: Compound Inequality
Solve the inequality -2 ≤ 3x - 2 ≤ 5.
This is a compound inequality, which means it involves two inequalities that must be true simultaneously. To solve this inequality, we need to isolate the variable x.
-2 ≤ 3x - 2 ≤ 5
Adding 2 to all parts of the inequality gives us:
0 ≤ 3x ≤ 7
Dividing all parts of the inequality by 3 gives us:
0 ≤ x ≤ 7⁄3
Therefore, the solution to the inequality is 0 ≤ x ≤ 7⁄3.
These five essential inequality problems to solve will help you develop a deeper understanding of this fundamental concept in mathematics. By practicing and solving these problems, you will become more confident and proficient in your ability to solve inequality problems.
In conclusion, inequality problems are an essential part of mathematics, and solving them requires a combination of algebraic techniques and logical reasoning. By mastering these techniques, you will be able to solve a wide range of inequality problems and apply them to real-world applications.
What is the difference between an equation and an inequality?
+An equation is a statement that expresses the equality of two mathematical expressions, whereas an inequality is a statement that expresses the relationship between two expressions, such as greater than, less than, or equal to.
How do you solve a linear inequality?
+To solve a linear inequality, you need to isolate the variable by adding, subtracting, multiplying, or dividing both sides of the inequality by the same value. The direction of the inequality sign remains the same when adding or subtracting the same value from both sides, but changes when multiplying or dividing both sides by a negative number.
What is the purpose of critical points in solving rational inequalities?
+Critical points are used to divide the number line into intervals where the inequality is either true or false. By testing a value from each interval, you can determine which intervals satisfy the inequality.
Related Terms:
- Basic Inequalities Worksheet pdf
- Inequalities Worksheet PDF with answers
- Inequalities Worksheet with answers
- Inequalities word problems Worksheet
- Two-step Inequalities Worksheet
- Inequality Worksheet PDF