5 Ways to Master Average Rate of Change
Unlocking the Power of Average Rate of Change
The average rate of change is a fundamental concept in calculus, and it’s a crucial tool for understanding how functions change over time. In this article, we’ll explore five ways to master the average rate of change, from basic definitions to advanced applications.
What is Average Rate of Change?
The average rate of change is a measure of how a function changes over a given interval. It’s calculated by finding the difference in the function’s values at the beginning and end of the interval, and then dividing by the length of the interval. Mathematically, it’s represented as:
Average Rate of Change = (f(b) - f(a)) / (b - a)
where f(x) is the function, a and b are the endpoints of the interval, and f(a) and f(b) are the function values at those endpoints.
1. Understanding the Basics
To master the average rate of change, you need to understand the basics. Here are some key points to get you started:
- The average rate of change is a measure of the function’s change over a specific interval.
- It’s calculated using the formula: (f(b) - f(a)) / (b - a)
- The average rate of change can be positive, negative, or zero, depending on the direction of the function’s change.
- It’s a useful tool for understanding how functions behave over time.
2. Visualizing the Average Rate of Change
Visualizing the average rate of change can help you understand how it works. Here are some ways to visualize it:
- Graph the function over the given interval.
- Draw a secant line between the endpoints of the interval.
- The slope of the secant line represents the average rate of change.
By visualizing the average rate of change, you can see how the function changes over the interval and how the average rate of change relates to the function’s values.
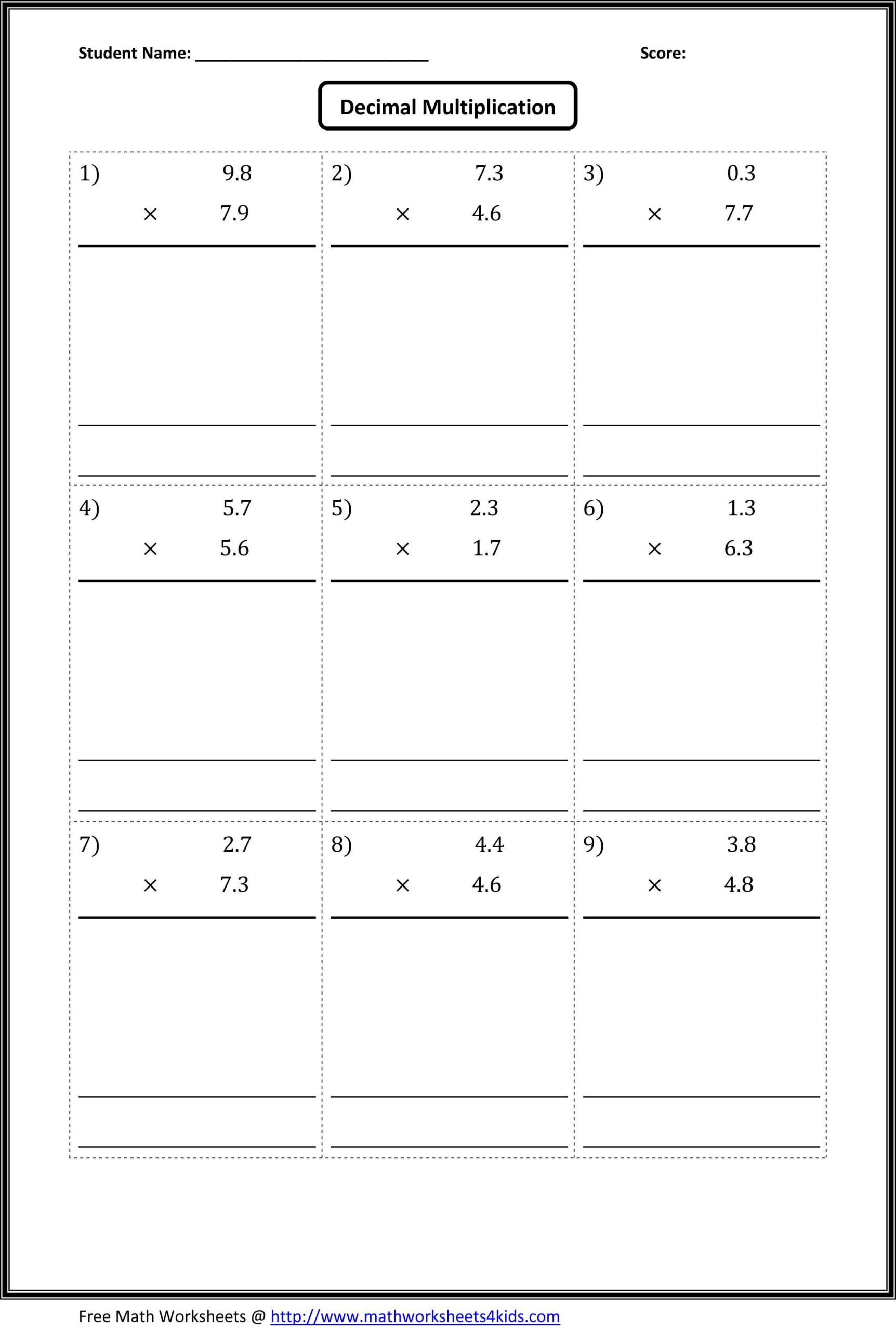
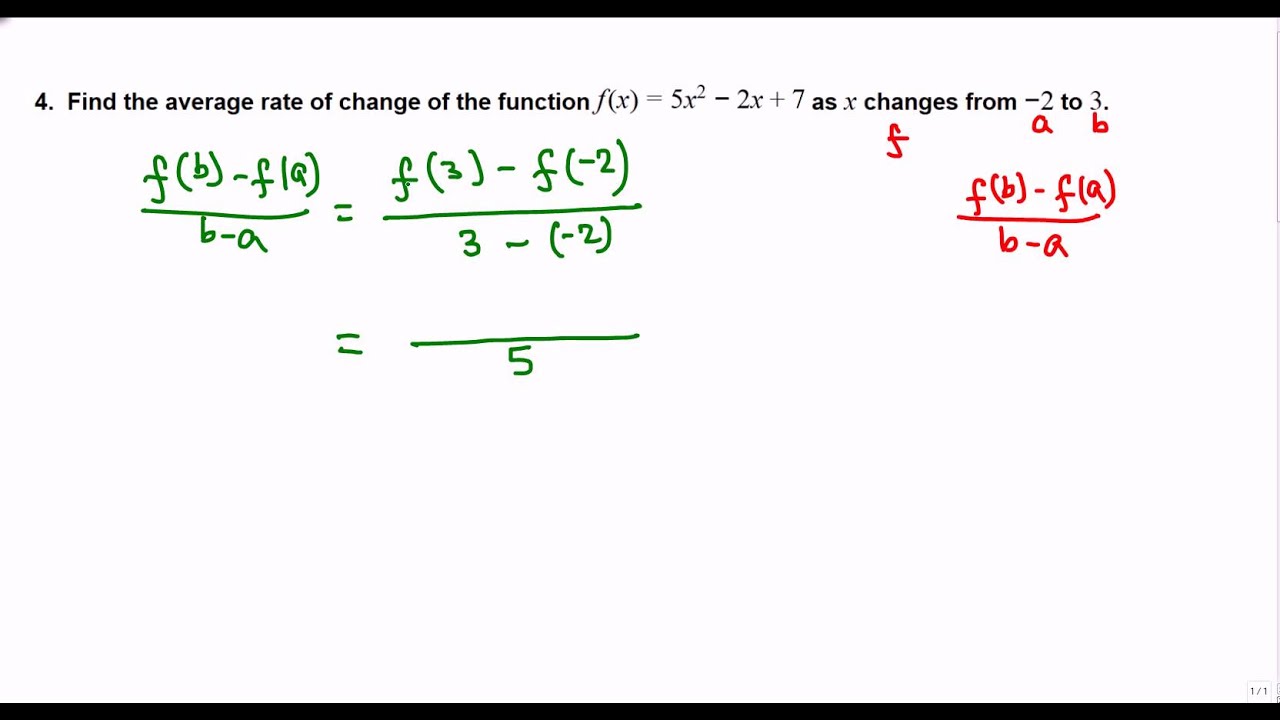
3. Calculating the Average Rate of Change
Now that you understand the basics and how to visualize the average rate of change, let’s calculate it. Here’s an example:
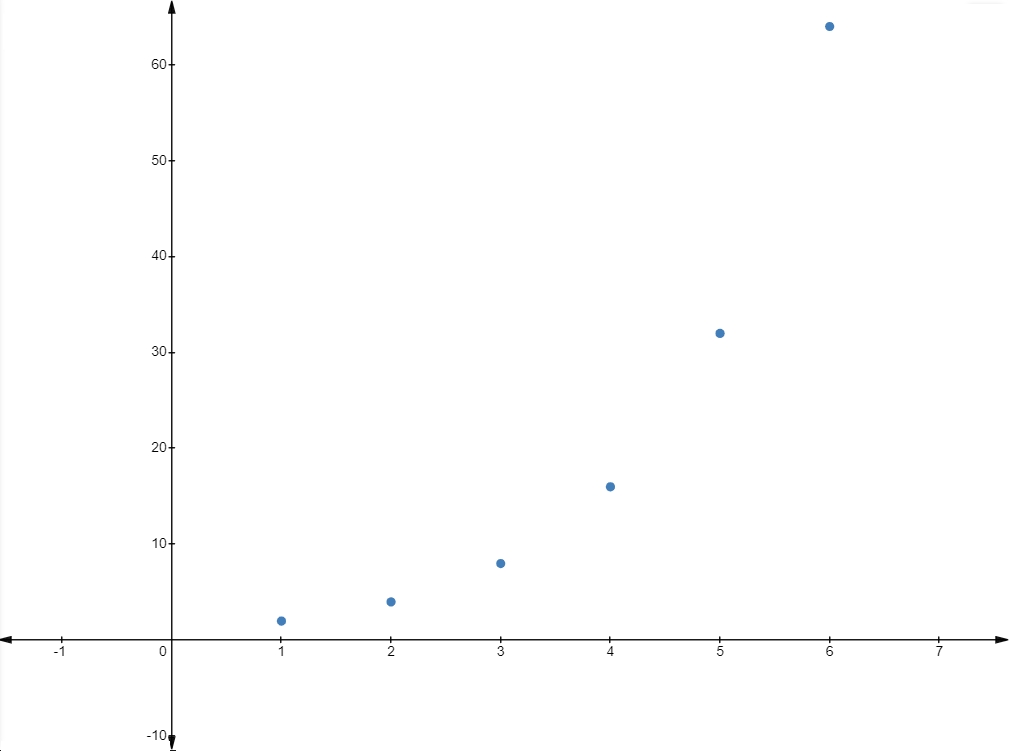
| x | f(x) |
|---|---|
| 0 | 2 |
| 2 | 6 |
To calculate the average rate of change, we use the formula:
Average Rate of Change = (f(2) - f(0)) / (2 - 0) = (6 - 2) / 2 = 4 / 2 = 2
The average rate of change is 2, which means the function increases by 2 units for every 1 unit increase in x.
4. Applying the Average Rate of Change
The average rate of change has many real-world applications. Here are a few examples:
- Physics: The average rate of change can be used to calculate the average velocity of an object over a given time interval.
- Economics: The average rate of change can be used to calculate the average rate of return on investment over a given time period.
- Biology: The average rate of change can be used to calculate the average rate of growth of a population over a given time period.
By applying the average rate of change, you can gain insights into how functions behave in different contexts.
5. Advanced Applications
The average rate of change has many advanced applications in calculus and beyond. Here are a few examples:
- Instantaneous Rate of Change: The average rate of change can be used to calculate the instantaneous rate of change, which is the rate of change at a single point.
- Derivatives: The average rate of change is closely related to derivatives, which are used to calculate the rate of change of a function at a single point.
- Multivariable Calculus: The average rate of change can be extended to multivariable calculus, where it’s used to calculate the rate of change of a function of multiple variables.
By exploring these advanced applications, you can gain a deeper understanding of the average rate of change and its connections to other mathematical concepts.
📝 Note: The average rate of change is a fundamental concept in calculus, and it's essential to understand it before moving on to more advanced topics.
As you can see, mastering the average rate of change requires a combination of understanding the basics, visualizing the concept, calculating it, applying it to real-world problems, and exploring advanced applications. By following these five ways, you’ll be well on your way to becoming a master of the average rate of change.
The concepts of average rate of change have far-reaching implications in various fields, including physics, economics, and biology. Understanding these concepts can help you analyze and solve complex problems in these fields.
What is the formula for calculating the average rate of change?
+The formula for calculating the average rate of change is: (f(b) - f(a)) / (b - a)
What is the difference between the average rate of change and the instantaneous rate of change?
+The average rate of change is the rate of change over a given interval, while the instantaneous rate of change is the rate of change at a single point.
How is the average rate of change used in real-world applications?
+The average rate of change is used in various fields, including physics, economics, and biology, to calculate the rate of change of a function over a given interval.