7 Ways to Calculate Area of Compound Shapes
Introduction to Compound Shapes
Compound shapes are geometric figures that are composed of multiple simple shapes, such as triangles, quadrilaterals, polygons, and circles. These shapes are combined to form a new shape, which can have various properties and characteristics. One of the essential properties of compound shapes is their area, which is the total space occupied by the shape.
In this article, we will explore 7 ways to calculate the area of compound shapes. We will discuss the different types of compound shapes, their properties, and the formulas used to calculate their areas.
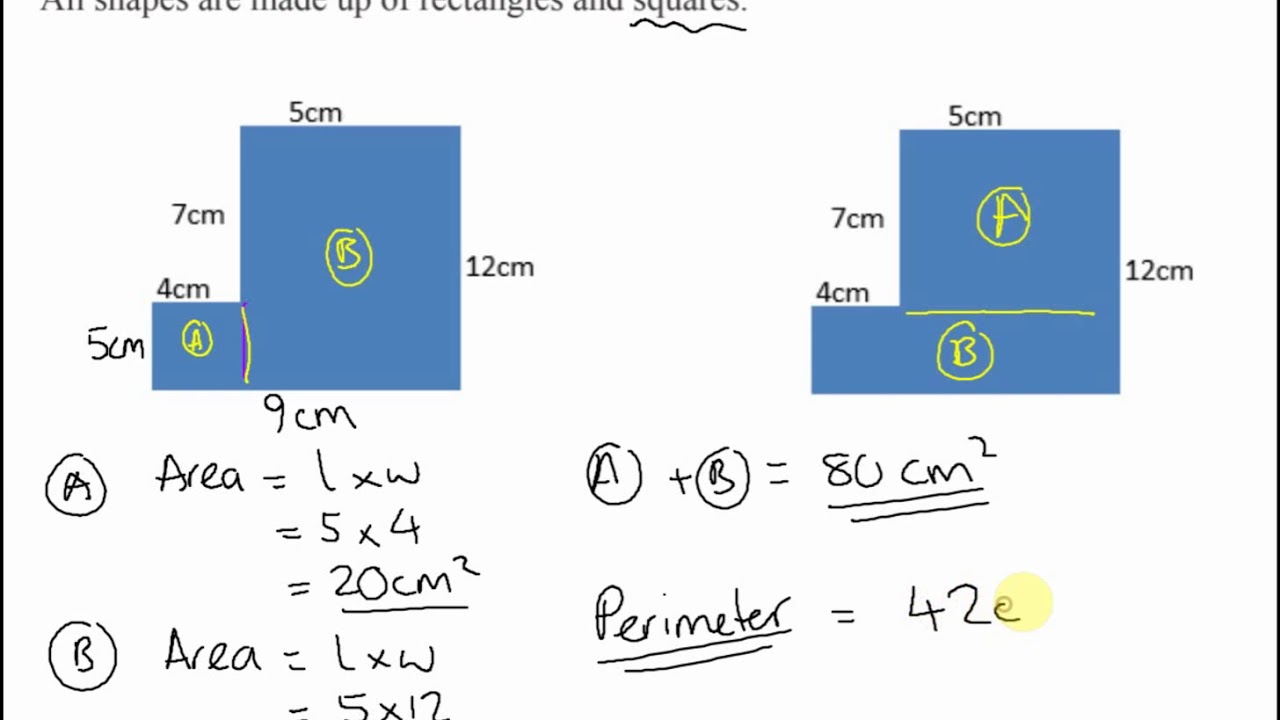
1. Decomposition Method
The decomposition method involves breaking down a compound shape into its individual simple shapes. Once the shape is decomposed, we can calculate the area of each individual shape and then add them up to find the total area of the compound shape.
For example, let’s consider a compound shape consisting of a rectangle and a triangle.
| Shape | Area Formula | Area Value |
|---|---|---|
| Rectangle | Length x Width | 10 x 5 = 50 |
| Triangle | 1/2 x Base x Height | 1/2 x 6 x 8 = 24 |
The total area of the compound shape is the sum of the areas of the rectangle and the triangle, which is 50 + 24 = 74.
2. Addition Method
The addition method involves adding the areas of two or more shapes to find the area of the compound shape. This method is useful when the shapes are not overlapping.
For example, let’s consider a compound shape consisting of two rectangles.
| Shape | Area Formula | Area Value |
|---|---|---|
| Rectangle 1 | Length x Width | 8 x 4 = 32 |
| Rectangle 2 | Length x Width | 6 x 3 = 18 |
The total area of the compound shape is the sum of the areas of the two rectangles, which is 32 + 18 = 50.
📝 Note: The addition method assumes that the shapes are not overlapping. If the shapes are overlapping, the area of the overlap must be subtracted from the total area.
3. Subtraction Method
The subtraction method involves subtracting the area of one shape from the area of another shape to find the area of the compound shape. This method is useful when one shape is a hole or an overlap.
For example, let’s consider a compound shape consisting of a large rectangle with a small rectangle hole.
| Shape | Area Formula | Area Value |
|---|---|---|
| Large Rectangle | Length x Width | 10 x 6 = 60 |
| Small Rectangle Hole | Length x Width | 2 x 2 = 4 |
The total area of the compound shape is the area of the large rectangle minus the area of the small rectangle hole, which is 60 - 4 = 56.
4. Tessellation Method
The tessellation method involves dividing a compound shape into smaller, non-overlapping shapes called tiles or tessellations. The area of each tile is calculated, and then the total area of the compound shape is found by adding up the areas of the tiles.
For example, let’s consider a compound shape consisting of a hexagon with a repeating pattern of equilateral triangles.
| Shape | Area Formula | Area Value |
|---|---|---|
| Equilateral Triangle | 1/2 x Base x Height | 1/2 x 4 x 4 = 8 |
The total area of the compound shape is the sum of the areas of the 6 equilateral triangles, which is 6 x 8 = 48.
5. Grid Method
The grid method involves superimposing a grid over a compound shape and counting the number of squares or rectangles that make up the shape. The area of each square or rectangle is calculated, and then the total area of the compound shape is found by adding up the areas of the grid cells.
For example, let’s consider a compound shape consisting of a rectangle with a triangular hole.
| Grid Cell | Area Formula | Area Value |
|---|---|---|
| 1 x 1 Square | Length x Width | 1 x 1 = 1 |
The total area of the compound shape is the sum of the areas of the 12 grid cells, which is 12 x 1 = 12.
6. Sector Method
The sector method involves dividing a compound shape into sectors or segments, and then calculating the area of each sector. The total area of the compound shape is found by adding up the areas of the sectors.
For example, let’s consider a compound shape consisting of a circle with a sector missing.
| Shape | Area Formula | Area Value |
|---|---|---|
| Circle | π x Radius^2 | 3.14 x 4^2 = 50.24 |
| Sector | 1/2 x Radius^2 x Angle | 1/2 x 4^2 x 60 = 24 |
The total area of the compound shape is the area of the circle minus the area of the sector, which is 50.24 - 24 = 26.24.
7. Coordinate Geometry Method
The coordinate geometry method involves using the coordinates of the vertices of a compound shape to calculate its area. This method is useful for complex shapes with many vertices.
For example, let’s consider a compound shape consisting of a polygon with 5 vertices.
| Vertex | X-Coordinate | Y-Coordinate |
|---|---|---|
| Vertex 1 | 0 | 0 |
| Vertex 2 | 3 | 4 |
| Vertex 3 | 6 | 8 |
| Vertex 4 | 9 | 4 |
| Vertex 5 | 12 | 0 |
The total area of the compound shape can be calculated using the Shoelace formula, which is:
Area = 1⁄2 x |(x1y2 + x2y3 + x3y4 + x4y5 + x5y1) - (x2y1 + x3y2 + x4y3 + x5y4 + x1y5)|
Using the coordinates of the vertices, the area of the compound shape can be calculated to be 54.
To summarize, there are many ways to calculate the area of compound shapes, including decomposition, addition, subtraction, tessellation, grid, sector, and coordinate geometry methods. Each method has its own strengths and weaknesses, and the choice of method depends on the specific shape and its properties.
What is a compound shape?
+A compound shape is a geometric figure that is composed of multiple simple shapes, such as triangles, quadrilaterals, polygons, and circles.
How do I calculate the area of a compound shape?
+There are several methods to calculate the area of a compound shape, including decomposition, addition, subtraction, tessellation, grid, sector, and coordinate geometry methods.
Which method is the most accurate?
+The most accurate method depends on the specific shape and its properties. However, the coordinate geometry method is generally considered to be the most accurate method for complex shapes.