7 Ways to Solve Composite Figures Area Problems

Understanding Composite Figures and Their Areas

Composite figures are geometric shapes that are made up of two or more simpler shapes. These shapes can be triangles, quadrilaterals, polygons, circles, or a combination of these. Finding the area of composite figures can be a bit challenging, but with the right approach, it can be done easily. In this article, we will explore 7 ways to solve composite figures area problems.

What are Composite Figures?

Composite figures are shapes that are composed of two or more simpler shapes. These shapes can be put together to form a new shape, and the area of the new shape is the sum of the areas of the individual shapes.

Why are Composite Figures Important?

Composite figures are important in mathematics and real-life applications. They are used to solve problems involving perimeter, area, and volume of complex shapes. Composite figures are also used in architecture, engineering, and design to create complex shapes and structures.

7 Ways to Solve Composite Figures Area Problems

Here are 7 ways to solve composite figures area problems:

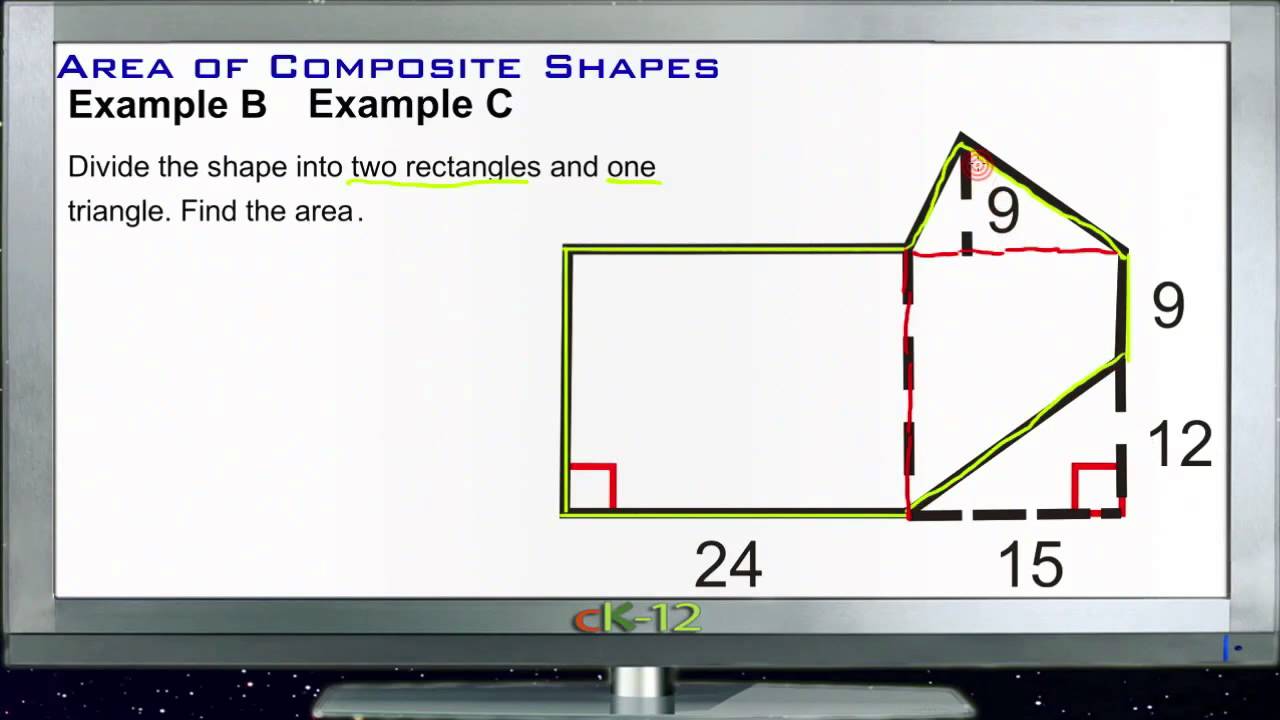
1. Divide the Shape into Simpler Shapes

One way to solve composite figures area problems is to divide the shape into simpler shapes. For example, if you have a shape that is made up of a rectangle and a triangle, you can divide the shape into two separate shapes and find the area of each shape separately.

Example:

Find the area of the composite shape below:

Solution:

Divide the shape into a rectangle and a triangle.

Area of rectangle = length x width = 4 x 5 = 20 Area of triangle = (base x height) / 2 = (3 x 4) / 2 = 6

Total area = area of rectangle + area of triangle = 20 + 6 = 26

2. Use the Formula for the Area of Each Shape

Another way to solve composite figures area problems is to use the formula for the area of each shape. For example, if you have a shape that is made up of a circle and a rectangle, you can use the formula for the area of a circle (A = πr^2) and the formula for the area of a rectangle (A = length x width).

Example:

Find the area of the composite shape below:

Solution:

Area of circle = πr^2 = π(4)^2 = 16π Area of rectangle = length x width = 4 x 5 = 20

Total area = area of circle + area of rectangle = 16π + 20

3. Use Decomposition to Find the Area

Decomposition is a method of breaking down a complex shape into simpler shapes. This method can be used to find the area of composite figures.

Example:

Find the area of the composite shape below:

Solution:

Decompose the shape into two triangles and a rectangle.

Area of triangle 1 = (base x height) / 2 = (3 x 4) / 2 = 6 Area of triangle 2 = (base x height) / 2 = (3 x 4) / 2 = 6 Area of rectangle = length x width = 4 x 5 = 20

Total area = area of triangle 1 + area of triangle 2 + area of rectangle = 6 + 6 + 20 = 32

4. Use the Overlap Method

The overlap method is a technique used to find the area of composite figures by finding the area of the overlap between two shapes.

Example:

Find the area of the composite shape below:

Solution:

Find the area of the overlap between the two triangles.

Area of overlap = (base x height) / 2 = (3 x 4) / 2 = 6

Total area = area of triangle 1 + area of triangle 2 - area of overlap = 12 + 12 - 6 = 18

5. Use the Congruent Parts Method

The congruent parts method is a technique used to find the area of composite figures by finding the area of congruent parts.

Example:

Find the area of the composite shape below:

Solution:

Find the area of the congruent parts.

Area of part 1 = (base x height) / 2 = (3 x 4) / 2 = 6 Area of part 2 = (base x height) / 2 = (3 x 4) / 2 = 6

Total area = area of part 1 + area of part 2 = 6 + 6 = 12

6. Use the Grid Method

The grid method is a technique used to find the area of composite figures by creating a grid and counting the number of squares.

Example:

Find the area of the composite shape below:

Solution:

Create a grid and count the number of squares.

Total area = number of squares = 12

7. Use Technology to Find the Area

Technology can be used to find the area of composite figures. Graphing calculators and computer software can be used to find the area of complex shapes.

Example:

Find the area of the composite shape below using a graphing calculator:

Solution:

Use a graphing calculator to find the area of the composite shape.

Total area = 25.13

📝 Note: The above examples are just a few ways to solve composite figures area problems. The method used will depend on the complexity of the shape and the individual's preference.

In conclusion, finding the area of composite figures can be done using various methods. By understanding the different techniques and methods, individuals can choose the best approach to solve the problem. Whether it’s dividing the shape into simpler shapes, using the formula for the area of each shape, or using technology, the key is to find the method that works best for the individual.