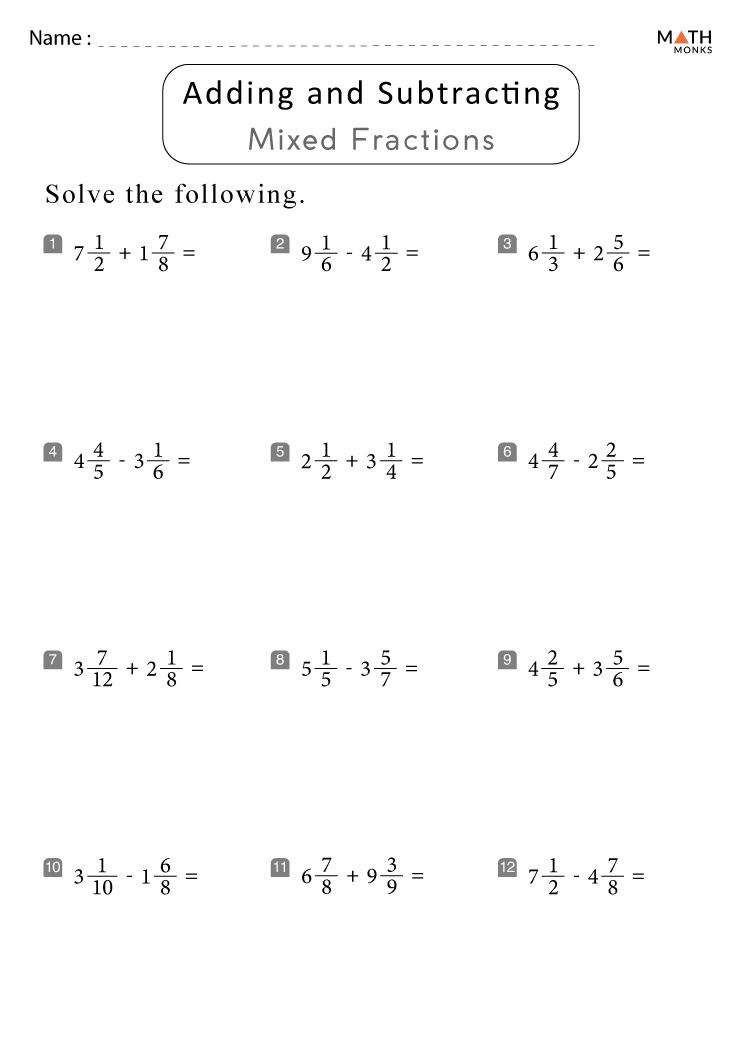5 Ways to Master Composite Figures Area Calculations
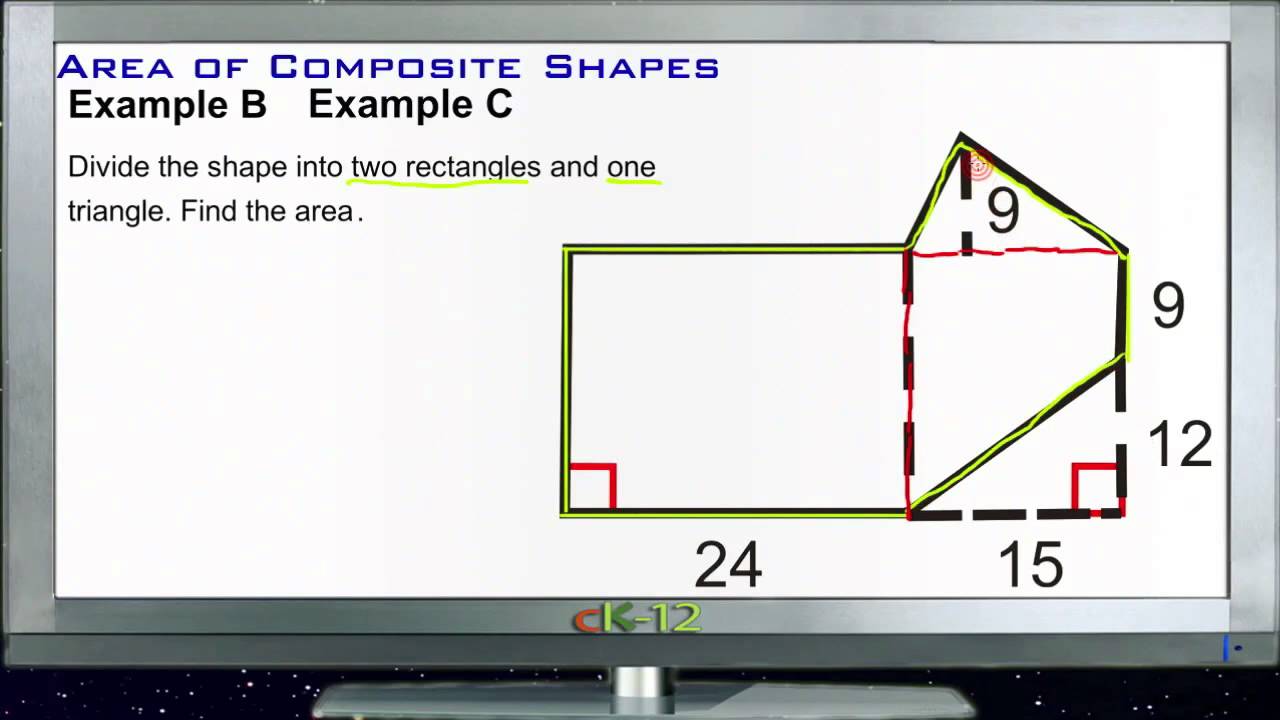
Understanding Composite Figures
Composite figures are geometric shapes that are composed of two or more simple shapes, such as triangles, rectangles, and circles. Calculating the area of these figures can be challenging, but with the right strategies, you can master them. In this article, we will explore five ways to calculate the area of composite figures.
Method 1: Break Down the Figure into Simple Shapes
One of the most effective ways to calculate the area of a composite figure is to break it down into simple shapes. This method involves identifying the individual shapes that make up the composite figure and calculating their areas separately.
For example, consider a composite figure composed of a rectangle and a triangle.
| Shape | Area Formula | Dimensions | Area |
| Rectangle | Length x Width | 4cm x 3cm | 12cm² |
| Triangle | 1/2 x Base x Height | 4cm x 2cm | 4cm² |
| Total Area | 16cm² |
By breaking down the composite figure into simple shapes, we can calculate the total area by adding the areas of the individual shapes.
📝 Note: Make sure to label each shape and its dimensions clearly to avoid confusion.
Method 2: Use the Shaded Region Method
Another method to calculate the area of a composite figure is to use the shaded region method. This method involves shading the region of the composite figure that you want to calculate the area of and then finding the area of the shaded region.
For example, consider a composite figure composed of a circle and a rectangle.

To calculate the area of the shaded region, we can use the formula:
Area of shaded region = Area of circle - Area of unshaded region
Area of shaded region = πr² - (Length x Width)
where r is the radius of the circle and Length and Width are the dimensions of the rectangle.
📝 Note: Make sure to identify the radius of the circle and the dimensions of the rectangle correctly.
Method 3: Use the Sector Method
The sector method is a useful technique for calculating the area of a composite figure that involves a circle and a triangle. This method involves finding the area of the sector of the circle that is bounded by the triangle.
For example, consider a composite figure composed of a circle and a triangle.

To calculate the area of the sector, we can use the formula:
Area of sector = (θ/360) x πr²
where θ is the central angle of the sector and r is the radius of the circle.
📝 Note: Make sure to identify the central angle and the radius of the circle correctly.
Method 4: Use the Overlapping Shapes Method
The overlapping shapes method is a useful technique for calculating the area of a composite figure that involves two or more overlapping shapes. This method involves finding the area of each shape separately and then subtracting the area of the overlapping region.
For example, consider a composite figure composed of two overlapping rectangles.

To calculate the area of the composite figure, we can use the formula:
Area of composite figure = Area of Shape 1 + Area of Shape 2 - Area of overlapping region
Area of composite figure = (Length x Width) + (Length x Width) - (Length x Width)
where Length and Width are the dimensions of the rectangles.
📝 Note: Make sure to identify the dimensions of the rectangles and the overlapping region correctly.
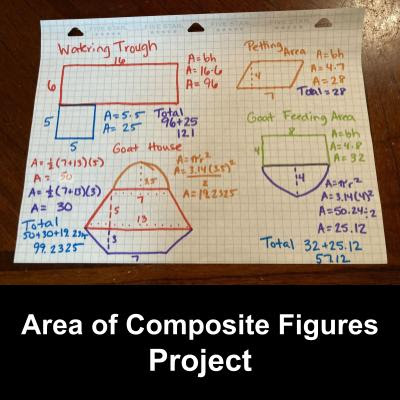
Method 5: Use the Grid Method
The grid method is a useful technique for calculating the area of a composite figure that involves a grid of squares. This method involves counting the number of squares that make up the composite figure and multiplying it by the area of each square.
For example, consider a composite figure composed of a grid of squares.

To calculate the area of the composite figure, we can use the formula:
Area of composite figure = Number of squares x Area of each square
Area of composite figure = 10 x 1cm²
where the number of squares is 10 and the area of each square is 1cm².
📝 Note: Make sure to count the number of squares correctly and multiply it by the area of each square correctly.
In summary, calculating the area of composite figures can be challenging, but with the right strategies, you can master them. The five methods discussed in this article are:
- Breaking down the figure into simple shapes
- Using the shaded region method
- Using the sector method
- Using the overlapping shapes method
- Using the grid method
By practicing these methods, you can become proficient in calculating the area of composite figures.
In conclusion, mastering composite figures area calculations requires practice, patience, and persistence. By using the methods discussed in this article, you can improve your skills and become more confident in calculating the area of complex geometric shapes.
What is a composite figure?
+A composite figure is a geometric shape that is composed of two or more simple shapes, such as triangles, rectangles, and circles.
How do I calculate the area of a composite figure?
+There are several methods to calculate the area of a composite figure, including breaking down the figure into simple shapes, using the shaded region method, using the sector method, using the overlapping shapes method, and using the grid method.
What is the shaded region method?
+The shaded region method involves shading the region of the composite figure that you want to calculate the area of and then finding the area of the shaded region.