Arc Length and Sector Area Calculations Made Easy
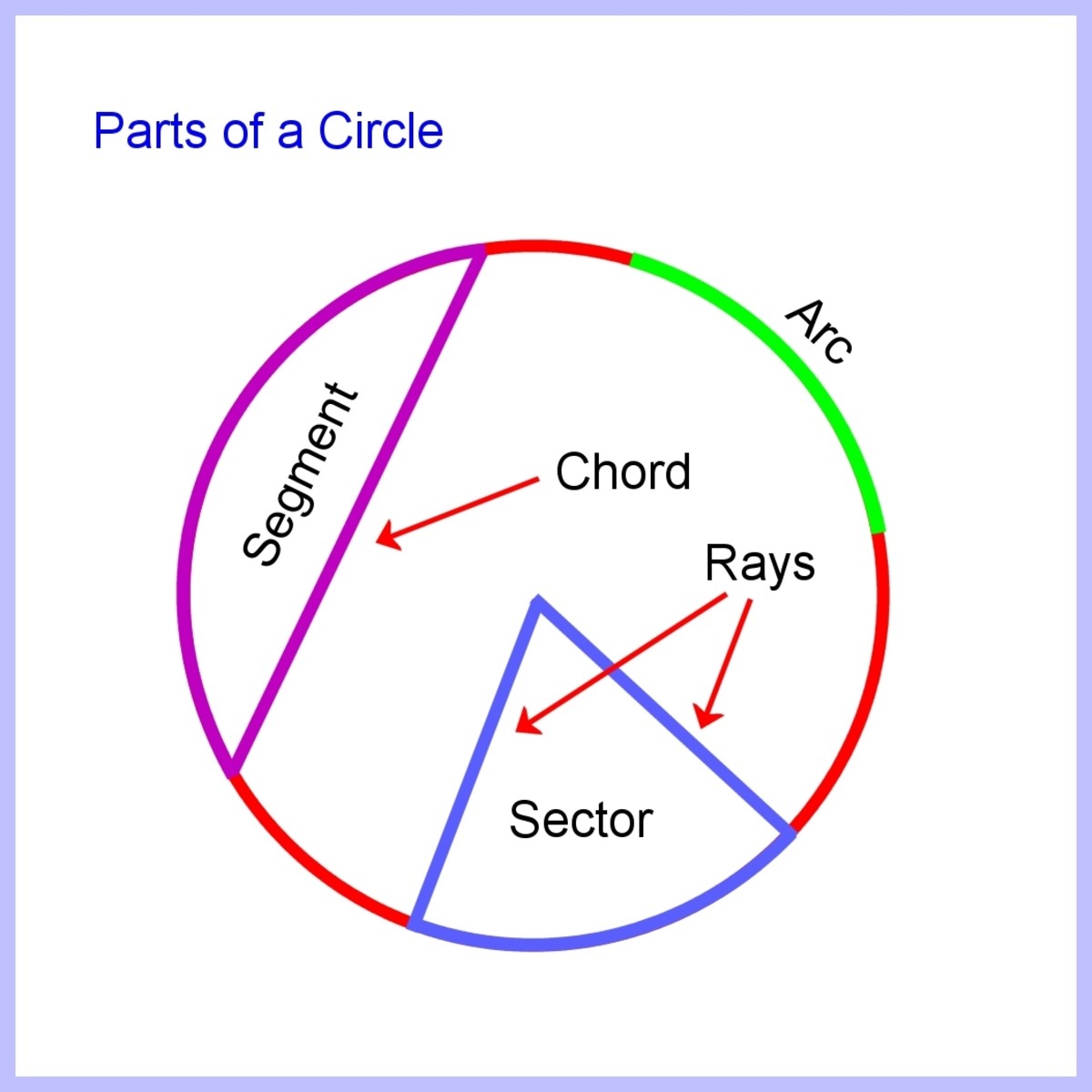
Understanding Arc Length and Sector Area
Calculating arc length and sector area can be a daunting task for many students and professionals alike. However, with the right approach and formulas, these calculations can be made easy and straightforward. In this article, we will explore the concepts of arc length and sector area, discuss the formulas used to calculate them, and provide examples to illustrate the calculations.
Arc Length Calculation
The arc length is the distance along a curve or arc. It is a fundamental concept in mathematics and is used in various fields such as engineering, physics, and architecture. To calculate the arc length, we use the following formula:
Arc Length Formula:
L = θ × r
Where:
- L is the arc length
- θ is the central angle in radians
- r is the radius of the circle
For example, let’s calculate the arc length of a circle with a radius of 4 cm and a central angle of 60 degrees.
📝 Note: To convert degrees to radians, multiply the angle by π/180.
θ = 60 × π/180 = π/3 radians
L = θ × r = (π/3) × 4 = 4π/3 cm
Sector Area Calculation
The sector area is the area of a sector of a circle. It is calculated using the following formula:
Sector Area Formula:
A = (θ/360) × πr^2
Where:
- A is the sector area
- θ is the central angle in degrees
- r is the radius of the circle
For example, let’s calculate the sector area of a circle with a radius of 5 cm and a central angle of 120 degrees.
A = (120⁄360) × π(5)^2 = (1⁄3) × 25π = 25π/3 cm^2
Table of Formulas
Here is a summary of the formulas used to calculate arc length and sector area:
| Formula | Description |
|---|---|
| L = θ × r | Arc Length Formula |
| A = (θ/360) × πr^2 | Sector Area Formula |
Real-World Applications
Arc length and sector area calculations have numerous real-world applications in various fields. Here are a few examples:
- Architecture: Arc length calculations are used to design arches, bridges, and other curved structures.
- Engineering: Sector area calculations are used to design circular tanks, pipes, and other cylindrical structures.
- Physics: Arc length calculations are used to calculate the distance traveled by an object along a curved path.
Conclusion
In conclusion, calculating arc length and sector area can be made easy and straightforward by using the right formulas and approach. By understanding the concepts and formulas, you can apply them to real-world problems and make accurate calculations.
What is the difference between arc length and sector area?
+Arc length is the distance along a curve or arc, while sector area is the area of a sector of a circle.
How do I convert degrees to radians?
+To convert degrees to radians, multiply the angle by π/180.
What is the formula for calculating sector area?
+The formula for calculating sector area is A = (θ/360) × πr^2.