Slope Intercept Form Worksheet Answers
Understanding Slope Intercept Form: A Comprehensive Guide
Slope intercept form is a fundamental concept in algebra and geometry, used to represent linear equations in a concise and intuitive way. In this article, we will delve into the world of slope intercept form, exploring its definition, applications, and providing a worksheet with answers to help you master this essential math concept.
What is Slope Intercept Form?
Slope intercept form is a way of representing linear equations in the form y = mx + b, where:
- m is the slope of the line (a measure of how steep it is)
- b is the y-intercept (the point where the line crosses the y-axis)
- x is the independent variable (the input or x-coordinate)
- y is the dependent variable (the output or y-coordinate)
The slope intercept form is useful because it allows us to easily identify the slope and y-intercept of a line, which can be used to graph the line, find the equation of a line, and solve problems involving linear relationships.
How to Graph a Line in Slope Intercept Form
To graph a line in slope intercept form, follow these steps:
- Plot the y-intercept (b) on the y-axis.
- Use the slope (m) to determine the direction and steepness of the line.
- Plot a second point on the line by moving horizontally (right or left) by one unit and vertically (up or down) by the slope.
- Draw a straight line through the two points.
Slope Intercept Form Worksheet
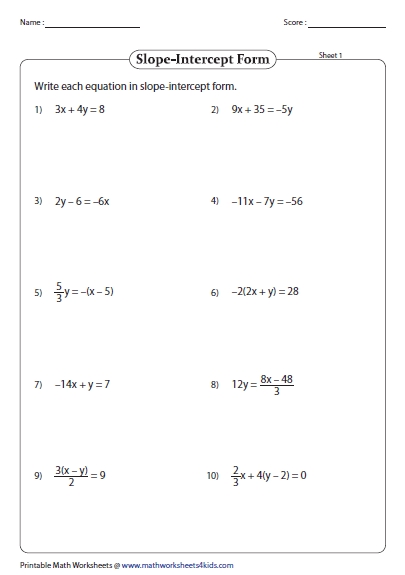
Now, it’s time to practice! Here’s a worksheet with 10 questions to help you master slope intercept form.
| Question | Equation | Slope (m) | Y-Intercept (b) |
|---|---|---|---|
| 1 | y = 2x + 3 | 2 | 3 |
| 2 | y = -4x - 2 | -4 | -2 |
| 3 | y = x + 1 | 1 | 1 |
| 4 | y = -2x + 5 | -2 | 5 |
| 5 | y = 3x - 4 | 3 | -4 |
| 6 | y = -x - 3 | -1 | -3 |
| 7 | y = 2x - 1 | 2 | -1 |
| 8 | y = x + 2 | 1 | 2 |
| 9 | y = -3x + 1 | -3 | 1 |
| 10 | y = 4x - 2 | 4 | -2 |
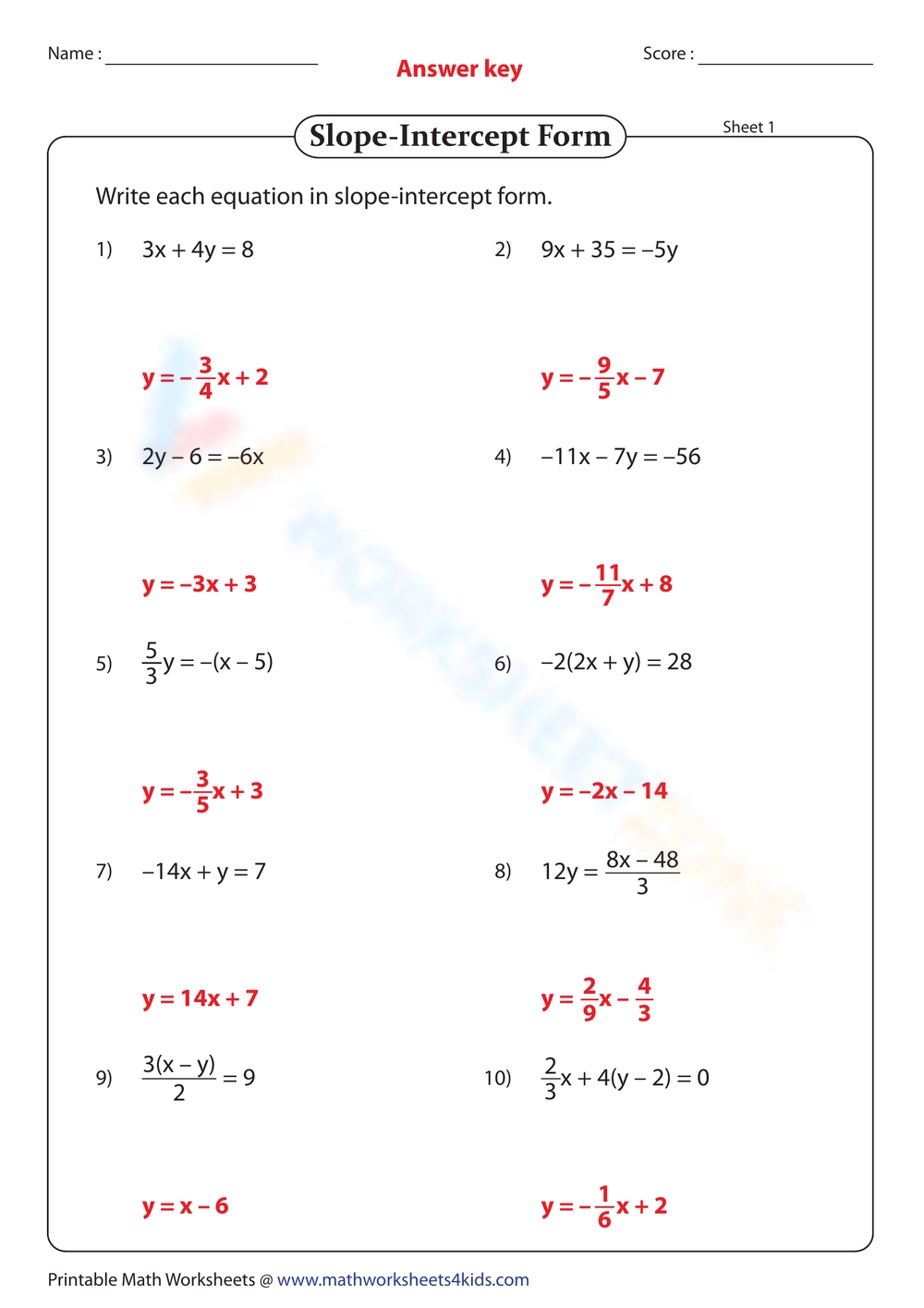
Answers
Here are the answers to the worksheet:
- m = 2, b = 3
- m = -4, b = -2
- m = 1, b = 1
- m = -2, b = 5
- m = 3, b = -4
- m = -1, b = -3
- m = 2, b = -1
- m = 1, b = 2
- m = -3, b = 1
- m = 4, b = -2
📝 Note: Remember to identify the slope (m) and y-intercept (b) for each equation to graph the line correctly.
Common Applications of Slope Intercept Form
Slope intercept form has numerous applications in various fields, including:
- Physics: to model the motion of objects
- Engineering: to design and optimize systems
- Economics: to analyze supply and demand curves
- Computer Science: to create algorithms for graphing and data analysis
Real-World Examples of Slope Intercept Form
- Cost Analysis: A company has a fixed cost of 500 and a variable cost of 10 per unit produced. The total cost © can be represented by the equation C = 10x + 500, where x is the number of units produced.
- Motion Analysis: A car is traveling at a constant speed of 60 miles per hour. The distance (d) traveled can be represented by the equation d = 60t + 0, where t is the time in hours.
Conclusion
Slope intercept form is a powerful tool for representing linear equations and analyzing relationships between variables. By mastering slope intercept form, you’ll be able to graph lines, find equations, and solve problems in a wide range of fields. Remember to identify the slope and y-intercept for each equation, and practice applying slope intercept form to real-world examples.
What is the slope intercept form of a linear equation?
+The slope intercept form of a linear equation is y = mx + b, where m is the slope and b is the y-intercept.
How do I graph a line in slope intercept form?
+To graph a line in slope intercept form, plot the y-intercept (b) on the y-axis, use the slope (m) to determine the direction and steepness of the line, and plot a second point on the line by moving horizontally (right or left) by one unit and vertically (up or down) by the slope.
What are some real-world applications of slope intercept form?
+Slope intercept form has numerous applications in physics, engineering, economics, and computer science, including cost analysis, motion analysis, and data analysis.