Algebra 2 Answer Key: Graphing Quadratic Functions Made Easy

Unlocking the Secrets of Quadratic Functions

Quadratic functions are a fundamental part of algebra, and graphing them can seem intimidating at first. However, with the right approach, it can be made easy. In this post, we’ll explore the world of quadratic functions, and by the end of it, you’ll be able to graph them with confidence.

What are Quadratic Functions?

Quadratic functions are polynomial functions of degree two, which means the highest power of the variable (usually x) is two. They have the general form:

f(x) = ax^2 + bx + c

where a, b, and c are constants, and a cannot be zero.

The Anatomy of a Quadratic Function

A quadratic function has several key components that help us graph it:

• Vertex: The vertex is the lowest or highest point on the graph, depending on whether the parabola opens upwards or downwards. It’s also the point where the axis of symmetry intersects the graph.
• Axis of Symmetry: This is the vertical line that passes through the vertex and divides the graph into two symmetrical halves.
• X-Intercepts: These are the points where the graph crosses the x-axis. They can be found by setting f(x) = 0 and solving for x.
• Y-Intercept: This is the point where the graph crosses the y-axis. It can be found by evaluating f(0).

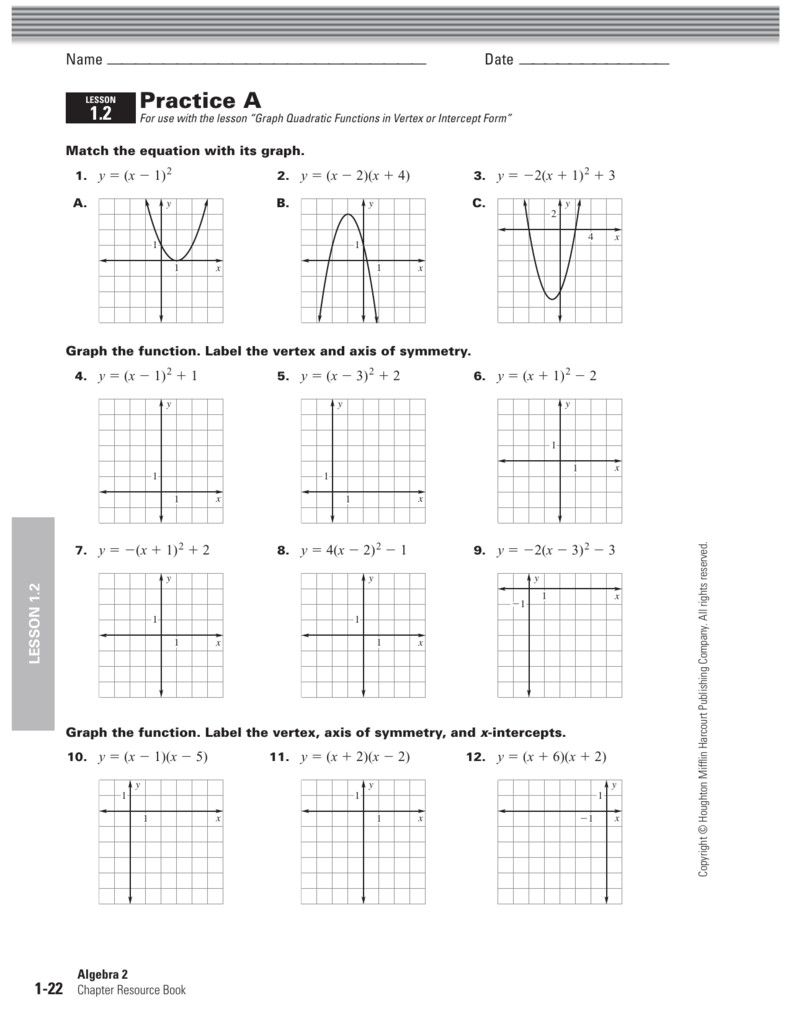
How to Graph a Quadratic Function

Now that we know the anatomy of a quadratic function, let’s learn how to graph it. Here are the steps:

1. Find the Vertex: The vertex can be found using the formula x = -b/2a. Plug this value back into the equation to find the corresponding y-coordinate.
2. Find the Axis of Symmetry: The axis of symmetry is the vertical line that passes through the vertex. Its equation is x = h, where h is the x-coordinate of the vertex.
3. Find the X-Intercepts: Set f(x) = 0 and solve for x. This will give you the x-coordinates of the x-intercepts.
4. Find the Y-Intercept: Evaluate f(0) to find the y-coordinate of the y-intercept.
5. Plot the Points: Plot the vertex, x-intercepts, and y-intercept on the coordinate plane.
6. Draw the Graph: Use the points you’ve plotted to draw a smooth curve. Make sure it’s symmetrical about the axis of symmetry.

Example Problem

Let’s graph the quadratic function f(x) = x^2 + 4x + 4.

1. Find the Vertex: x = -b/2a = -⁴⁄₂(1) = -2 y = f(-2) = (-2)^2 + 4(-2) + 4 = 4 - 8 + 4 = 0 The vertex is (-2, 0).
2. Find the Axis of Symmetry: x = h = -2
3. Find the X-Intercepts: f(x) = 0 –> x^2 + 4x + 4 = 0 –> (x + 2)^2 = 0 –> x + 2 = 0 –> x = -2 There’s only one x-intercept, which is (-2, 0).
4. Find the Y-Intercept: f(0) = 0^2 + 4(0) + 4 = 4 The y-intercept is (0, 4).
5. Plot the Points: Plot the vertex (-2, 0), x-intercept (-2, 0), and y-intercept (0, 4).
6. Draw the Graph: Draw a smooth curve that passes through the points. Make sure it’s symmetrical about the axis of symmetry x = -2.

📝 Note: The graph of f(x) = x^2 + 4x + 4 is a parabola that opens upwards, and its vertex is the lowest point on the graph.

Common Mistakes to Avoid

When graphing quadratic functions, here are some common mistakes to avoid:

• Forgetting to find the vertex: The vertex is a crucial point on the graph, and forgetting to find it can lead to an incorrect graph.
• Not finding the axis of symmetry: The axis of symmetry helps you draw a symmetrical graph, so make sure to find it.
• Not plotting the points correctly: Plotting the points correctly is crucial in drawing an accurate graph.

By following these steps and avoiding common mistakes, you’ll be able to graph quadratic functions with ease.

Graphing quadratic functions is a fundamental skill in algebra, and with practice, you’ll become proficient in no time. Remember to find the vertex, axis of symmetry, x-intercepts, and y-intercept, and plot the points correctly. With these steps, you’ll be able to graph any quadratic function that comes your way.