5 Ways to Solve Angles of Depression and Elevation
Understanding Angles of Depression and Elevation
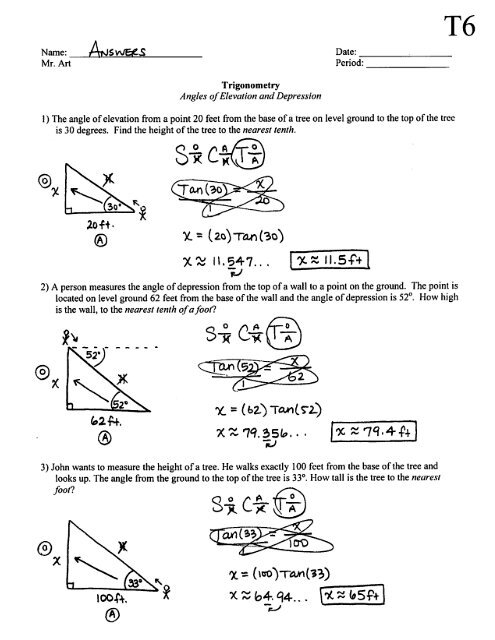
In trigonometry and geometry, angles of depression and elevation are used to describe the position of an object in relation to the horizontal plane. The angle of depression is the angle between the horizontal plane and the line of sight to an object below the horizontal plane, while the angle of elevation is the angle between the horizontal plane and the line of sight to an object above the horizontal plane. Solving angles of depression and elevation is essential in various fields, including physics, engineering, and surveying. Here are five ways to solve angles of depression and elevation.
Method 1: Using Trigonometric Ratios
Trigonometric ratios can be used to solve angles of depression and elevation. The tangent, sine, and cosine ratios can be used to relate the angle of depression or elevation to the opposite side, adjacent side, and hypotenuse of a right triangle.
- Tangent Ratio: The tangent ratio is the ratio of the opposite side to the adjacent side. This ratio can be used to find the angle of depression or elevation.
- Sine Ratio: The sine ratio is the ratio of the opposite side to the hypotenuse. This ratio can be used to find the angle of depression or elevation.
- Cosine Ratio: The cosine ratio is the ratio of the adjacent side to the hypotenuse. This ratio can be used to find the angle of depression or elevation.
Using these ratios, you can solve angles of depression and elevation by plugging in the values of the opposite side, adjacent side, and hypotenuse.
Method 2: Using the Angle of Depression Formula
The angle of depression formula can be used to solve angles of depression. The formula is:
θ = arctan (opposite side / adjacent side)
where θ is the angle of depression, and opposite side and adjacent side are the lengths of the opposite side and adjacent side, respectively.
Similarly, the angle of elevation formula can be used to solve angles of elevation. The formula is:
θ = arctan (opposite side / adjacent side)
where θ is the angle of elevation, and opposite side and adjacent side are the lengths of the opposite side and adjacent side, respectively.
Method 3: Using the Angle of Elevation Formula with the Distance and Height
If the distance and height of the object are known, the angle of elevation can be solved using the following formula:
θ = arctan (height / distance)
where θ is the angle of elevation, height is the height of the object, and distance is the distance from the observer to the object.
Similarly, if the distance and depth of the object are known, the angle of depression can be solved using the following formula:
θ = arctan (depth / distance)
where θ is the angle of depression, depth is the depth of the object, and distance is the distance from the observer to the object.
Method 4: Using a Calculator or Trigonometry Table
A calculator or trigonometry table can be used to solve angles of depression and elevation. Most calculators have a built-in trigonometric function that can be used to find the angle of depression or elevation.
Trigonometry tables can also be used to solve angles of depression and elevation. These tables provide the values of the sine, cosine, and tangent ratios for different angles.
Method 5: Using Geometry and Similar Triangles
Geometry and similar triangles can be used to solve angles of depression and elevation. By drawing a diagram of the situation, you can use similar triangles to relate the angle of depression or elevation to other known angles.
For example, if two triangles are similar, the corresponding angles are equal. This property can be used to solve angles of depression and elevation.
[📝] Note: When solving angles of depression and elevation, it is essential to ensure that the units of measurement are consistent. Inconsistent units can lead to incorrect solutions.
To summarize, there are five ways to solve angles of depression and elevation: using trigonometric ratios, the angle of depression formula, the angle of elevation formula with the distance and height, a calculator or trigonometry table, and geometry and similar triangles. By mastering these methods, you can solve angles of depression and elevation with confidence.
Now, we will look at some Frequently Asked Questions (FAQs) about angles of depression and elevation.
What is the difference between an angle of depression and an angle of elevation?
+The angle of depression is the angle between the horizontal plane and the line of sight to an object below the horizontal plane, while the angle of elevation is the angle between the horizontal plane and the line of sight to an object above the horizontal plane.
How do I solve an angle of depression or elevation using trigonometric ratios?
+Use the tangent, sine, or cosine ratio to relate the angle of depression or elevation to the opposite side, adjacent side, and hypotenuse of a right triangle. For example, the tangent ratio is the ratio of the opposite side to the adjacent side.
What is the formula for the angle of depression?
+The formula for the angle of depression is θ = arctan (opposite side / adjacent side), where θ is the angle of depression, and opposite side and adjacent side are the lengths of the opposite side and adjacent side, respectively.
How do I use a calculator to solve an angle of depression or elevation?
+Most calculators have a built-in trigonometric function that can be used to find the angle of depression or elevation. Simply enter the values of the opposite side, adjacent side, and hypotenuse, and the calculator will provide the solution.
What are some real-world applications of angles of depression and elevation?
+Angles of depression and elevation have numerous real-world applications in fields such as physics, engineering, surveying, and navigation. For example, they are used to determine the height of buildings, the distance to ships at sea, and the elevation of mountains.