Angles in a Circle Worksheet With Answers
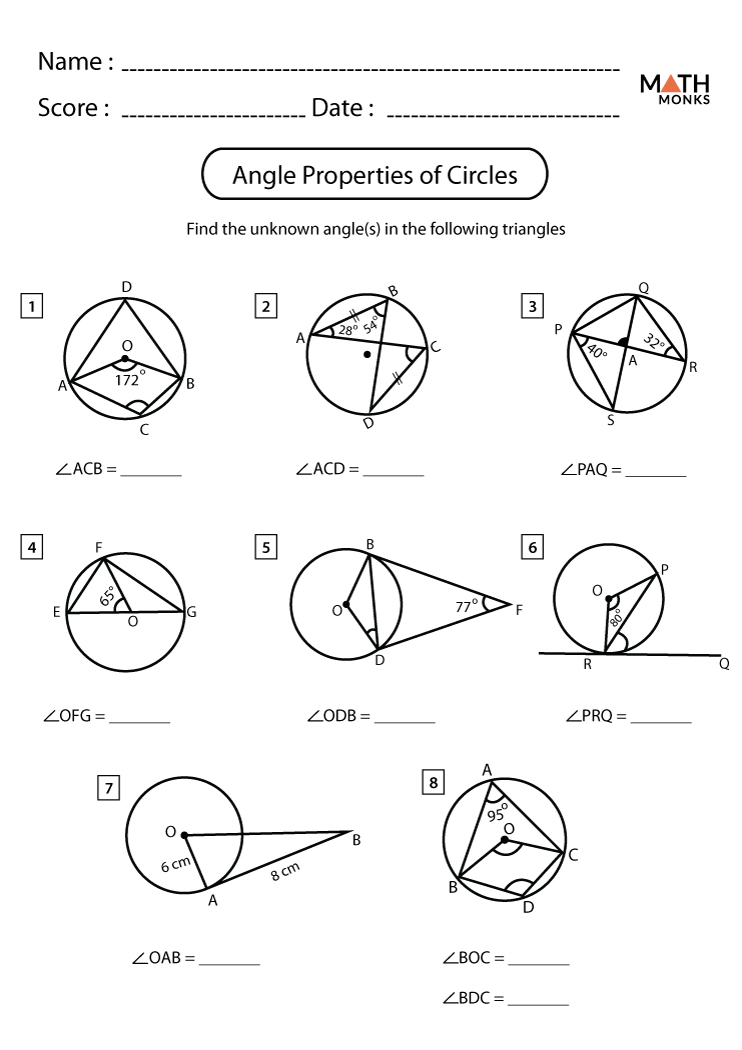
Understanding Angles in a Circle
Angles in a circle are a fundamental concept in geometry, and mastering them is essential for solving various problems in mathematics. In this worksheet, we will explore the different types of angles that can be formed within a circle and provide answers to help you assess your understanding.
Types of Angles in a Circle
There are several types of angles that can be formed within a circle, including:
- Central angles: These are angles formed by two radii of a circle.
- Inscribed angles: These are angles formed by two chords of a circle that intersect on the circle.
- Circumscribed angles: These are angles formed by two chords of a circle that intersect outside the circle.
- Intersecting angles: These are angles formed by two chords of a circle that intersect inside the circle.
Properties of Angles in a Circle
Angles in a circle have several important properties, including:
- Inscribed angle theorem: The measure of an inscribed angle is equal to half the measure of its intercepted arc.
- Central angle theorem: The measure of a central angle is equal to the measure of its intercepted arc.
- Circumscribed angle theorem: The measure of a circumscribed angle is equal to half the measure of its intercepted arc.
Worksheet
Complete the following problems to test your understanding of angles in a circle:
- In the diagram below, angle A is an inscribed angle that intercepts an arc of 120 degrees. What is the measure of angle A?
Answer: 60 degrees
- In the diagram below, angle B is a central angle that intercepts an arc of 150 degrees. What is the measure of angle B?
Answer: 150 degrees
- In the diagram below, angle C is a circumscribed angle that intercepts an arc of 90 degrees. What is the measure of angle C?
Answer: 45 degrees
- In the diagram below, angles D and E are intersecting angles that intercept an arc of 60 degrees. What is the measure of angle D and angle E?
Answer: Angle D = 30 degrees, Angle E = 30 degrees
- In the diagram below, angle F is an inscribed angle that intercepts an arc of 180 degrees. What is the measure of angle F?
Answer: 90 degrees
📝 Note: When solving problems involving angles in a circle, it's essential to identify the type of angle and apply the relevant theorem to find the measure of the angle.
Answers and Explanations
Since angle A is an inscribed angle, we can apply the inscribed angle theorem to find its measure. The measure of angle A is equal to half the measure of its intercepted arc, which is 120 degrees. Therefore, the measure of angle A is 60 degrees.
Since angle B is a central angle, we can apply the central angle theorem to find its measure. The measure of angle B is equal to the measure of its intercepted arc, which is 150 degrees. Therefore, the measure of angle B is 150 degrees.
Since angle C is a circumscribed angle, we can apply the circumscribed angle theorem to find its measure. The measure of angle C is equal to half the measure of its intercepted arc, which is 90 degrees. Therefore, the measure of angle C is 45 degrees.
Since angles D and E are intersecting angles, we can apply the intersecting angle theorem to find their measures. The measures of angles D and E are equal to half the measure of their intercepted arc, which is 60 degrees. Therefore, the measures of angles D and E are 30 degrees each.
Since angle F is an inscribed angle, we can apply the inscribed angle theorem to find its measure. The measure of angle F is equal to half the measure of its intercepted arc, which is 180 degrees. Therefore, the measure of angle F is 90 degrees.
Conclusion
Angles in a circle are a fundamental concept in geometry, and understanding their properties and theorems is essential for solving various problems in mathematics. By mastering the inscribed angle theorem, central angle theorem, and circumscribed angle theorem, you can easily find the measure of angles in a circle.
What is the inscribed angle theorem?
+The inscribed angle theorem states that the measure of an inscribed angle is equal to half the measure of its intercepted arc.
What is the central angle theorem?
+The central angle theorem states that the measure of a central angle is equal to the measure of its intercepted arc.
What is the circumscribed angle theorem?
+The circumscribed angle theorem states that the measure of a circumscribed angle is equal to half the measure of its intercepted arc.
Related Terms:
- Angles in Circles Worksheet pdf
- Circles Review Worksheet pdf
- Angles in circles practice
- Circumscribed angles Worksheet pdf
- Angles in a circle pdf