Mastering Algebraic Expressions Worksheet for Students

Mastering Algebraic Expressions Worksheet for Students

Algebraic expressions are a fundamental concept in mathematics, and mastering them is crucial for students to succeed in various mathematical disciplines. This worksheet is designed to help students practice and reinforce their understanding of algebraic expressions, which are a crucial building block for more advanced mathematical concepts.

What are Algebraic Expressions?

Algebraic expressions are mathematical statements that contain variables, constants, and mathematical operations. They are used to represent relationships between variables and constants, and they can be simplified, manipulated, and evaluated to solve equations and inequalities.

Types of Algebraic Expressions

There are several types of algebraic expressions, including:

• Monomials: Expressions that contain only one term, such as 2x or 3y.
• Binomials: Expressions that contain two terms, such as x + 3 or 2x - 4.
• Polynomials: Expressions that contain more than two terms, such as x^2 + 3x - 4 or 2x^3 - 5x^2 + x.
• Rational Expressions: Expressions that contain fractions, such as (x + 1)/(x - 2).

Simplifying Algebraic Expressions

Simplifying algebraic expressions involves combining like terms, factoring, and canceling out any common factors. Here are some examples:

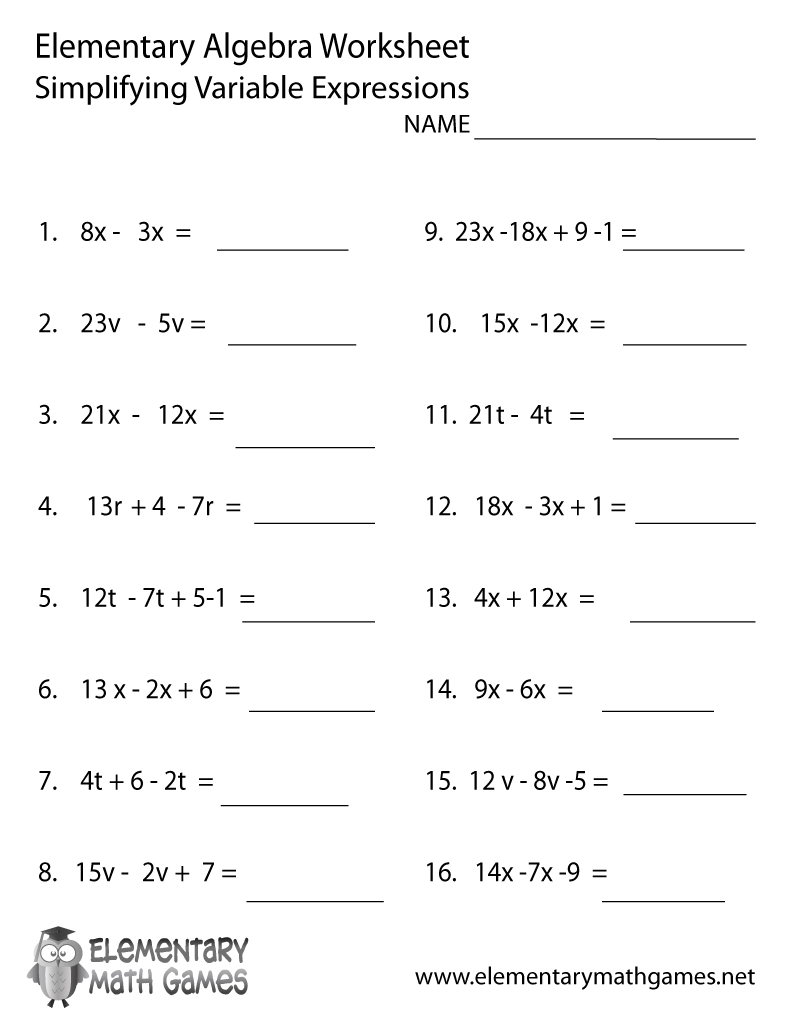
• Combining Like Terms: 2x + 3x = 5x
• Factoring: x^2 + 5x + 6 = (x + 2)(x + 3)
• Canceling Common Factors: (x + 1)(x - 1) = x^2 - 1

Evaluating Algebraic Expressions

Evaluating algebraic expressions involves substituting values for the variables and simplifying the resulting expression. Here are some examples:

• Substituting Values: If x = 2, then 2x + 3 = 2(2) + 3 = 7
• Simplifying the Resulting Expression: If x = 3, then x^2 + 2x - 1 = (3)^2 + 2(3) - 1 = 9 + 6 - 1 = 14

Practicing Algebraic Expressions

Now it’s time to practice! Here are some exercises to help you master algebraic expressions:

Exercise 1: Simplify the following expressions

• 2x + 3x
• x^2 + 4x + 4
• (x + 2)(x - 3)

Exercise 2: Evaluate the following expressions

• If x = 2, then 2x + 3
• If x = 4, then x^2 - 2x - 1
• If x = 1, then (x + 1)(x - 2)

Exercise 3: Solve the following equations

• 2x + 3 = 7
• x^2 + 2x - 3 = 0
• (x + 1)(x - 2) = 0

📝 Note: Make sure to show your work and simplify your answers. Use a calculator only if necessary.

Common Mistakes to Avoid

When working with algebraic expressions, it’s easy to make mistakes. Here are some common mistakes to avoid:

• Incorrectly combining like terms: Make sure to combine only terms with the same variable and coefficient.
• Forgetting to distribute: When multiplying expressions, make sure to distribute the terms correctly.
• Canceling out terms incorrectly: Make sure to cancel out only common factors, not just any terms.

Conclusion

Mastering algebraic expressions is a fundamental skill for any math student. By practicing and reinforcing their understanding of these expressions, students can build a strong foundation for more advanced mathematical concepts. Remember to simplify, evaluate, and solve algebraic expressions with care, and don’t be afraid to ask for help when needed.