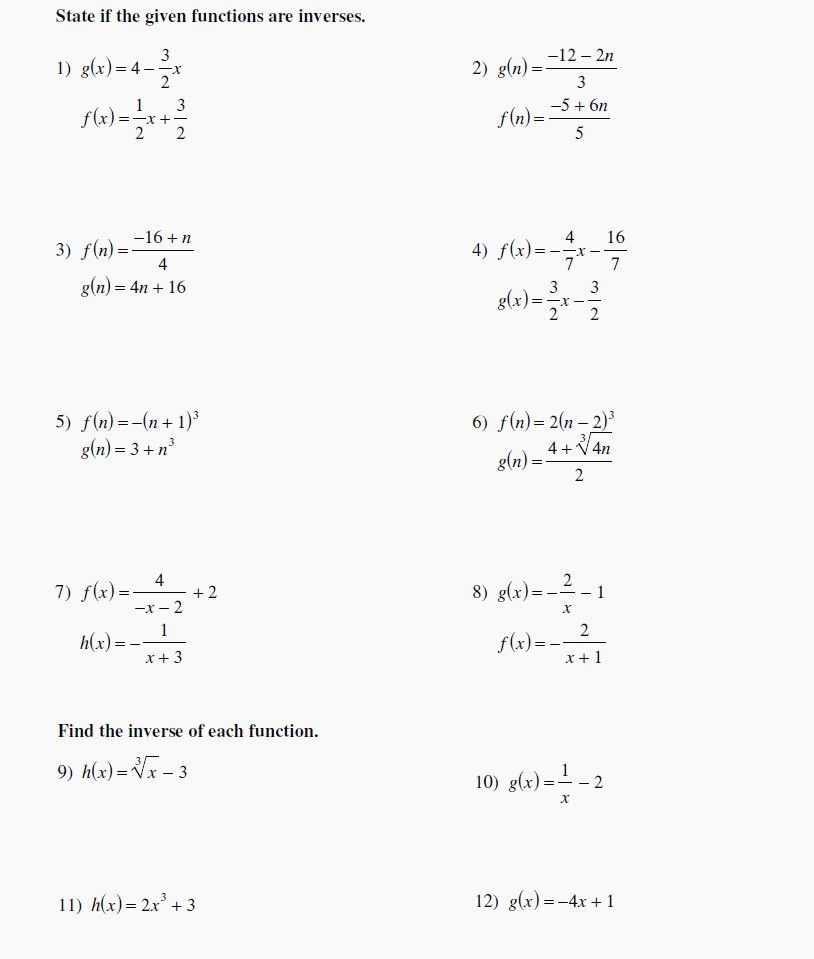
5 Ways to Master Addition of Fractions

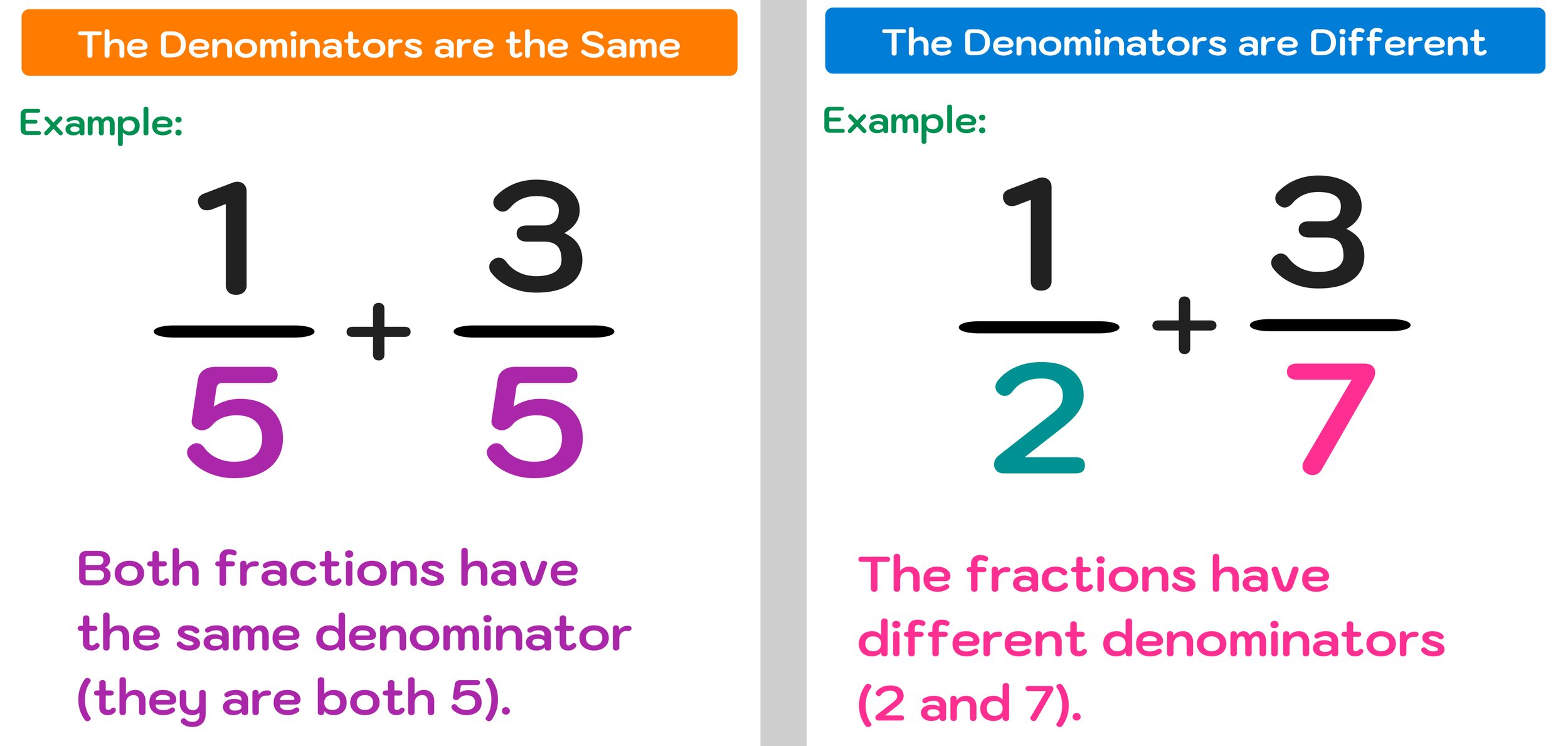
Understanding Fractions and Their Importance

Fractions are a fundamental concept in mathematics, and understanding how to work with them is crucial for building a strong foundation in math. One of the most critical operations involving fractions is addition. Adding fractions may seem daunting at first, but with practice and the right strategies, it can become a breeze. In this article, we will explore five ways to master the addition of fractions.

Method 1: Finding the Least Common Denominator (LCD)

One of the most common methods for adding fractions is to find the least common denominator (LCD). The LCD is the smallest multiple that both denominators share. To find the LCD, follow these steps:

• List the multiples of each denominator
• Identify the smallest multiple that appears in both lists
• Use the LCD as the new denominator for both fractions

For example, let’s add ¹⁄₄ and ¹⁄₆:

• Multiples of 4: 4, 8, 12, 16,…
• Multiples of 6: 6, 12, 18, 24,…
• The LCD is 12, so we can rewrite the fractions as ³⁄₁₂ and ²⁄₁₂
• Add the fractions: ³⁄₁₂ + ²⁄₁₂ = ⁵⁄₁₂

Benefits of Using the LCD Method

Using the LCD method has several benefits:

• It allows for easy addition and subtraction of fractions
• It helps to simplify complex fractions
• It is a versatile method that can be applied to various math operations

🤔 Note: When finding the LCD, make sure to list the multiples of each denominator correctly to avoid errors.

Method 2: Using Equivalent Fractions

Another method for adding fractions is to use equivalent fractions. Equivalent fractions have the same value but different denominators. To add fractions using equivalent fractions, follow these steps:

• Find the equivalent fractions for each fraction
• Add the equivalent fractions

For example, let’s add ¹⁄₂ and ¹⁄₄:

• Equivalent fractions for ¹⁄₂: ²⁄₄, ³⁄₆, ⁴⁄₈,…
• Equivalent fractions for ¹⁄₄: ¹⁄₄, ²⁄₈, ³⁄₁₂,…
• Add the equivalent fractions: ²⁄₄ + ¹⁄₄ = ³⁄₄

Benefits of Using Equivalent Fractions

Using equivalent fractions has several benefits:

• It allows for easy comparison of fractions
• It helps to identify equivalent ratios
• It is a useful method for simplifying complex fractions

📝 Note: When using equivalent fractions, make sure to find the equivalent fractions correctly to avoid errors.

Method 3: Using Visual Aids

Visual aids such as diagrams, charts, and graphs can be a powerful tool for adding fractions. Visual aids help to illustrate the concept of fractions and make it easier to understand the relationship between the numerator and denominator.

For example, let’s add ¹⁄₂ and ¹⁄₄ using a diagram:

• Draw a diagram with two rectangles, one for each fraction
• Shade in the rectangles to represent the fractions
• Count the shaded areas to find the sum

Benefits of Using Visual Aids

Using visual aids has several benefits:

• It helps to illustrate complex concepts
• It makes math more engaging and interactive
• It is a useful tool for visual learners

Method 4: Using Real-World Examples

Using real-world examples is an effective way to make math more meaningful and relevant. Real-world examples help to illustrate the practical application of math concepts and make it easier to understand the importance of fractions.

For example, let’s add ¹⁄₂ and ¹⁄₄ using a real-world example:

• Imagine you have ¹⁄₂ of a pizza and your friend has ¹⁄₄ of a pizza
• Combine the pizzas to find the total amount

Benefits of Using Real-World Examples

Using real-world examples has several benefits:

• It makes math more meaningful and relevant
• It helps to illustrate the practical application of math concepts
• It is a useful tool for making math more engaging and interactive

Method 5: Practicing with Word Problems

Practicing with word problems is an effective way to master the addition of fractions. Word problems help to illustrate the practical application of math concepts and make it easier to understand the importance of fractions.

For example, let’s add ¹⁄₂ and ¹⁄₄ using a word problem:

• Tom has ¹⁄₂ of a bag of apples and his friend has ¹⁄₄ of a bag of apples
• How many bags of apples do they have in total?

Benefits of Practicing with Word Problems

Practicing with word problems has several benefits:

• It helps to illustrate the practical application of math concepts
• It makes math more engaging and interactive
• It is a useful tool for building problem-solving skills

In conclusion, mastering the addition of fractions requires practice, patience, and the right strategies. By using the five methods outlined above, you can build a strong foundation in math and develop a deeper understanding of fractions. Remember to practice regularly, use visual aids, and apply math concepts to real-world examples to become a master of fraction addition.