Mastering Unlike Fractions with Easy Worksheets
Understanding Unlike Fractions
Unlike fractions are fractions that have different denominators. For example, 1⁄2 and 1⁄3 are unlike fractions because they have different denominators (2 and 3, respectively). Mastering unlike fractions is an essential skill for any student, as it is a fundamental concept in mathematics. In this blog post, we will discuss how to work with unlike fractions, including adding, subtracting, multiplying, and dividing them.
Adding Unlike Fractions
Adding unlike fractions requires a common denominator. To find a common denominator, we need to find the least common multiple (LCM) of the two denominators. For example, to add 1⁄2 and 1⁄3, we need to find the LCM of 2 and 3, which is 6.
📝 Note: The LCM is the smallest number that both denominators can divide into evenly.
Once we have the common denominator, we can convert each fraction to have that denominator. In our example, we can convert 1⁄2 to 3⁄6 and 1⁄3 to 2⁄6. Then, we can add the two fractions:
3⁄6 + 2⁄6 = 5⁄6
Subtracting Unlike Fractions
Subtracting unlike fractions is similar to adding them. We need to find a common denominator, convert each fraction to have that denominator, and then subtract the two fractions.
For example, to subtract 1⁄2 from 1⁄3, we need to find the LCM of 2 and 3, which is 6. We can convert 1⁄2 to 3⁄6 and 1⁄3 to 2⁄6. Then, we can subtract the two fractions:
2⁄6 - 3⁄6 = -1⁄6
Multiplying Unlike Fractions
Multiplying unlike fractions is easier than adding or subtracting them. We can simply multiply the numerators and multiply the denominators, without finding a common denominator.
For example, to multiply 1⁄2 and 1⁄3, we can multiply the numerators (1 and 1) and multiply the denominators (2 and 3):
(1 × 1) / (2 × 3) = 1⁄6
Dividing Unlike Fractions
Dividing unlike fractions is similar to multiplying them. We can invert the second fraction (i.e., flip the numerator and denominator) and then multiply the two fractions.
For example, to divide 1⁄2 by 1⁄3, we can invert the second fraction (1⁄3 becomes 3⁄1) and then multiply the two fractions:
(1 × 3) / (2 × 1) = 3⁄2
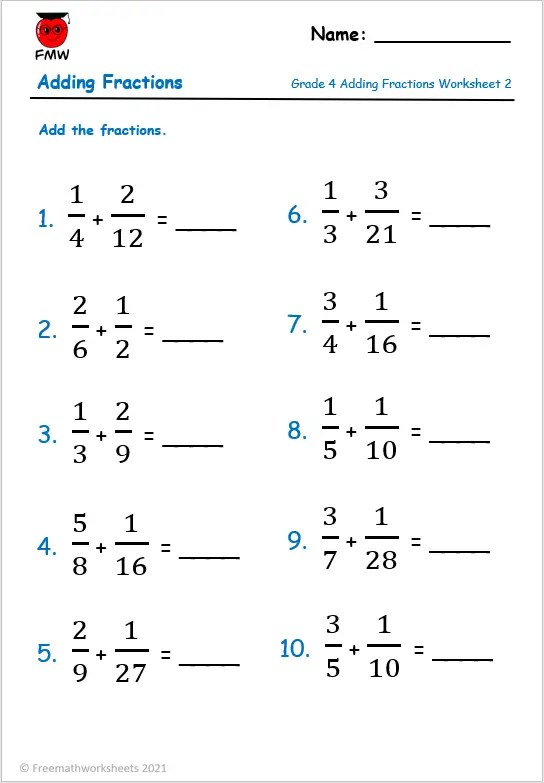
Worksheets for Unlike Fractions
Worksheets can be a great way to practice working with unlike fractions. Here are a few examples of worksheets you can use:
Adding Unlike Fractions Worksheet

| Problem | Solution |
|---|---|
| 1⁄2 + 1⁄3 | 5⁄6 |
| 1⁄4 + 1⁄6 | 5⁄12 |
| 2⁄3 + 1⁄4 | 11⁄12 |
Subtracting Unlike Fractions Worksheet
| Problem | Solution |
|---|---|
| 1⁄2 - 1⁄3 | -1⁄6 |
| 1⁄4 - 1⁄6 | -1⁄12 |
| 2⁄3 - 1⁄4 | 5⁄12 |
Multiplying Unlike Fractions Worksheet
| Problem | Solution |
|---|---|
| 1⁄2 × 1⁄3 | 1⁄6 |
| 1⁄4 × 1⁄6 | 1⁄24 |
| 2⁄3 × 1⁄4 | 1⁄6 |
Dividing Unlike Fractions Worksheet
| Problem | Solution |
|---|---|
| 1⁄2 ÷ 1⁄3 | 3⁄2 |
| 1⁄4 ÷ 1⁄6 | 3⁄2 |
| 2⁄3 ÷ 1⁄4 | 8⁄3 |
By practicing with these worksheets, you can master working with unlike fractions and become more confident in your math skills.
In conclusion, working with unlike fractions requires finding a common denominator, converting each fraction to have that denominator, and then adding, subtracting, multiplying, or dividing the fractions. With practice and the right worksheets, you can become proficient in working with unlike fractions.
What is the least common multiple (LCM) of two numbers?
+
The LCM is the smallest number that both numbers can divide into evenly.
How do I add unlike fractions?
+
To add unlike fractions, find a common denominator, convert each fraction to have that denominator, and then add the two fractions.
How do I multiply unlike fractions?
+
To multiply unlike fractions, multiply the numerators and multiply the denominators.
Related Terms:
- Adding unlike fractions Worksheet PDF