5 Ways to Simplify Unlike Fractions Easily

h2>Understanding Unlike Fractions and the Need for Simplification

Unlike fractions are fractions that have different denominators. Simplifying unlike fractions is an essential math skill that can help solve complex problems in various fields, including science, engineering, and finance. In this article, we will explore five ways to simplify unlike fractions easily.

h2>Merging the Least Common Multiple (LCM) Method

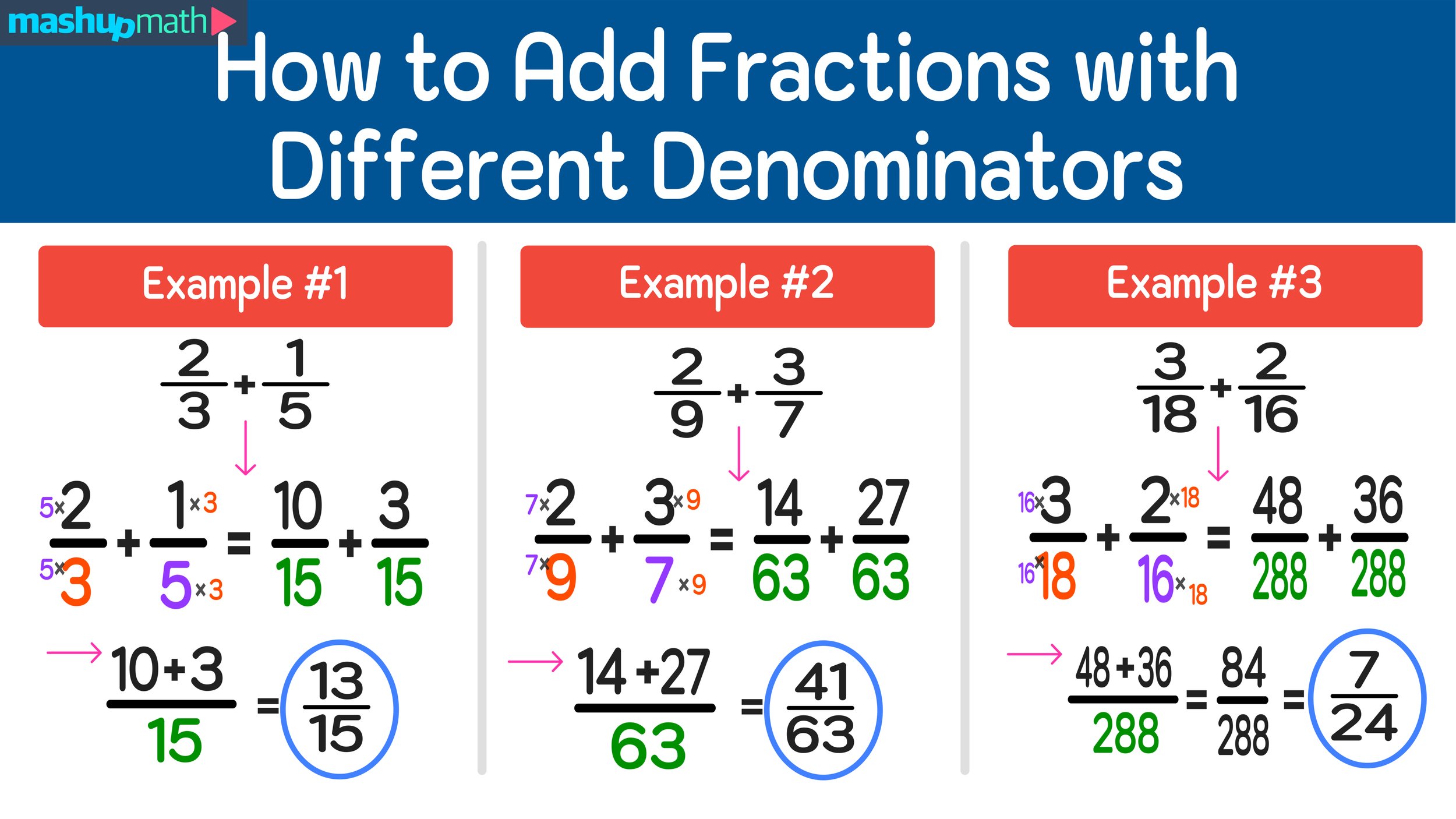
One of the most common methods to simplify unlike fractions is by finding the Least Common Multiple (LCM) of the denominators. The LCM is the smallest multiple that both denominators have in common.

For example, let’s simplify the following unlike fractions:

¹⁄₄ + ¹⁄₆

To find the LCM, list the multiples of each denominator:

Multiples of 4: 4, 8, 12, 16, 20,… Multiples of 6: 6, 12, 18, 24, 30,…

The first number that appears in both lists is 12, which is the LCM. Now, convert each fraction to have the LCM as the denominator:

(¹⁄₄) × (³⁄₃) = ³⁄₁₂ (¹⁄₆) × (²⁄₂) = ²⁄₁₂

Now, add the fractions:

³⁄₁₂ + ²⁄₁₂ = ⁵⁄₁₂

h2>The Visual Method: Drawing Diagrams

The visual method involves drawing diagrams to represent the fractions. This method can help visualize the problem and make it easier to simplify.

For example, let’s simplify the following unlike fractions:

¹⁄₃ + ¹⁄₄

Draw a diagram with 12 equal parts, divided into thirds and fourths:

Diagram

¹⁄₃ = 4 parts ¹⁄₄ = 3 parts

Now, count the total number of parts:

4 parts + 3 parts = 7 parts

The total number of parts is 12, so the simplified fraction is:

⁷⁄₁₂

h2>Using the Greatest Common Divisor (GCD) Method

The Greatest Common Divisor (GCD) method involves finding the greatest common divisor of the denominators and dividing both denominators by the GCD.

For example, let’s simplify the following unlike fractions:

²⁄₈ + ³⁄₁₂

Find the GCD of 8 and 12:

GCD(8, 12) = 4

Now, divide both denominators by the GCD:

(²⁄₈) ÷ (⁴⁄₄) = ¹⁄₂ (³⁄₁₂) ÷ (⁴⁄₄) = ³⁄₄

Now, find the LCM of the new denominators (2 and 4):

LCM(2, 4) = 4

Convert each fraction to have the LCM as the denominator:

(¹⁄₂) × (²⁄₂) = ²⁄₄ (³⁄₄) × (¹⁄₁) = ³⁄₄

Now, add the fractions:

²⁄₄ + ³⁄₄ = ⁵⁄₄

h2>The Equivalent Ratios Method

The equivalent ratios method involves finding equivalent ratios for each fraction and then comparing the ratios.

For example, let’s simplify the following unlike fractions:

¹⁄₂ + ¹⁄₃

Find equivalent ratios for each fraction:

¹⁄₂ = ²⁄₄ = ³⁄₆ ¹⁄₃ = ²⁄₆ = ³⁄₉

Now, compare the ratios:

²⁄₄ = ³⁄₆ ²⁄₆ = ³⁄₉

Now, find the LCM of the new denominators (4 and 6):

LCM(4, 6) = 12

Convert each fraction to have the LCM as the denominator:

(²⁄₄) × (³⁄₃) = ⁶⁄₁₂ (³⁄₆) × (²⁄₂) = ⁶⁄₁₂

Now, add the fractions:

⁶⁄₁₂ + ⁶⁄₁₂ = ¹²⁄₁₂

h2>The Number Line Method

The number line method involves using a number line to visualize the fractions and find the simplest form.

For example, let’s simplify the following unlike fractions:

¹⁄₄ + ¹⁄₆

Draw a number line with 12 equal parts:

Number Line

¹⁄₄ = 3 parts ¹⁄₆ = 2 parts

Now, count the total number of parts:

3 parts + 2 parts = 5 parts

The total number of parts is 12, so the simplified fraction is:

⁵⁄₁₂

p class=“pro-note”>💡 Note: The number line method can be used for more complex fractions, but it may become impractical for very large denominators.

In summary, simplifying unlike fractions can be done using various methods, including the LCM method, visual method, GCD method, equivalent ratios method, and number line method. Each method has its own advantages and disadvantages, and the choice of method depends on the specific problem and personal preference.