5 Ways to Add Negative and Positive Numbers
Understanding Negative and Positive Numbers
When dealing with numbers, it’s essential to understand the concept of negative and positive numbers. Negative numbers are numbers that are less than zero, while positive numbers are numbers that are greater than zero. Adding these numbers can seem intimidating, but with the right approach, it can be a breeze. In this article, we will explore five ways to add negative and positive numbers.
Method 1: Visualizing with a Number Line
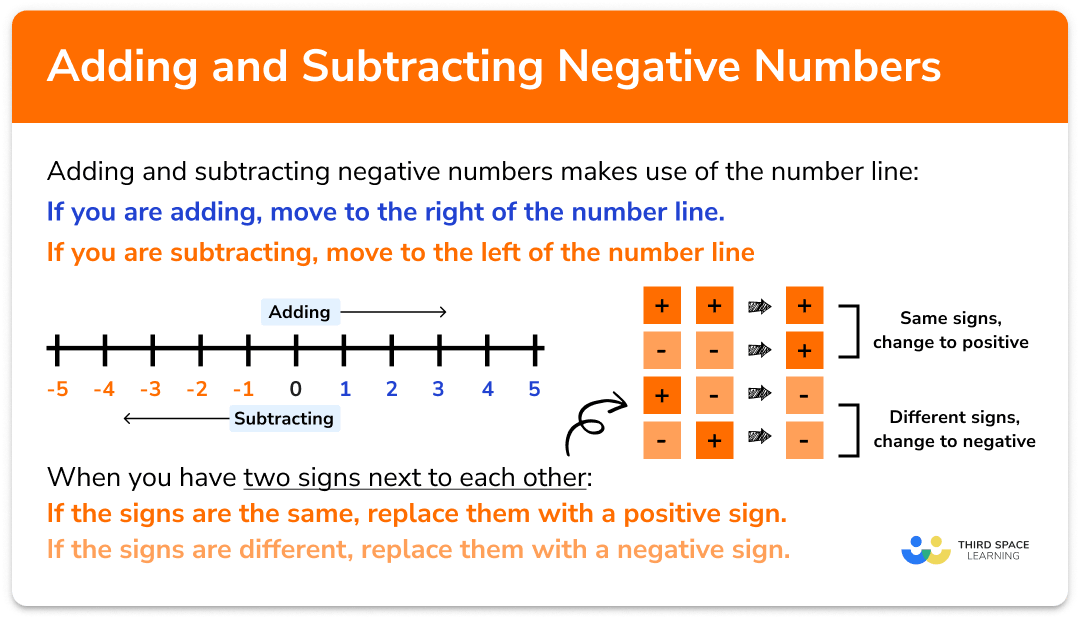
One of the most effective ways to add negative and positive numbers is by visualizing the process using a number line. A number line is a line that represents all the numbers, with zero in the middle. Positive numbers are represented on the right side of the number line, while negative numbers are represented on the left side.
To add a positive and a negative number using a number line, start by identifying the starting point, which is zero. Move to the right for positive numbers and to the left for negative numbers. For example, if you want to add 5 and -3, start at zero, move 5 units to the right, and then move 3 units to the left.
Example: 5 + (-3) =?
- Start at 0
- Move 5 units to the right (5)
- Move 3 units to the left (-3)
- End at 2
Answer: 2
📝 Note: When adding a positive and a negative number, you can think of it as "moving" along the number line. If you move to the right, you are adding a positive number, and if you move to the left, you are adding a negative number.
Method 2: Using the Rules of Signs
Another way to add negative and positive numbers is by using the rules of signs. The rules are as follows:
- Same signs: Add the numbers and keep the same sign
- Different signs: Subtract the numbers and take the sign of the larger number
Example: 2 + (-4) =?
- Different signs: Subtract the numbers (4 - 2 = 2)
- Take the sign of the larger number (-)
- Answer: -2
Method 3: Converting to Absolute Values
Converting numbers to their absolute values can make it easier to add negative and positive numbers. The absolute value of a number is its distance from zero, without considering its sign.
Example: -2 + 5 =?
- Convert to absolute values: |-2| = 2 and |5| = 5
- Add the absolute values: 2 + 5 = 7
- Determine the sign: Since -2 is negative, the result will be positive (5 is larger)
- Answer: 7
📝 Note: When converting numbers to their absolute values, remember that the absolute value of a negative number is always positive.
Method 4: Using Real-World Examples
Using real-world examples can help make adding negative and positive numbers more relatable. For instance, imagine you have 5 in your wallet and you owe someone 3.
Example: 5 + (-3) =?
- Think of the $5 as a positive number (you have it)
- Think of the -$3 as a negative number (you owe it)
- Add the numbers: 5 - 3 = $2
- Answer: $2
Method 5: Using a Calculator
Lastly, you can use a calculator to add negative and positive numbers. Most calculators have a (+/-) button that allows you to enter negative numbers.
Example: -2 + 5 =?
- Enter the numbers: -2 + 5 =
- Press the equal button: =
- Answer: 3

| Method | Example | Answer |
|---|---|---|
| Number Line | 5 + (-3) =? | 2 |
| Rules of Signs | 2 + (-4) =? | -2 |
| Absolute Values | -2 + 5 =? | 7 |
| Real-World Examples | $5 + (-$3) =? | $2 |
| Calculator | -2 + 5 =? | 3 |
In conclusion, adding negative and positive numbers can be a straightforward process using the right approach. By visualizing with a number line, using the rules of signs, converting to absolute values, using real-world examples, or using a calculator, you can become more confident in your ability to add these types of numbers.
What is the difference between a positive and a negative number?
+A positive number is a number that is greater than zero, while a negative number is a number that is less than zero.
How do I add a positive and a negative number?
+You can add a positive and a negative number by using a number line, the rules of signs, converting to absolute values, using real-world examples, or using a calculator.
What is the absolute value of a number?
+The absolute value of a number is its distance from zero, without considering its sign.
Related Terms:
- Integers worksheet addition and subtraction
- Multiplication negative numbers worksheet