Add Mixed Numbers with Like Denominators Made Easy

Mastering Mixed Numbers with Like Denominators: A Comprehensive Guide

When dealing with fractions, it’s essential to understand how to add mixed numbers with like denominators. This mathematical operation can seem daunting at first, but with the right approach, you’ll be able to tackle it with ease. In this article, we’ll break down the steps to add mixed numbers with like denominators, provide examples, and offer valuable tips to help you become a pro in no time.

Understanding Mixed Numbers and Like Denominators

Before we dive into the addition process, let’s quickly review the concepts of mixed numbers and like denominators.

• Mixed Numbers: A mixed number is a combination of a whole number and a fraction. For example, 2 ¹⁄₃ is a mixed number, where 2 is the whole number and ¹⁄₃ is the fraction.
• Like Denominators: Like denominators refer to fractions that have the same denominator. For instance, ¹⁄₄ and ³⁄₄ have like denominators because they both have 4 as the denominator.

Step-by-Step Guide to Adding Mixed Numbers with Like Denominators

Now that we’ve covered the basics, let’s move on to the step-by-step guide on how to add mixed numbers with like denominators:

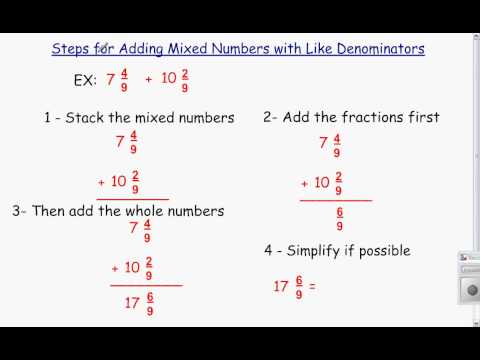
1. Identify the Whole Numbers: Identify the whole numbers in each mixed number. For example, if you’re adding 2 ¹⁄₄ and 1 ³⁄₄, the whole numbers are 2 and 1.
2. Add the Whole Numbers: Add the whole numbers together. In this case, 2 + 1 = 3.
3. Identify the Fractions: Identify the fractions in each mixed number. In this example, the fractions are ¹⁄₄ and ³⁄₄.
4. Add the Fractions: Add the fractions together. Since they have like denominators, you can directly add the numerators (1 + 3 = 4) and keep the same denominator (4). So, ¹⁄₄ + ³⁄₄ = ⁴⁄₄.
5. Simplify the Fraction: Simplify the fraction, if possible. In this case, ⁴⁄₄ can be simplified to 1.
6. Combine the Whole Number and Fraction: Combine the whole number and fraction to form the final mixed number. In this example, 3 + 1 = 4.

The final answer is 4.

Example Problems

Let’s practice with a few more examples:

• Example 1: Add 3 ¹⁄₆ and 2 ⁵⁄₆.

  • Identify the whole numbers: 3 and 2.
  • Add the whole numbers: 3 + 2 = 5.
  • Identify the fractions: ¹⁄₆ and ⁵⁄₆.
  • Add the fractions: ¹⁄₆ + ⁵⁄₆ = ⁶⁄₆.
  • Simplify the fraction: ⁶⁄₆ = 1.
  • Combine the whole number and fraction: 5 + 1 = 6.

  The final answer is 6.

• Example 2: Add 2 ³⁄₈ and 1 ⁵⁄₈.

  • Identify the whole numbers: 2 and 1.
  • Add the whole numbers: 2 + 1 = 3.
  • Identify the fractions: ³⁄₈ and ⁵⁄₈.
  • Add the fractions: ³⁄₈ + ⁵⁄₈ = ⁸⁄₈.
  • Simplify the fraction: ⁸⁄₈ = 1.
  • Combine the whole number and fraction: 3 + 1 = 4.

  The final answer is 4.

Tips and Tricks

• Make sure to line up the fractions: When adding fractions, make sure to line up the denominators to ensure accurate calculations.
• Simplify fractions: Always simplify fractions to their lowest terms to avoid confusion and ensure accuracy.
• Practice, practice, practice: The more you practice adding mixed numbers with like denominators, the more comfortable you’ll become with the process.

💡 Note: When adding mixed numbers with like denominators, it's essential to follow the order of operations and simplify fractions to ensure accurate calculations.

In conclusion, adding mixed numbers with like denominators is a straightforward process that requires attention to detail and a solid understanding of fractions. By following the step-by-step guide and practicing with example problems, you’ll become proficient in no time. Remember to simplify fractions and line up the denominators to ensure accurate calculations. Happy calculating!