5 Ways to Add Fractions With Same Denominator Easily

Understanding Fractions and Their Importance in Math

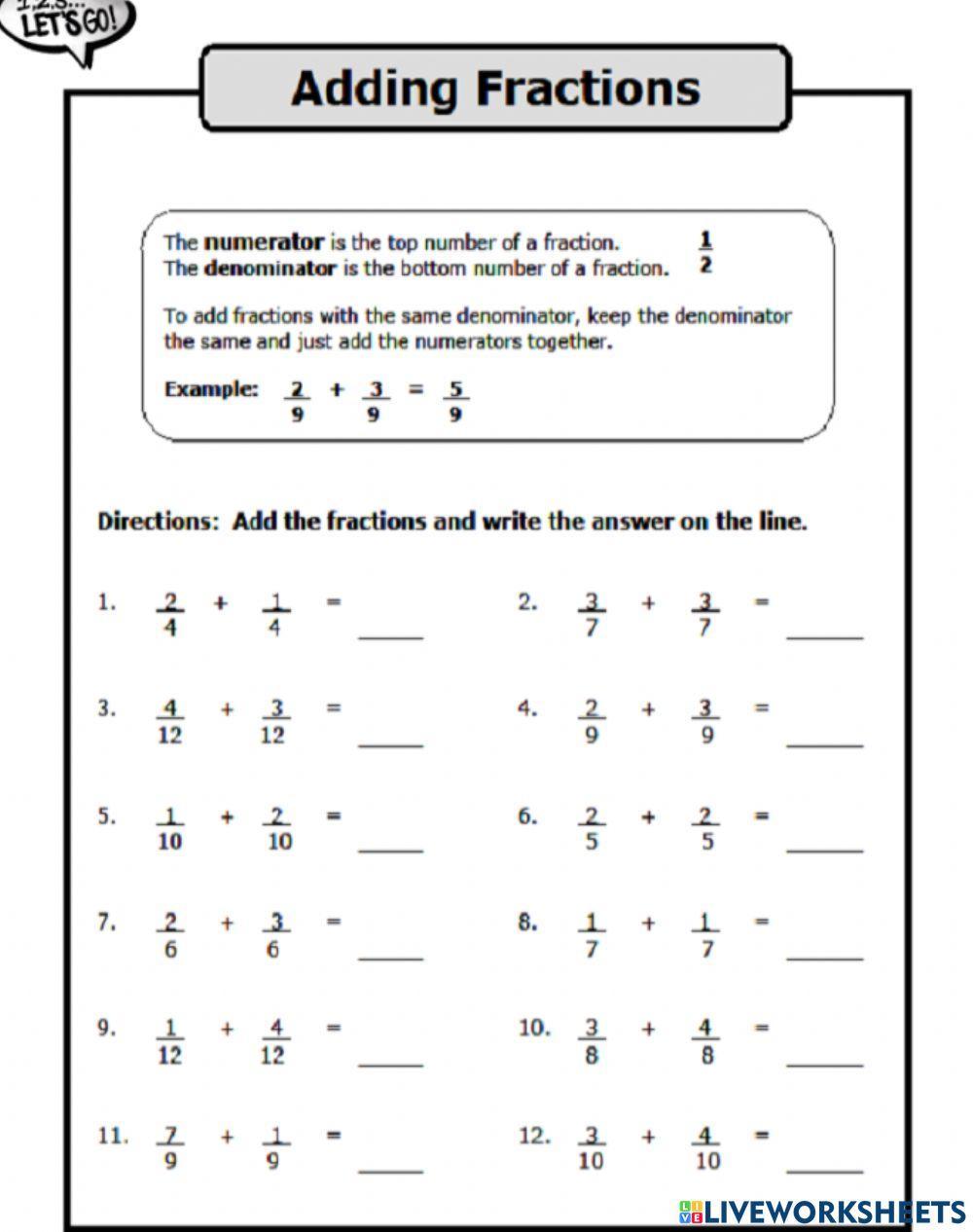
Fractions are a fundamental concept in mathematics, and learning how to add them is a crucial skill for students of all ages. A fraction is a way to represent a part of a whole, with the numerator (the top number) telling us how many equal parts we have, and the denominator (the bottom number) telling us how many parts the whole is divided into. In this article, we will focus on adding fractions with the same denominator, also known as like fractions.

Why is Adding Fractions with Same Denominator Important?

Adding fractions with the same denominator is an essential skill in mathematics because it allows us to combine different parts of a whole to find the total amount. This concept is used in various real-life situations, such as measuring ingredients for a recipe, calculating the area of a room, or determining the time it takes to complete a task.

Method 1: Adding Fractions with Same Denominator by Counting On

One way to add fractions with the same denominator is by counting on. This method involves starting with the first fraction and counting on to find the next fraction.

🤔 Note: This method is useful when the numerators are small.

For example, let’s add ¹⁄₆ and ²⁄₆:

• Start with ¹⁄₆
• Count on ²⁄₆: ¹⁄₆, ²⁄₆, ³⁄₆
• The answer is ³⁄₆

Method 2: Adding Fractions with Same Denominator by Using Number Lines

Another way to add fractions with the same denominator is by using number lines. This method involves creating a number line with the denominators marked and moving the fractions along the line to find the sum.

🤔 Note: This method is useful when the numerators are larger.

For example, let’s add ³⁄₈ and ²⁄₈:

• Create a number line with the denominators marked: 0, ¹⁄₈, ²⁄₈, ³⁄₈, ⁴⁄₈, ⁵⁄₈
• Move ³⁄₈ along the line: 0, ¹⁄₈, ²⁄₈, ³⁄₈
• Move ²⁄₈ along the line: ³⁄₈, ⁴⁄₈, ⁵⁄₈
• The answer is ⁵⁄₈

Method 3: Adding Fractions with Same Denominator by Using Real-World Objects

A third way to add fractions with the same denominator is by using real-world objects. This method involves using everyday objects to represent the fractions and counting the total amount.

🤔 Note: This method is useful when the numerators are small.

For example, let’s add ¹⁄₄ and ¹⁄₄:

• Use 4 blocks to represent ¹⁄₄
• Use another 4 blocks to represent ¹⁄₄
• Count the total number of blocks: 8 blocks
• The answer is ²⁄₄ or ¹⁄₂

Method 4: Adding Fractions with Same Denominator by Using Fraction Strips

A fourth way to add fractions with the same denominator is by using fraction strips. This method involves creating strips to represent the fractions and counting the total amount.

🤔 Note: This method is useful when the numerators are larger.

For example, let’s add ³⁄₆ and ²⁄₆:

• Create a strip to represent ³⁄₆
• Create a strip to represent ²⁄₆
• Count the total number of strips: ⁵⁄₆
• The answer is ⁵⁄₆

Method 5: Adding Fractions with Same Denominator by Using the Standard Algorithm

A fifth way to add fractions with the same denominator is by using the standard algorithm. This method involves adding the numerators and keeping the denominator the same.

🤔 Note: This method is useful when the numerators are large.

For example, let’s add ⁵⁄₁₂ and ³⁄₁₂:

• Add the numerators: 5 + 3 = 8
• Keep the denominator the same: 12
• The answer is ⁸⁄₁₂

In conclusion, adding fractions with the same denominator is an essential skill in mathematics, and there are several methods for doing so. By understanding the different methods and practicing with examples, students can become proficient in adding fractions with the same denominator and apply this skill to real-world situations.