Rational Numbers Addition and Subtraction Made Easy

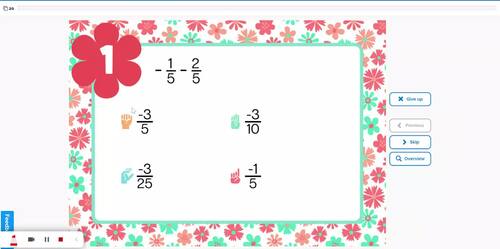
Understanding Rational Numbers

Rational numbers are an essential concept in mathematics, and understanding how to add and subtract them is crucial for solving various mathematical problems. Rational numbers are numbers that can be expressed as the ratio of two integers, where the denominator is non-zero. In other words, a rational number is a number that can be written in the form a/b, where a and b are integers and b is not equal to zero.

Adding Rational Numbers

Adding rational numbers is a straightforward process, but it requires some basic understanding of equivalent ratios and common denominators. To add two rational numbers, we need to follow these steps:

• Check if the denominators are the same. If they are, we can add the numerators directly.
• If the denominators are different, we need to find the least common multiple (LCM) of the two denominators.
• Once we have the LCM, we can rewrite each rational number with the LCM as the denominator.
• Finally, we can add the numerators and simplify the result.

🤔 Note: When adding rational numbers, it's essential to ensure that the denominators are the same. If they're not, we need to find the least common multiple (LCM) of the two denominators.

Example of Adding Rational Numbers

Suppose we want to add the rational numbers ¹⁄₄ and ¹⁄₆. To do this, we need to follow the steps outlined above.

• Check if the denominators are the same: In this case, the denominators are different (4 and 6).
• Find the least common multiple (LCM) of 4 and 6: The LCM of 4 and 6 is 12.
• Rewrite each rational number with the LCM as the denominator: ¹⁄₄ = ³⁄₁₂ and ¹⁄₆ = ²⁄₁₂.
• Add the numerators: 3 + 2 = 5.
• Simplify the result: ⁵⁄₁₂.

Subtracting Rational Numbers

Subtracting rational numbers is similar to adding them, but with a few key differences. To subtract two rational numbers, we need to follow these steps:

• Check if the denominators are the same. If they are, we can subtract the numerators directly.
• If the denominators are different, we need to find the least common multiple (LCM) of the two denominators.
• Once we have the LCM, we can rewrite each rational number with the LCM as the denominator.
• Finally, we can subtract the numerators and simplify the result.

🤔 Note: When subtracting rational numbers, it's essential to ensure that the denominators are the same. If they're not, we need to find the least common multiple (LCM) of the two denominators.

Example of Subtracting Rational Numbers

Suppose we want to subtract the rational numbers ¹⁄₄ and ¹⁄₆. To do this, we need to follow the steps outlined above.

• Check if the denominators are the same: In this case, the denominators are different (4 and 6).
• Find the least common multiple (LCM) of 4 and 6: The LCM of 4 and 6 is 12.
• Rewrite each rational number with the LCM as the denominator: ¹⁄₄ = ³⁄₁₂ and ¹⁄₆ = ²⁄₁₂.
• Subtract the numerators: 3 - 2 = 1.
• Simplify the result: ¹⁄₁₂.

Conclusion

In conclusion, adding and subtracting rational numbers is a straightforward process that requires some basic understanding of equivalent ratios and common denominators. By following the steps outlined above, we can easily add and subtract rational numbers, even when the denominators are different. By practicing these steps and becoming more comfortable with rational numbers, we can improve our math skills and become more confident in our ability to solve mathematical problems.