Rational Expressions Worksheet with Answers: Add and Subtract
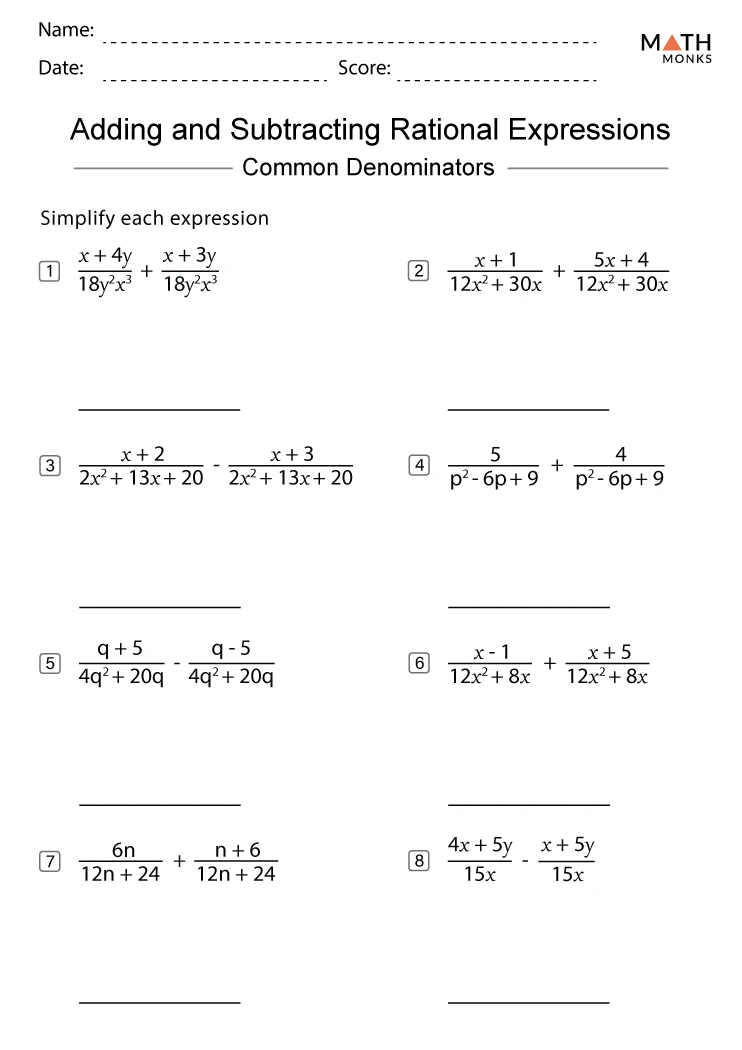
Rational Expressions Worksheet with Answers: Add and Subtract
Rational expressions are a fundamental concept in algebra, and adding and subtracting them can be a bit tricky. In this worksheet, we will provide you with a comprehensive guide on how to add and subtract rational expressions, along with examples and answers.
What are Rational Expressions?
A rational expression is a fraction that contains polynomials in both the numerator and denominator. For example, the expression 1/x is a rational expression, where x is a variable.
How to Add Rational Expressions
To add rational expressions, we need to follow these steps:
- Make sure the denominators of both expressions are the same.
- If the denominators are not the same, find the least common denominator (LCD).
- Rewrite each expression with the LCD as the denominator.
- Add the numerators and keep the denominator the same.
- Simplify the resulting expression.
📝 Note: When adding rational expressions, we can only add the numerators, not the denominators.
Examples of Adding Rational Expressions
Example 1: Add 1/x and 2/x
Since the denominators are the same (x), we can directly add the numerators:
1/x + 2/x = (1 + 2) / x = 3/x
Example 2: Add 1/x and 1/(x + 1)
The denominators are not the same, so we need to find the LCD, which is x(x + 1).
1/x = (x + 1) / x(x + 1)
1/(x + 1) = x / x(x + 1)
Now we can add:
(x + 1) / x(x + 1) + x / x(x + 1) = (2x + 1) / x(x + 1)
How to Subtract Rational Expressions
To subtract rational expressions, we need to follow these steps:
- Make sure the denominators of both expressions are the same.
- If the denominators are not the same, find the least common denominator (LCD).
- Rewrite each expression with the LCD as the denominator.
- Subtract the numerators and keep the denominator the same.
- Simplify the resulting expression.
📝 Note: When subtracting rational expressions, we can only subtract the numerators, not the denominators.
Examples of Subtracting Rational Expressions
Example 1: Subtract 2/x from 1/x
Since the denominators are the same (x), we can directly subtract the numerators:
1/x - 2/x = (1 - 2) / x = -1/x
Example 2: Subtract 1/(x + 1) from 1/x
The denominators are not the same, so we need to find the LCD, which is x(x + 1).
1/x = (x + 1) / x(x + 1)
1/(x + 1) = x / x(x + 1)
Now we can subtract:
(x + 1) / x(x + 1) - x / x(x + 1) = (1 - x) / x(x + 1)
Practice Time!
Here are some practice questions for you to try:
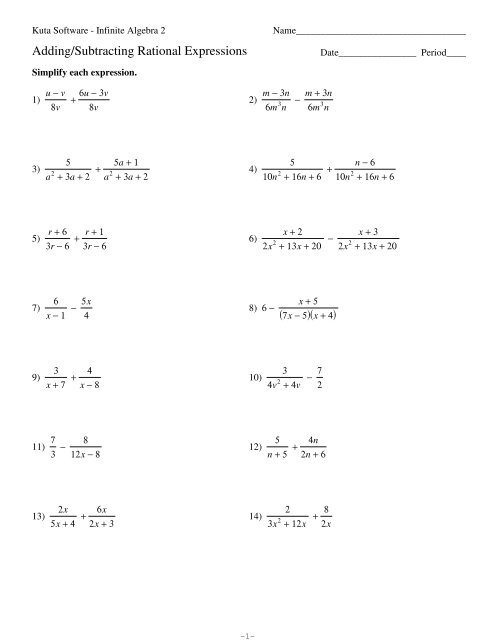
| Expression 1 | Expression 2 | Result |
|---|---|---|
| 1/x | 2/x | |
| 1/x | 1/(x + 1) | |
| 2/x | 3/x | |
| 1/(x + 1) | 1/(x - 1) |
Answers:
| Expression 1 | Expression 2 | Result |
|---|---|---|
| 1/x | 2/x | 3/x |
| 1/x | 1/(x + 1) | (2x + 1) / x(x + 1) |
| 2/x | 3/x | 5/x |
| 1/(x + 1) | 1/(x - 1) | (x - 2) / (x + 1)(x - 1) |
What is a rational expression?
+A rational expression is a fraction that contains polynomials in both the numerator and denominator.
How do I add rational expressions?
+To add rational expressions, make sure the denominators are the same, find the LCD if necessary, rewrite each expression with the LCD as the denominator, add the numerators, and simplify the resulting expression.
How do I subtract rational expressions?
+To subtract rational expressions, make sure the denominators are the same, find the LCD if necessary, rewrite each expression with the LCD as the denominator, subtract the numerators, and simplify the resulting expression.
In conclusion, adding and subtracting rational expressions can be done by following the steps outlined above. Remember to always check if the denominators are the same, find the LCD if necessary, and simplify the resulting expression. With practice, you will become more comfortable working with rational expressions.