Rational Expressions Made Easy: Addition and Subtraction Guide

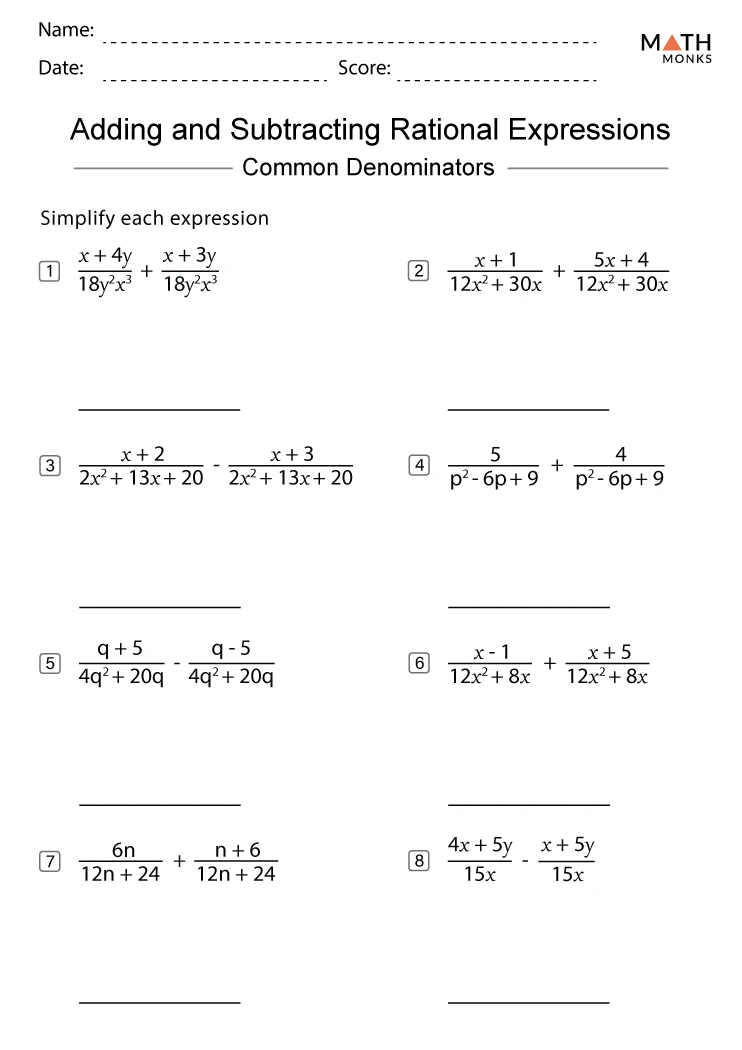
Rational Expressions Made Easy: Addition and Subtraction Guide

Rational expressions are a fundamental concept in algebra, and adding and subtracting them can be a bit tricky. However, with a few simple steps and some practice, you can master this skill. In this guide, we will walk you through the process of adding and subtracting rational expressions, and provide some tips and tricks to help you along the way.

What are Rational Expressions?

Before we dive into adding and subtracting rational expressions, let’s first define what they are. A rational expression is a fraction that contains polynomials in the numerator and denominator. For example:

1/(x+1), (x+2)/(x-1), and (x^2+1)/(x^2-4)

are all rational expressions.

Adding Rational Expressions

To add rational expressions, we need to follow these steps:

1. Find a common denominator: The first step is to find a common denominator for both rational expressions. This can be done by finding the least common multiple (LCM) of the denominators.
2. Write each rational expression with the common denominator: Once we have the common denominator, we can rewrite each rational expression with that denominator.
3. Add the numerators: Now that both rational expressions have the same denominator, we can add the numerators.
4. Simplify the result: Finally, we simplify the result by canceling out any common factors.

Let’s look at an example:

Add (x+1)/(x+2) and (x-1)/(x+2)

1. Find a common denominator: The least common multiple of (x+2) and (x+2) is (x+2).
2. Write each rational expression with the common denominator: (x+1)/(x+2) and (x-1)/(x+2)
3. Add the numerators: (x+1) + (x-1) = 2x
4. Simplify the result: 2x/(x+2)

So, the result of adding (x+1)/(x+2) and (x-1)/(x+2) is 2x/(x+2).

Subtracting Rational Expressions

To subtract rational expressions, we follow the same steps as adding, except that we subtract the numerators instead of adding them.

1. Find a common denominator: The first step is to find a common denominator for both rational expressions.
2. Write each rational expression with the common denominator: Once we have the common denominator, we can rewrite each rational expression with that denominator.
3. Subtract the numerators: Now that both rational expressions have the same denominator, we can subtract the numerators.
4. Simplify the result: Finally, we simplify the result by canceling out any common factors.

Let’s look at an example:

Subtract (x+1)/(x+2) from (x-1)/(x+2)

1. Find a common denominator: The least common multiple of (x+2) and (x+2) is (x+2).
2. Write each rational expression with the common denominator: (x-1)/(x+2) and (x+1)/(x+2)
3. Subtract the numerators: (x-1) - (x+1) = -2
4. Simplify the result: -2/(x+2)

So, the result of subtracting (x+1)/(x+2) from (x-1)/(x+2) is -2/(x+2).

Common Mistakes to Avoid

When adding and subtracting rational expressions, there are a few common mistakes to avoid:

• Not finding a common denominator: Make sure to find a common denominator for both rational expressions before adding or subtracting.
• Not simplifying the result: Don’t forget to simplify the result by canceling out any common factors.
• Adding or subtracting the wrong numerators: Double-check that you are adding or subtracting the correct numerators.

Conclusion

Adding and subtracting rational expressions can seem daunting at first, but with practice and patience, you can master this skill. Remember to always find a common denominator, write each rational expression with the common denominator, add or subtract the numerators, and simplify the result. With these steps and a few tips and tricks, you’ll be adding and subtracting rational expressions like a pro in no time!