Mastering Mixed Numbers: Add and Subtract with Ease

Mastering Mixed Numbers: Add and Subtract with Ease

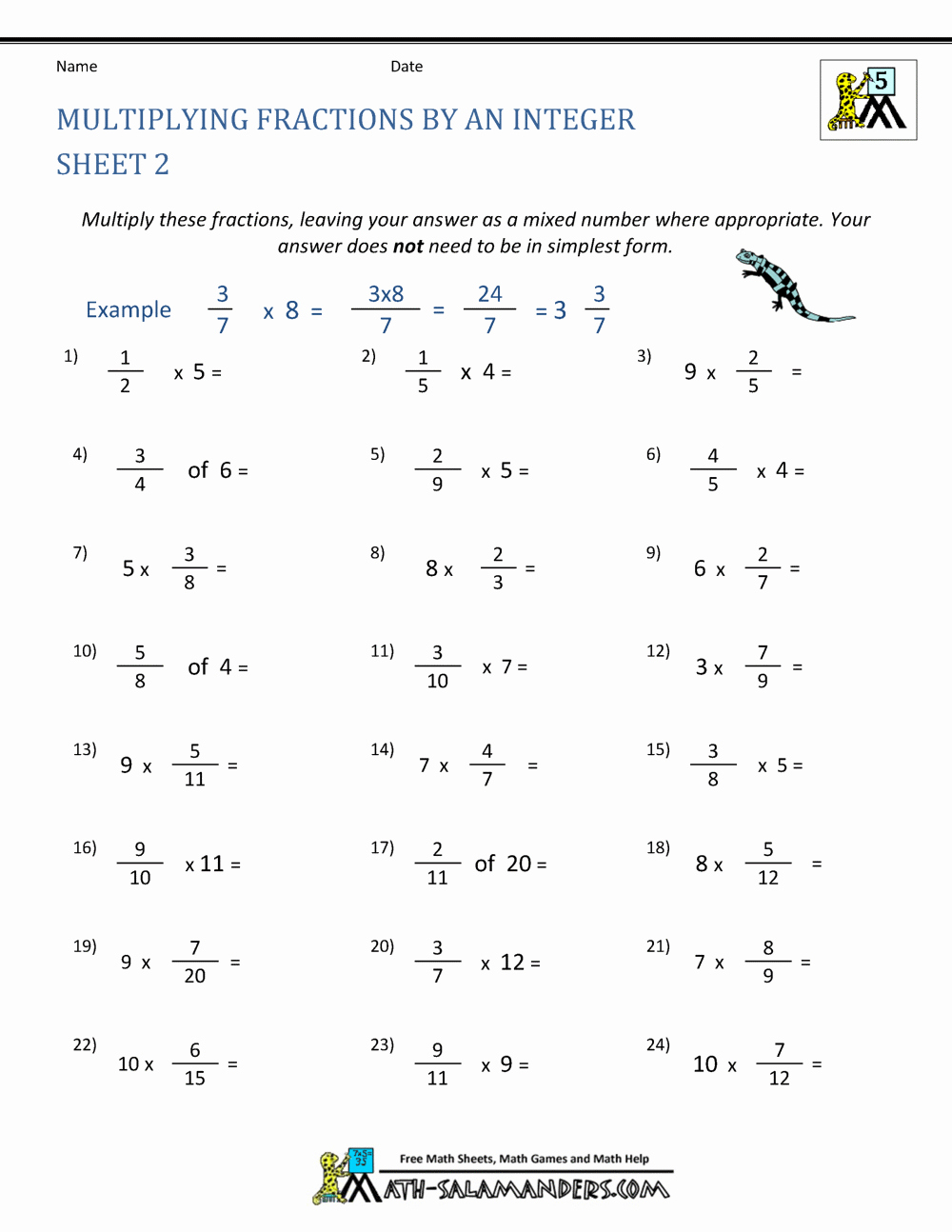
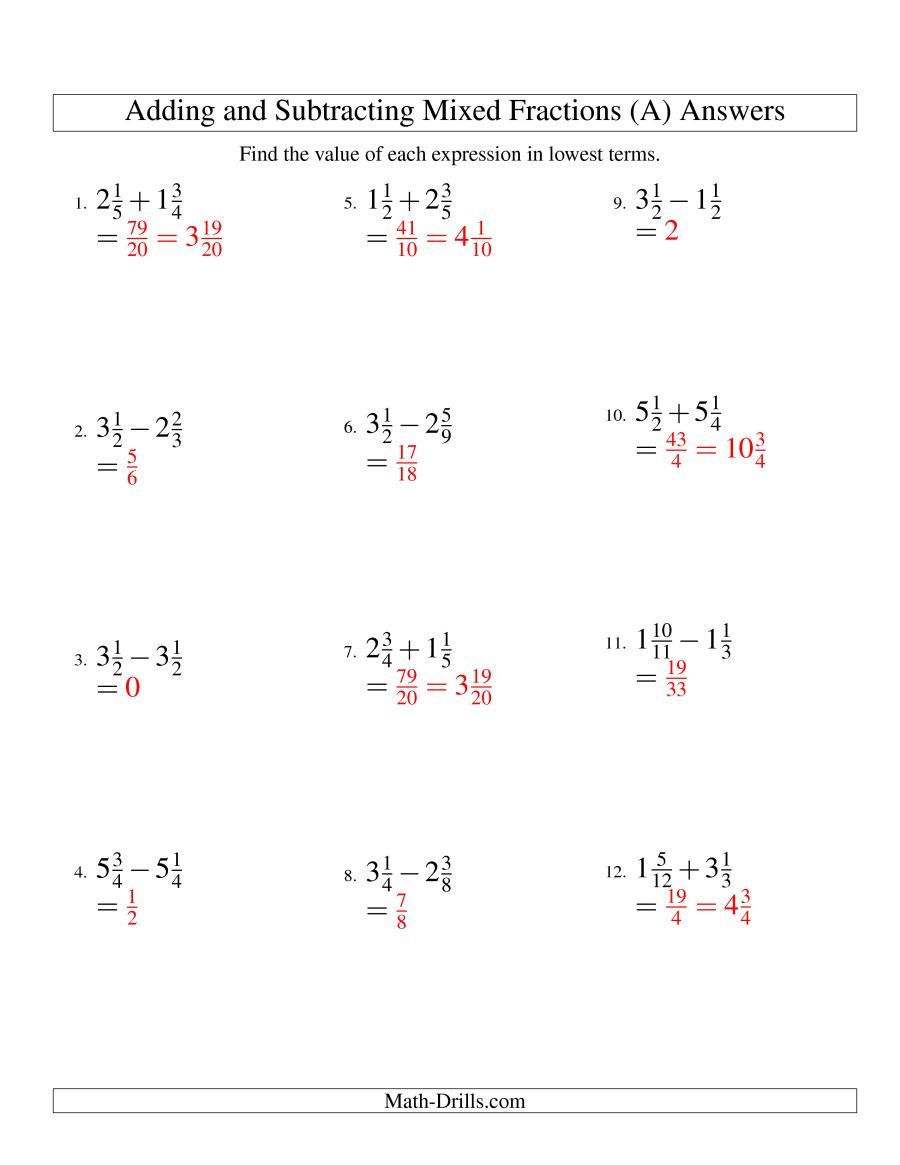
Mixed numbers are a combination of a whole number and a fraction. They are used to represent quantities that are not whole, but not entirely fractional either. For example, 3 ¹⁄₂ is a mixed number that represents three whole units and half a unit. Mastering mixed numbers is essential for performing arithmetic operations, such as addition and subtraction.

Adding Mixed Numbers

Adding mixed numbers involves adding the whole number parts and the fractional parts separately. Here’s a step-by-step guide:

1. Add the whole number parts: Add the whole number parts of the two mixed numbers.
2. Add the fractional parts: Add the fractional parts of the two mixed numbers. If the denominators are different, find the least common multiple (LCM) and convert both fractions to have the same denominator.
3. Combine the whole and fractional parts: Combine the whole number part and the fractional part to get the final answer.

Example: Add 2 ³⁄₄ and 1 ¹⁄₂

1. Add the whole number parts: 2 + 1 = 3
2. Add the fractional parts: ³⁄₄ + ¹⁄₂ = ³⁄₄ + ²⁄₄ = ⁵⁄₄
3. Combine the whole and fractional parts: 3 ⁵⁄₄

🤔 Note: When adding mixed numbers, make sure to simplify the fraction part if possible.

Subtracting Mixed Numbers

Subtracting mixed numbers involves subtracting the whole number parts and the fractional parts separately. Here’s a step-by-step guide:

1. Subtract the whole number parts: Subtract the whole number parts of the two mixed numbers.
2. Subtract the fractional parts: Subtract the fractional parts of the two mixed numbers. If the denominators are different, find the least common multiple (LCM) and convert both fractions to have the same denominator.
3. Combine the whole and fractional parts: Combine the whole number part and the fractional part to get the final answer.

Example: Subtract 3 ¹⁄₂ from 4 ³⁄₄

1. Subtract the whole number parts: 4 - 3 = 1
2. Subtract the fractional parts: ³⁄₄ - ¹⁄₂ = ³⁄₄ - ²⁄₄ = ¹⁄₄
3. Combine the whole and fractional parts: 1 ¹⁄₄

🤔 Note: When subtracting mixed numbers, make sure to simplify the fraction part if possible.

Real-World Applications of Mixed Numbers

Mixed numbers are used in various real-world applications, such as:

• Cooking: Recipes often involve mixed numbers, such as 2 ¹⁄₄ cups of flour.
• Building: Construction projects require precise measurements, including mixed numbers, such as 3 ³⁄₄ feet of lumber.
• Science: Scientific experiments often involve mixed numbers, such as 2 ¹⁄₂ liters of water.

Common Mistakes When Working with Mixed Numbers

Here are some common mistakes to avoid when working with mixed numbers:

• Forgetting to simplify fractions: Always simplify fractions to their lowest terms.
• Not finding the least common multiple (LCM): When adding or subtracting fractions, find the LCM to ensure the denominators are the same.
• Not combining whole and fractional parts: Make sure to combine the whole number part and the fractional part to get the final answer.

Conclusion

Mastering mixed numbers is essential for performing arithmetic operations, such as addition and subtraction. By following the step-by-step guides and avoiding common mistakes, you can become proficient in working with mixed numbers. Remember to simplify fractions, find the least common multiple (LCM), and combine whole and fractional parts to get the final answer.