Add and Subtract Mixed Fractions Made Easy
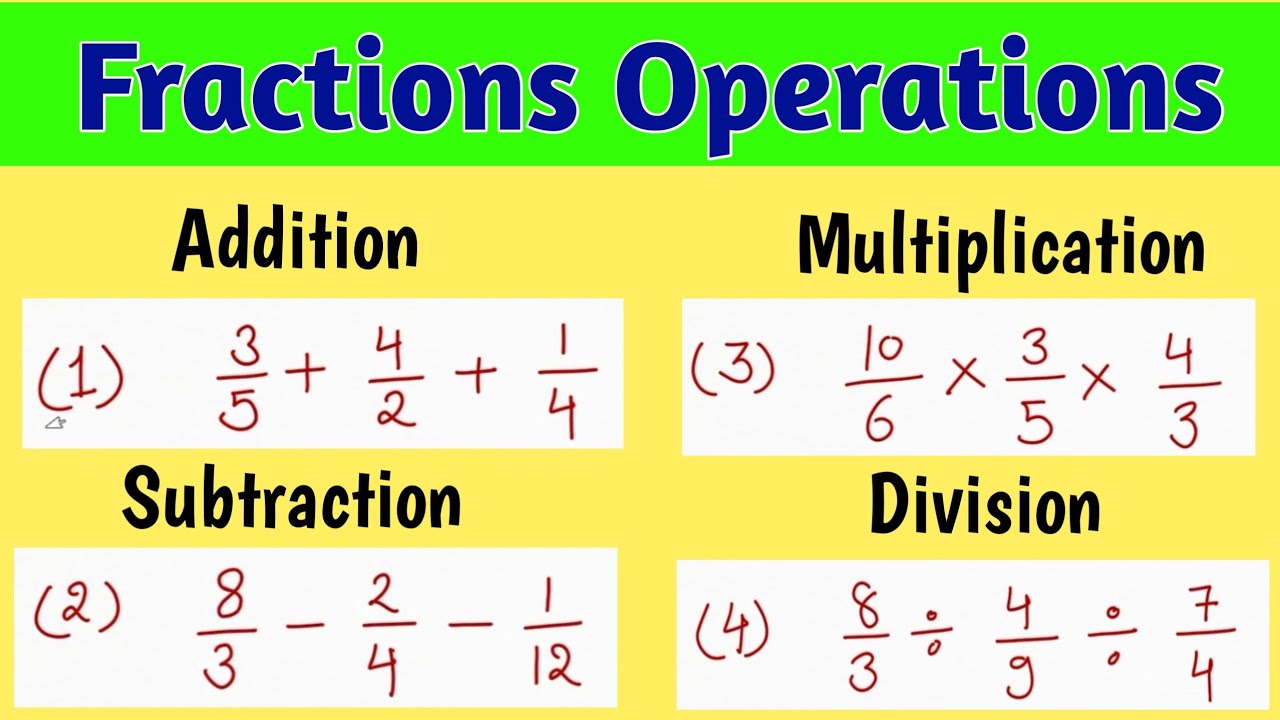
Understanding Mixed Fractions
Mixed fractions are a combination of a whole number and a proper fraction. They are used to represent quantities that are greater than one whole. For example, 2 3⁄4 is a mixed fraction that represents two whole units and three quarters of another unit. In this article, we will discuss how to add and subtract mixed fractions in a step-by-step manner.
Adding Mixed Fractions
Adding mixed fractions involves adding the whole number parts and the fractional parts separately. Here’s a step-by-step guide on how to add mixed fractions:
Step 1: Add the whole number parts Add the whole number parts of the two mixed fractions.
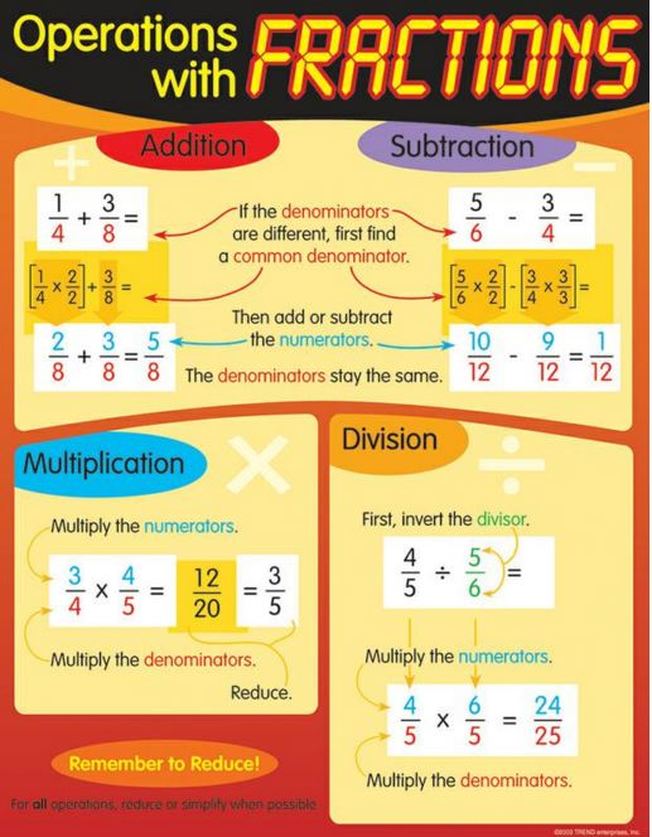
Step 2: Add the fractional parts Add the fractional parts of the two mixed fractions. If the denominators are the same, simply add the numerators. If the denominators are different, find the least common multiple (LCM) of the denominators and convert both fractions to have the same denominator.
Step 3: Combine the whole number and fractional parts Combine the whole number part from Step 1 and the fractional part from Step 2.
Step 4: Simplify the result Simplify the result by converting the improper fraction to a mixed fraction.
📝 Note: When adding mixed fractions, make sure to line up the whole number parts and the fractional parts separately.
Example 1:
Add 2 3⁄4 and 1 1⁄2
Step 1: Add the whole number parts 2 + 1 = 3
Step 2: Add the fractional parts 3⁄4 + 1⁄2 = 5⁄4 (convert 1⁄2 to 2⁄4 and then add)
Step 3: Combine the whole number and fractional parts 3 5⁄4
Step 4: Simplify the result 3 5⁄4 = 4 1⁄4 (convert 5⁄4 to 1 1⁄4 and then add to 3)
Therefore, the result of adding 2 3⁄4 and 1 1⁄2 is 4 1⁄4.
Subtracting Mixed Fractions
Subtracting mixed fractions involves subtracting the whole number parts and the fractional parts separately. Here’s a step-by-step guide on how to subtract mixed fractions:
Step 1: Subtract the whole number parts Subtract the whole number parts of the two mixed fractions.
Step 2: Subtract the fractional parts Subtract the fractional parts of the two mixed fractions. If the denominators are the same, simply subtract the numerators. If the denominators are different, find the least common multiple (LCM) of the denominators and convert both fractions to have the same denominator.
Step 3: Combine the whole number and fractional parts Combine the whole number part from Step 1 and the fractional part from Step 2.
Step 4: Simplify the result Simplify the result by converting the improper fraction to a mixed fraction.
📝 Note: When subtracting mixed fractions, make sure to line up the whole number parts and the fractional parts separately.
Example 2:
Subtract 3 1⁄2 from 5 3⁄4
Step 1: Subtract the whole number parts 5 - 3 = 2
Step 2: Subtract the fractional parts 3⁄4 - 1⁄2 = 1⁄4 (convert 1⁄2 to 2⁄4 and then subtract)
Step 3: Combine the whole number and fractional parts 2 1⁄4
Step 4: Simplify the result 2 1⁄4 (no simplification needed)
Therefore, the result of subtracting 3 1⁄2 from 5 3⁄4 is 2 1⁄4.
Table of Mixed Fraction Operations
| Operation | Example | Result |
|---|---|---|
| Addition | 2 3⁄4 + 1 1⁄2 | 4 1⁄4 |
| Subtraction | 5 3⁄4 - 3 1⁄2 | 2 1⁄4 |
To recap, adding and subtracting mixed fractions involves adding or subtracting the whole number parts and the fractional parts separately, and then combining the results. By following the step-by-step guides and examples provided in this article, you should be able to add and subtract mixed fractions with ease.
In conclusion, mixed fractions are an important part of mathematics, and being able to add and subtract them is a fundamental skill. With practice and patience, you can master the art of adding and subtracting mixed fractions.
What is a mixed fraction?
+A mixed fraction is a combination of a whole number and a proper fraction.
How do I add mixed fractions?
+To add mixed fractions, add the whole number parts and the fractional parts separately, and then combine the results.
How do I subtract mixed fractions?
+To subtract mixed fractions, subtract the whole number parts and the fractional parts separately, and then combine the results.