Solving Absolute Value Equations With Ease
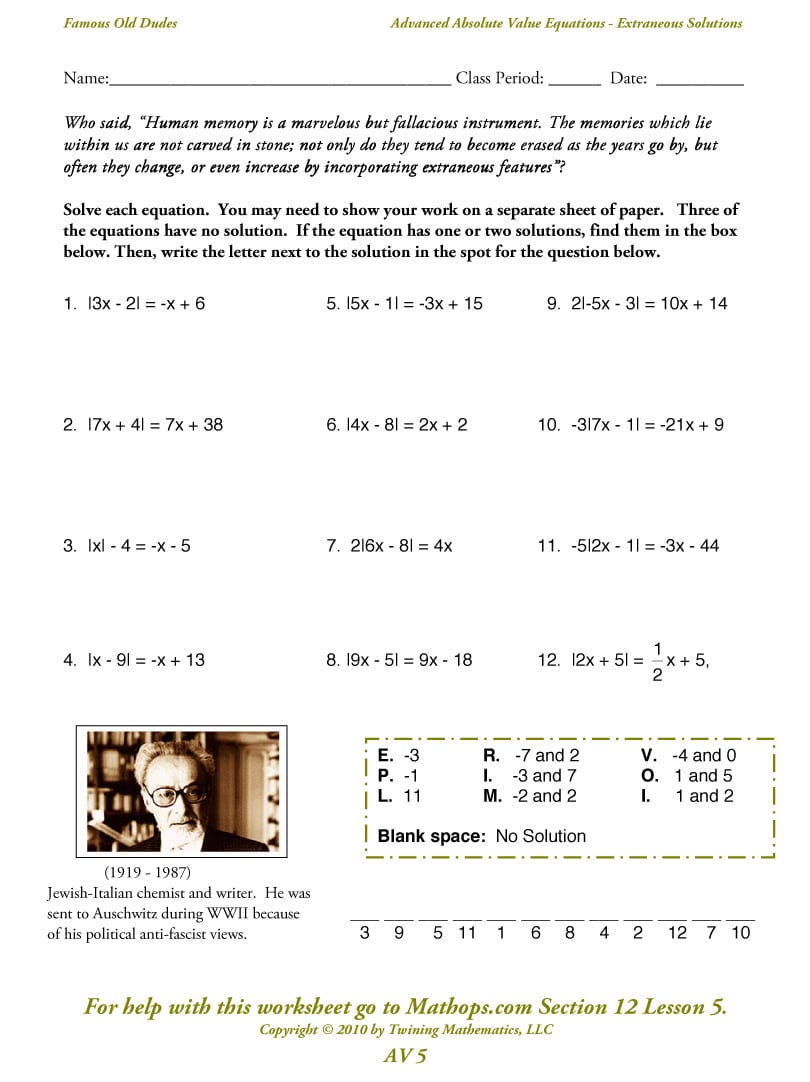
Introduction to Absolute Value Equations
Absolute value equations are a fundamental concept in algebra, and they can be a bit tricky to solve at first. However, with the right approach and understanding, you can master these types of equations with ease. In this blog post, we’ll delve into the world of absolute value equations, explore the different types of equations, and provide you with a step-by-step guide on how to solve them.
What are Absolute Value Equations?
Absolute value equations are equations that contain the absolute value of a variable or expression. The absolute value of a number is its distance from zero on the number line, without considering direction. It’s denoted by two vertical lines, | |, and it’s always non-negative. For example, |x| represents the absolute value of x.
Types of Absolute Value Equations
There are two main types of absolute value equations:
- Simple Absolute Value Equations: These are equations where the absolute value of a variable or expression is equal to a constant. For example, |x| = 5 or |2x + 3| = 7.
- Compound Absolute Value Equations: These are equations where the absolute value of a variable or expression is equal to another expression. For example, |x| = |2x + 3| or |x + 2| = |x - 3|.
Solving Simple Absolute Value Equations
Solving simple absolute value equations is relatively straightforward. Here are the steps to follow:
- Isolate the Absolute Value: Isolate the absolute value expression on one side of the equation.
- Write Two Equations: Write two separate equations, one with the positive value and one with the negative value.
- Solve Each Equation: Solve each equation separately to find the values of the variable.
Let’s illustrate this with an example:
Solve the equation |x| = 5.
- Isolate the absolute value: |x| = 5
- Write two equations: x = 5 or x = -5
- Solve each equation: x = 5 or x = -5
Therefore, the solutions to the equation are x = 5 and x = -5.
Solving Compound Absolute Value Equations
Solving compound absolute value equations is a bit more complex. Here are the steps to follow:
- Isolate the Absolute Value: Isolate the absolute value expression on one side of the equation.
- Write Two Equations: Write two separate equations, one with the positive value and one with the negative value.
- Solve Each Equation: Solve each equation separately to find the values of the variable.
- Check for Extraneous Solutions: Check for extraneous solutions by plugging the values back into the original equation.
Let’s illustrate this with an example:
Solve the equation |x| = |2x + 3|.
- Isolate the absolute value: |x| = |2x + 3|
- Write two equations: x = 2x + 3 or x = -(2x + 3)
- Solve each equation:
- x = 2x + 3 –> x = -3
- x = -(2x + 3) –> x = -1
- Check for extraneous solutions:
- Plug x = -3 back into the original equation: |-3| = |-6 + 3| –> 3 = 3 (True)
- Plug x = -1 back into the original equation: |-1| = |-2 + 3| –> 1 = 1 (True)
Therefore, the solutions to the equation are x = -3 and x = -1.
Common Mistakes to Avoid
When solving absolute value equations, it’s easy to make mistakes. Here are some common mistakes to avoid:
- Forgetting to Write Two Equations: When solving simple absolute value equations, make sure to write two separate equations, one with the positive value and one with the negative value.
- Forgetting to Check for Extraneous Solutions: When solving compound absolute value equations, make sure to check for extraneously solutions by plugging the values back into the original equation.
📝 Note: Always double-check your work to ensure that you haven't made any mistakes.
Conclusion
Solving absolute value equations can be a bit challenging, but with the right approach and understanding, you can master these types of equations with ease. Remember to isolate the absolute value, write two equations, solve each equation separately, and check for extraneous solutions. With practice and patience, you’ll become a pro at solving absolute value equations in no time!
What is the definition of an absolute value equation?
+An absolute value equation is an equation that contains the absolute value of a variable or expression.
What are the two main types of absolute value equations?
+The two main types of absolute value equations are simple absolute value equations and compound absolute value equations.
How do you solve a simple absolute value equation?
+To solve a simple absolute value equation, isolate the absolute value, write two equations, and solve each equation separately.
Related Terms:
- Absolute value Quadratic Equations worksheet