6 Ways to Master 6th Grade Inequalities

Mastering 6th Grade Inequalities: A Comprehensive Guide

Inequalities are a fundamental concept in mathematics, and mastering them is crucial for success in higher-level math courses. In 6th grade, students are introduced to basic inequality concepts, which can be challenging for some. In this article, we will explore six ways to master 6th grade inequalities, making it easier for students to grasp and apply these concepts.

Understanding the Basics of Inequalities

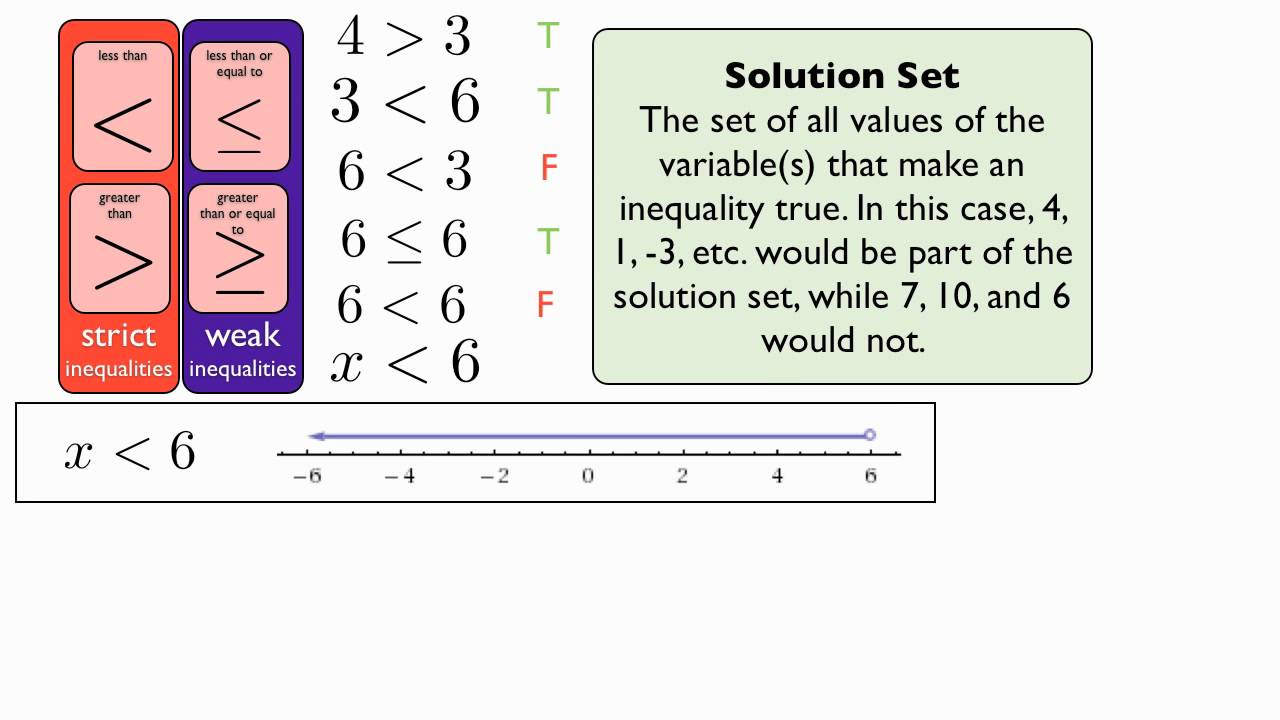
Before diving into the six ways to master 6th grade inequalities, it’s essential to understand the basics. An inequality is a statement that compares two expressions using greater than (>), less than (<), greater than or equal to (≥), or less than or equal to (≤) symbols. Inequalities can be solved using various methods, including addition, subtraction, multiplication, and division.

1. Visualizing Inequalities with Number Lines

Number lines are an excellent tool for visualizing inequalities. By plotting the inequality on a number line, students can easily identify the solution set. For example, the inequality x > 3 can be plotted on a number line, showing that all numbers greater than 3 are part of the solution set.

📝 Note: Using number lines helps students understand the concept of inequalities and how to represent them graphically.

2. Simplifying Inequalities with Algebraic Properties

Algebraic properties, such as the commutative and associative properties, can be used to simplify inequalities. For instance, the inequality 2x + 3 > 5 can be simplified by subtracting 3 from both sides, resulting in 2x > 2. This simplification helps students to more easily solve the inequality.

3. Solving Inequalities with Addition and Subtraction

Addition and subtraction are used to solve inequalities by isolating the variable on one side of the inequality. For example, the inequality x + 2 > 5 can be solved by subtracting 2 from both sides, resulting in x > 3.

4. Solving Inequalities with Multiplication and Division

Multiplication and division are also used to solve inequalities. When multiplying or dividing both sides of an inequality by a negative number, the direction of the inequality symbol must be reversed. For instance, the inequality -2x > 6 can be solved by dividing both sides by -2, resulting in x < -3.

5. Graphing Inequalities on Coordinate Planes

Graphing inequalities on coordinate planes is an excellent way to visualize the solution set. By plotting the inequality on a coordinate plane, students can see the relationship between the x and y variables. For example, the inequality y > 2x can be graphed on a coordinate plane, showing that all points above the line y = 2x are part of the solution set.

6. Applying Inequalities to Real-World Problems

Applying inequalities to real-world problems helps students to see the practical application of these concepts. For example, a store is having a sale on shirts, and the cost of a shirt is $15. If you have a 20% discount coupon, you can use an inequality to determine the maximum amount you can spend on a shirt.

📝 Note: Applying inequalities to real-world problems helps students to develop problem-solving skills and to see the relevance of inequalities in everyday life.

Conclusion

Mastering 6th grade inequalities requires practice, patience, and persistence. By using the six methods outlined in this article, students can develop a deeper understanding of inequalities and improve their math skills. Remember, inequalities are a fundamental concept in mathematics, and mastering them will help students to succeed in higher-level math courses.

Related Terms:

• 6th grade Inequalities Worksheet PDF
• Inequalities Worksheet PDF with answers
• Inequalities Grade 6
• Inequality worksheets